Basic Principles of Pharmacology

Volume of Distribution

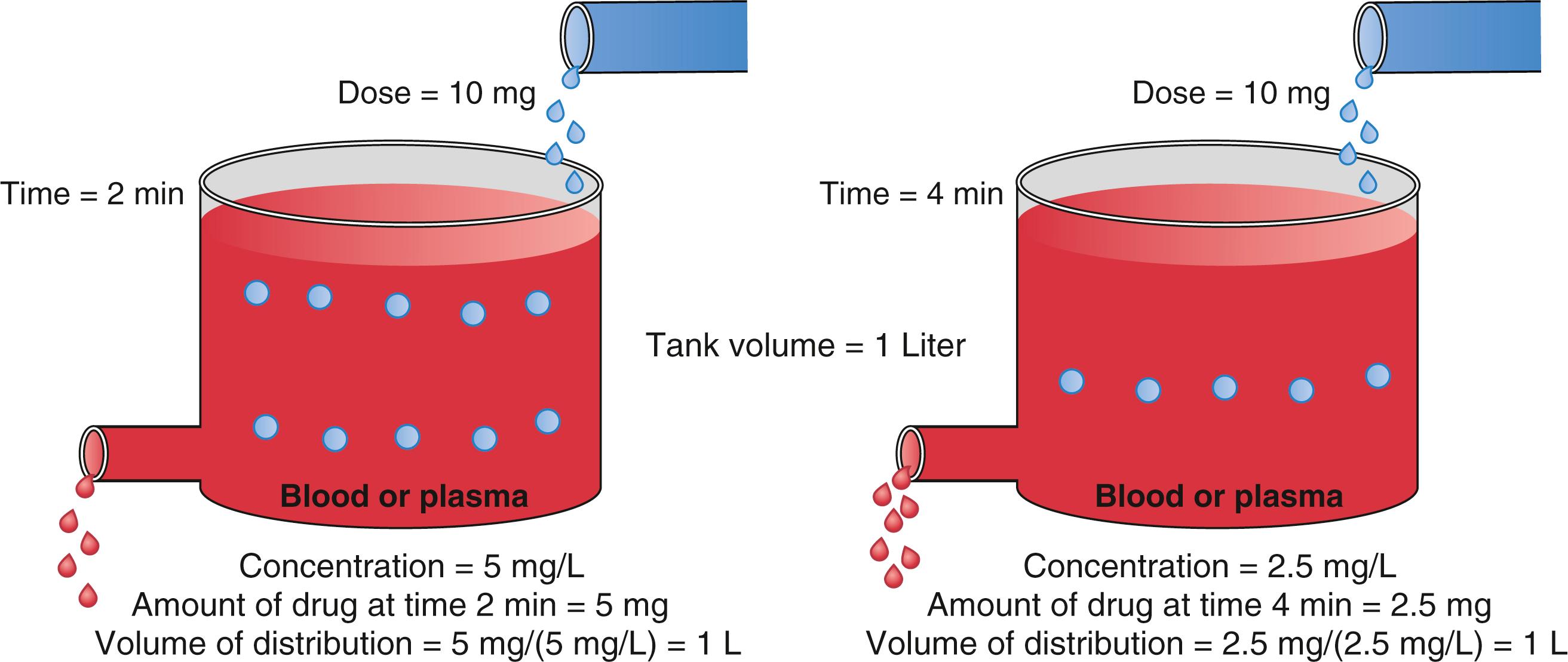
An over-simplified model of drug distribution throughout plasma and tissues is the dilution of a drug dose into a tank of water. The volume of distribution (Vd) is the apparent size of the tank in which a known amount of drug distributes to produce a measured drug concentration once the drug has had enough time to thoroughly mix within the tank ( Fig 18.1 ). If an injected drug disperses and distributes instantaneously throughout the tank without any drug degradation, the distribution volume is estimated using the simple relationship between dose (e.g., mg) and measured concentration (e.g., mg/L) as presented in Eq. (18.1) .

With an estimate of tank volume, drug concentration after any bolus dose can be calculated. Human bodies are not like water tanks. As soon as a drug is injected, it begins to be cleared from the body. To account for this in the schematic presented in Fig. 18.1 , a faucet is added to the tank to mimic drug elimination from the body ( Fig. 18.2 ). Considering the elimination of drug from the tank and the changes in concentration, the definition of distribution volume in Eq. (18.1) should be refined with the amount of drug and the concentration at a given time t.

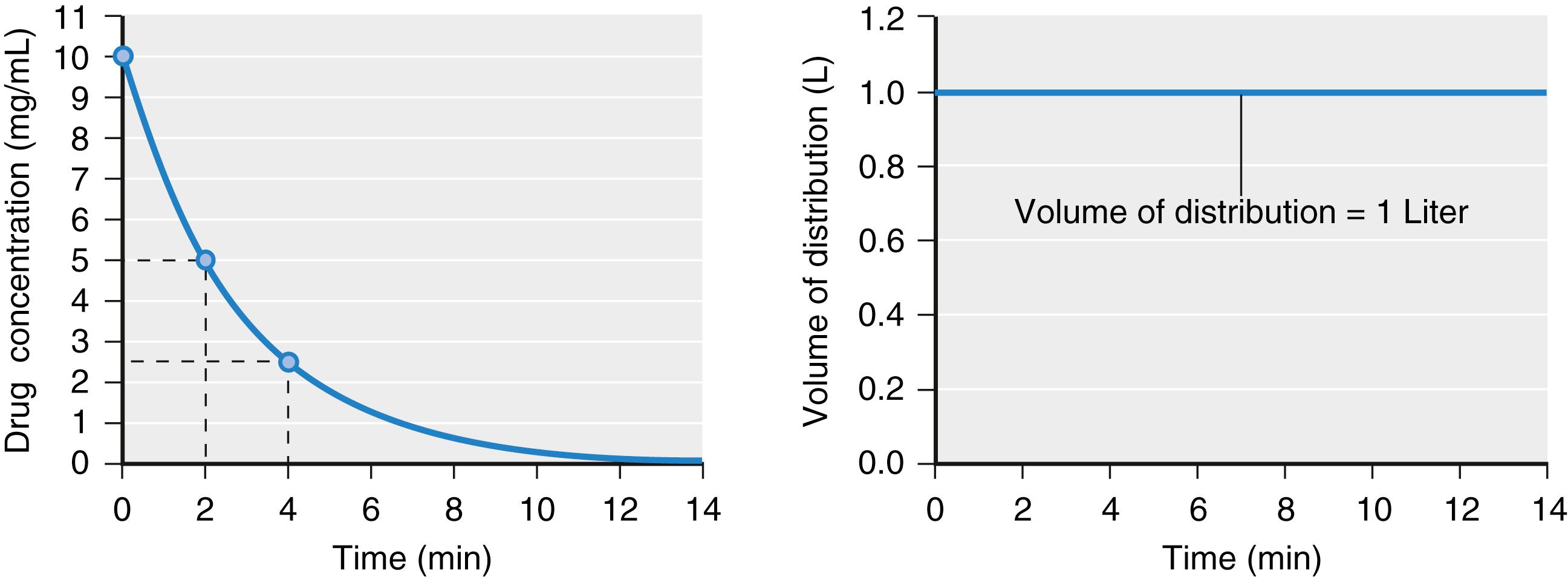
If drug elimination occurs as a first-order process (i.e., elimination is proportional to the concentration at that time), in a tank model, the volume of distribution calculated by Eq. (18.2) will be constant ( Fig. 18.3 ; see also Fig.18.2 ). When a drug is administered intravenously, some drug stays in the vascular volume, but most of the drug distributes to peripheral tissues. This distribution is often represented as additional tanks (peripheral distribution volumes) connected to a central tank (blood or plasma volume). Peripheral distribution volumes increase the total volume of distribution ( Fig. 18.4 ). For the calculation of distribution volumes, peripheral tissue concentrations are difficult to measure whereas plasma concentrations are easily measured.

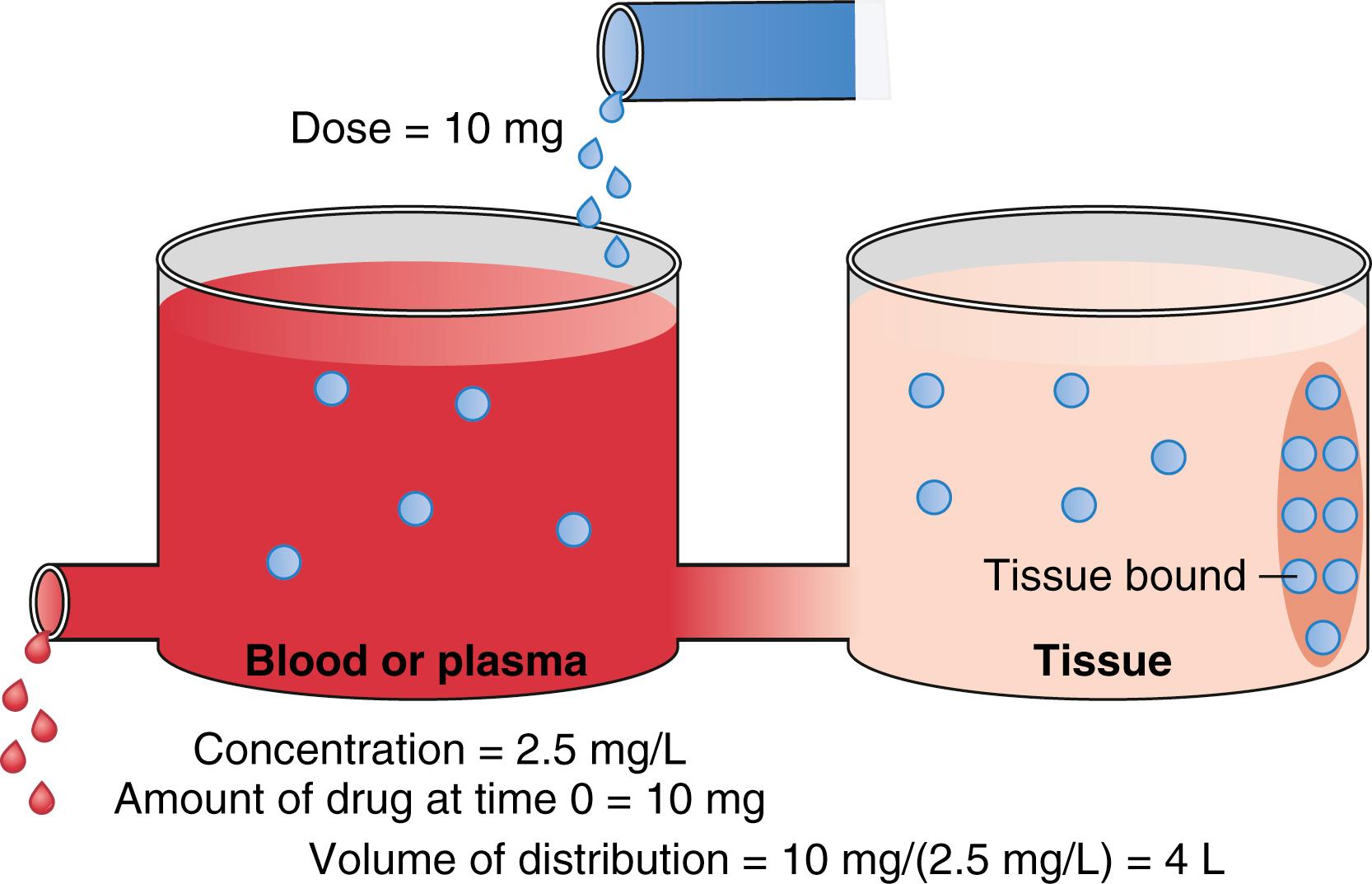
The schematic in Fig. 18.4 presents two tanks that represent plasma and peripheral tissue volumes. The peripheral tank represents the drug volume of distribution in peripheral tissues. There may be more than one peripheral tank (volume) to best describe the entire drug disposition in the body. The size of the peripheral volumes represents a drug’s solubility in tissue relative to blood or plasma. The more soluble a drug is in peripheral tissue relative to blood or plasma, the larger the peripheral volumes of distribution.

An important point illustrated in Fig. 18.4 is that drug not only distributes to the peripheral tank and thus increases the volume of distribution, but it also binds to tissue in that tank. This process further lowers the measurable concentration in the central tank. Thus, the total volume of distribution may even be larger than the two tanks added together. In fact, some anesthetics have huge distribution volumes (e.g., fentanyl has an apparent distribution volume of 4 L/kg) that are substantially larger than an individual’s vascular volume (0.07 L/kg) or extracellular volume (0.2 L/kg).

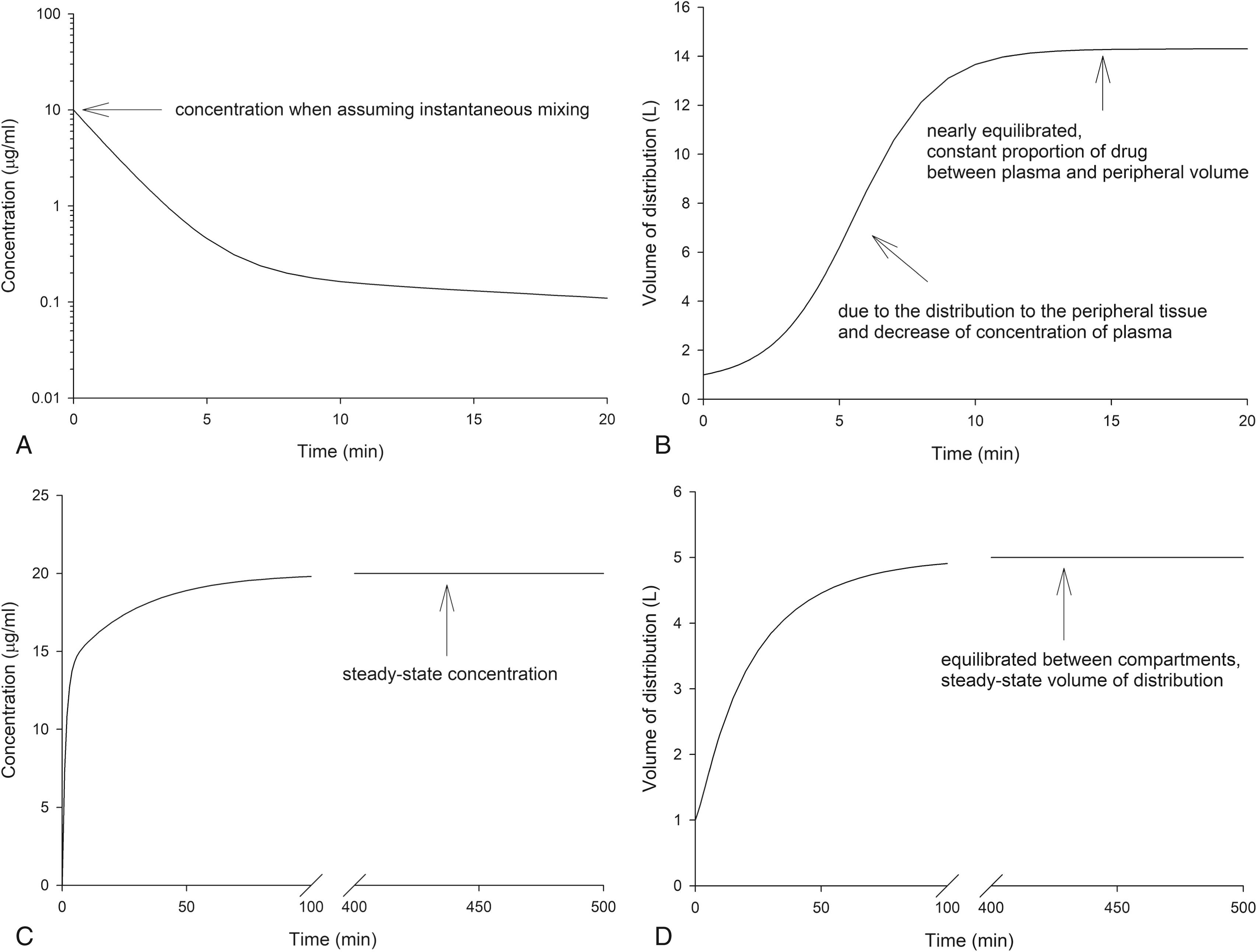
With additional distribution volumes, the overall volume of distribution can change over time and is a function of how drug is administered as well (e.g., as a bolus or a continuous infusion). For example, consider simulations of concentrations and distribution volumes over time following a bolus dose or a continuous infusion of an intravenous anesthetic as presented in Fig. 18.5 . For a bolus dose, assume that the volume of distribution is 1 L at time = 0 and that it then increases to 14 L as the plasma concentration falls over the next 10 minutes. The increase of the distribution volume is due to the distribution of drug to peripheral tissue and a decrease in the plasma concentration. For a constant infusion, assume the volume of distribution is again 1 L at time = 0 and that it then increases to 5 L as the plasma concentrations also increase to a steady-state concentration over the next several hours. This is known as the steady-state volume of distribution. It is estimated as the sum of the central and peripheral apparent distribution volumes.

Clearance

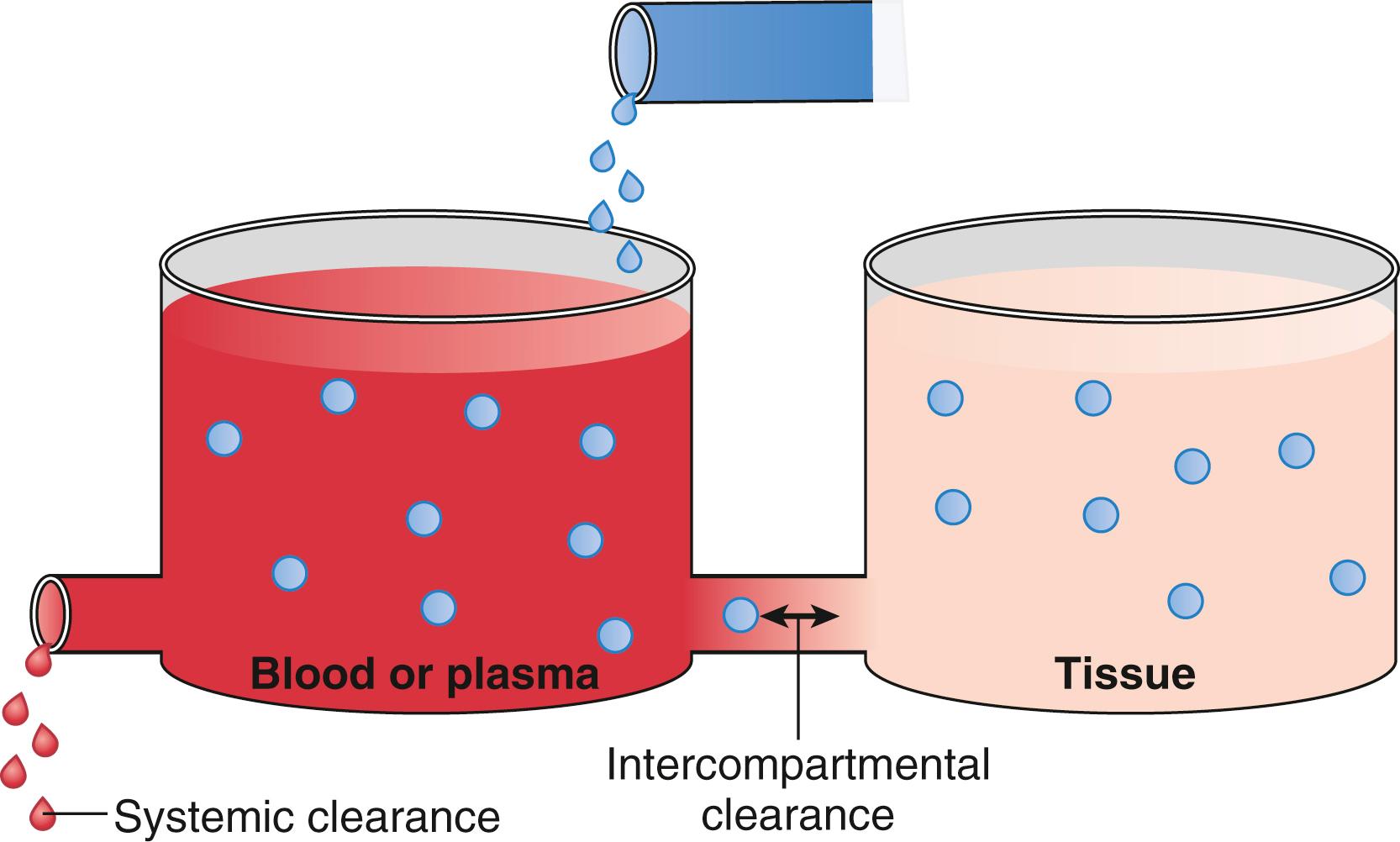
Clearance describes the rate of drug removal from the plasma/blood. Two processes contribute to drug clearance: systemic (removal from the tank) and intercompartmental (between tanks) clearance ( Fig. 18.6 ). Systemic clearance permanently removes drug from the body, either by eliminating the parent molecule or by transforming it into metabolites. Intercompartmental clearance moves drug between plasma and peripheral tissue tanks. By way of clarification, in this chapter, the words compartment and tank are interchangeable.

Clearance is defined in units of flow, that is, the volume completely cleared of drug per unit of time (e.g., L/min). Clearance is not to be confused with elimination rate (e.g., mg/min). The elimination rate is not an accurate method of describing the mass of drug removed over time. For example, assuming a first-order process, when plasma concentrations are high, the rate of drug elimination is high. When low, the rate is also low. Clearance is a better descriptor as it is independent of drug concentration.

To illustrate this point, consider the simulation presented in Fig. 18.7 . In this simulation, the total amount of drug at each time can be calculated from the known volume of distribution and measured concentration. The concentration change in time window A is larger than in time window B even though they are both 1 minute in duration. The elimination rates are 28.4 and 10.4 mg/min for time windows A and B, respectively. They are different, and neither can be used as a parameter to represent a measure of drug removal from the body. Because of this limitation with elimination rate, clearance was developed to provide a single number to describe the decay in drug concentration presented in Fig. 18.7 .

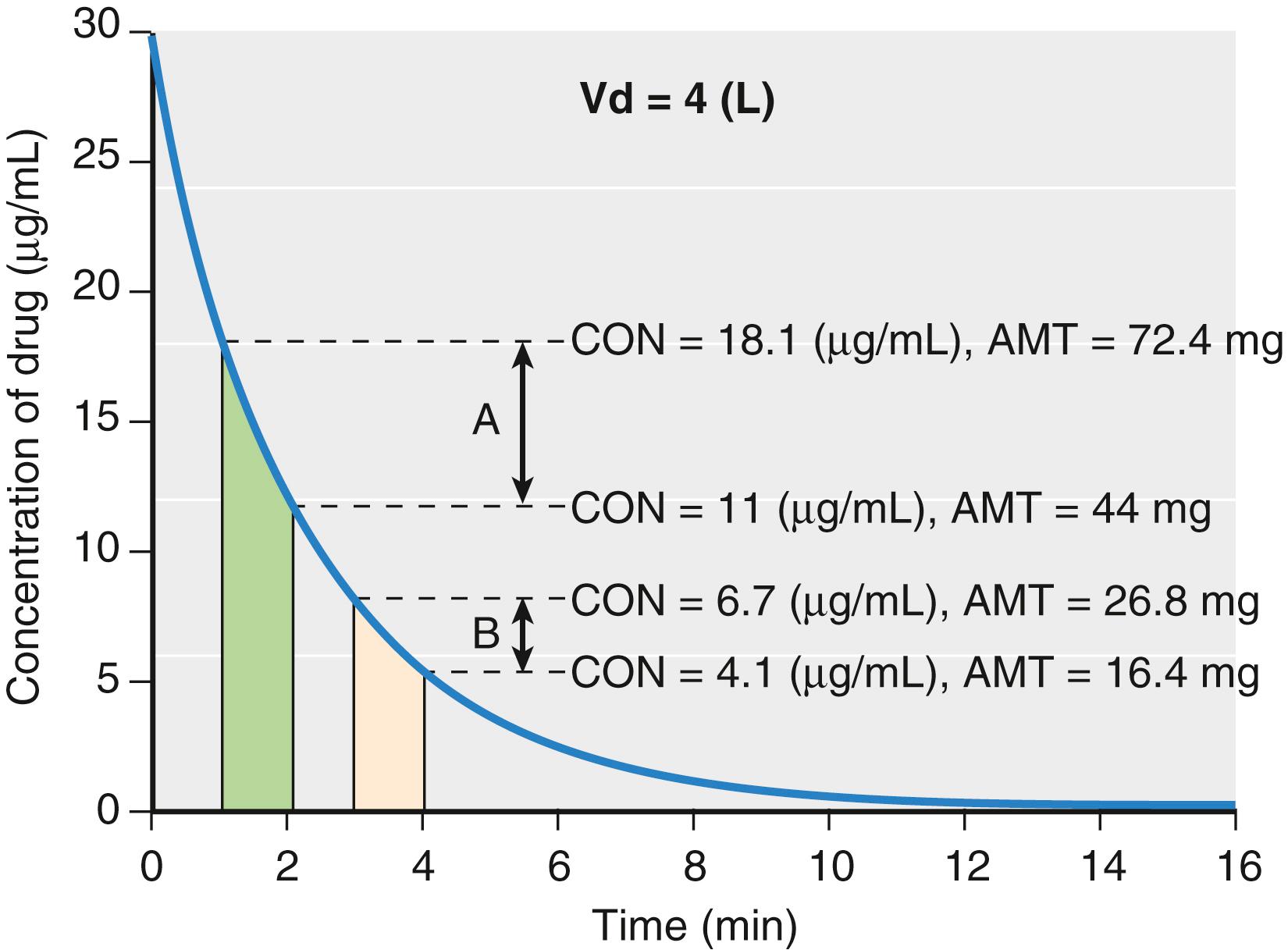
For discussion purposes, assume that concentration is the power necessary to push drug out of the water tank. The higher the concentration, the larger the amount of drug eliminated. To standardize the elimination rate, the eliminated amount of drug is scaled to concentration. For example, when the elimination rate in time window A (28.4 mg/min) is scaled to the concentration at the middle of the time window (14.2 μg/mL), the clearance is 2 L/min. When the elimination rate in time window B (10.4 mg/min) is scaled to the concentration at the middle of the time window (5.2 μg/mL), the clearance is again 2 L/min. If the time interval is narrowed so that the time window approaches zero, the definition of clearance becomes:

where dA(t)/dt is the rate of drug elimination at given time t, and C(t) is the corresponding concentration at that time. Rearranging Eq. (18.3) and integrating both numerator and denominator, the following relationship holds:

because the term ${\int }_0^{\infty }\text{dA}\left(\text{t}\right)$ is equal to the total amount of drug eliminated and ${\int }_0^{\infty }\text{C}\left(\text{t}\right)\text{dt}$ is the area under curve (AUC) in concentration versus time plot, then the following equation can be derived:

With long infusions, drug concentrations reach a steady-state condition where the rate of drug elimination $\left(\frac{\text{dA}\left(\text{t}\right)}{\text{dt}}\right)$ is in equilibrium with the rate of drug administration (infusion rate). Clearance in a steady-state condition can be obtained using Eq. (18.3) as follows:

where Css is the plasma concentration at steady state.

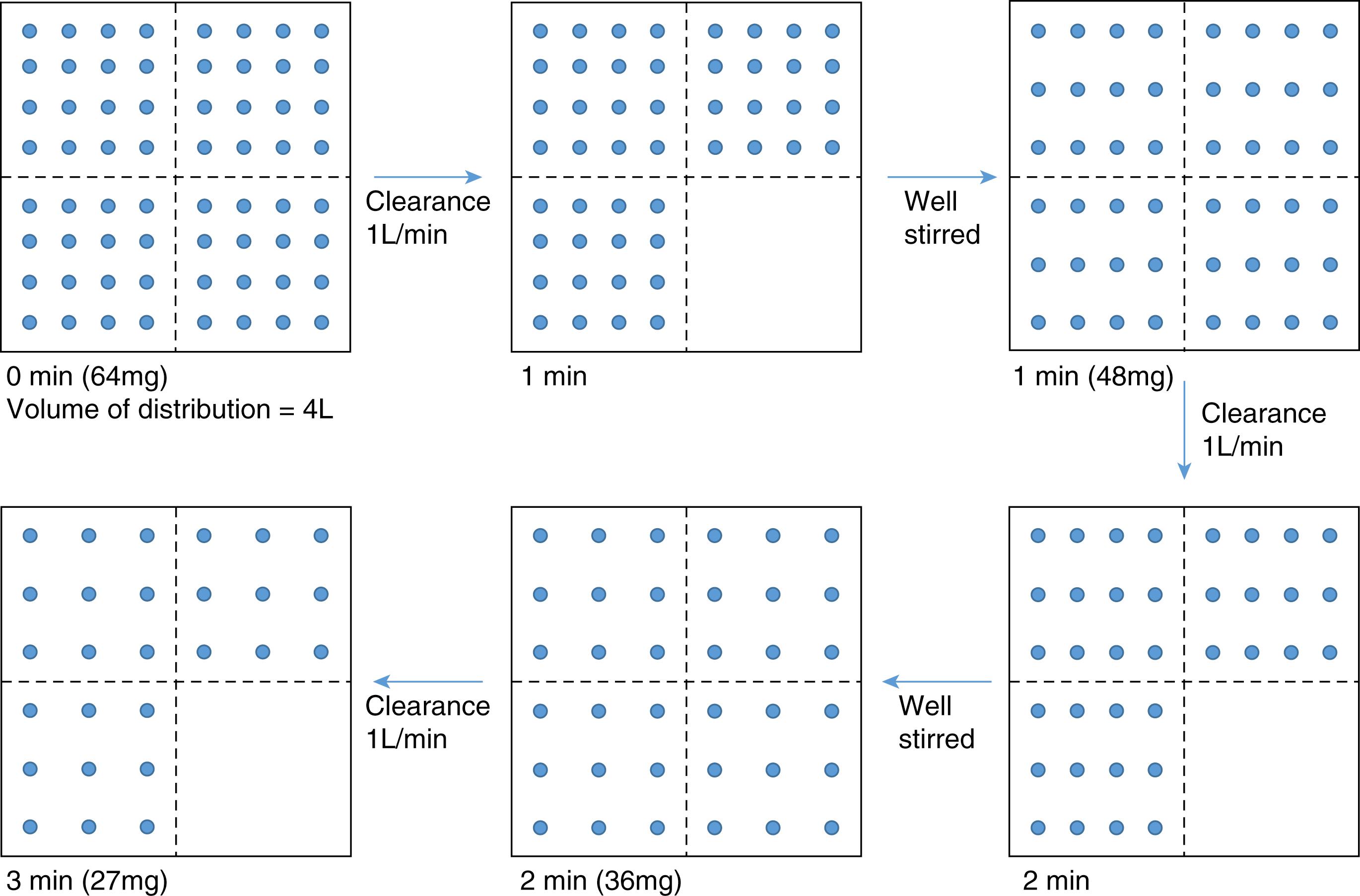
To illustrate the relationship between clearance and volume of distribution, consider the following simulation using a generic drug dosed in milligrams into a single compartment (tank) representing the distribution volume that has a clearance of 1 L/min. Assume that when drug is administered, the tank is well stirred and has instantaneous mixing throughout the entire volume. Assume the distribution volume is 4 L, the total dose of drug is 64 mg, and that drug elimination is proportional to the amount of drug present inside the tank at any given time. This rate of drug elimination is known as first-order elimination kinetics. When the drug is injected into the 4 L distribution volume, the drug will instantly evenly distribute throughout the compartment ( Fig. 18.8 ). With a clearance of 1 L/min, the amount of drug distributed to one fourth of compartment volume (1 L) will be cleared every minute. In the first minute, 16 mg drug is cleared. The remaining 48 mg will be redistributed evenly throughout the compartment. For the next minute, one fourth of the compartment volume (1 L) is again cleared. In the second minute, 12 mg of drug is cleared. This process repeats every minute. When assuming instantaneously mixing, the ratio of the amount of drug removed within the cleared portion of the distribution volume to the amount of drug within the total distribution volume will remain the same as illustrated in Eq. (18.7) .

This ratio, known as the elimination rate constant (k), is described in Eq. (18.8) .

Where CL is clearance with units of volume/time (L/min), Vd is the compartment distribution volume with units of liters (L), and k is the first-order elimination rate constant with units of inverse time (min ^–1 ).

Physiologic Model for Clearance

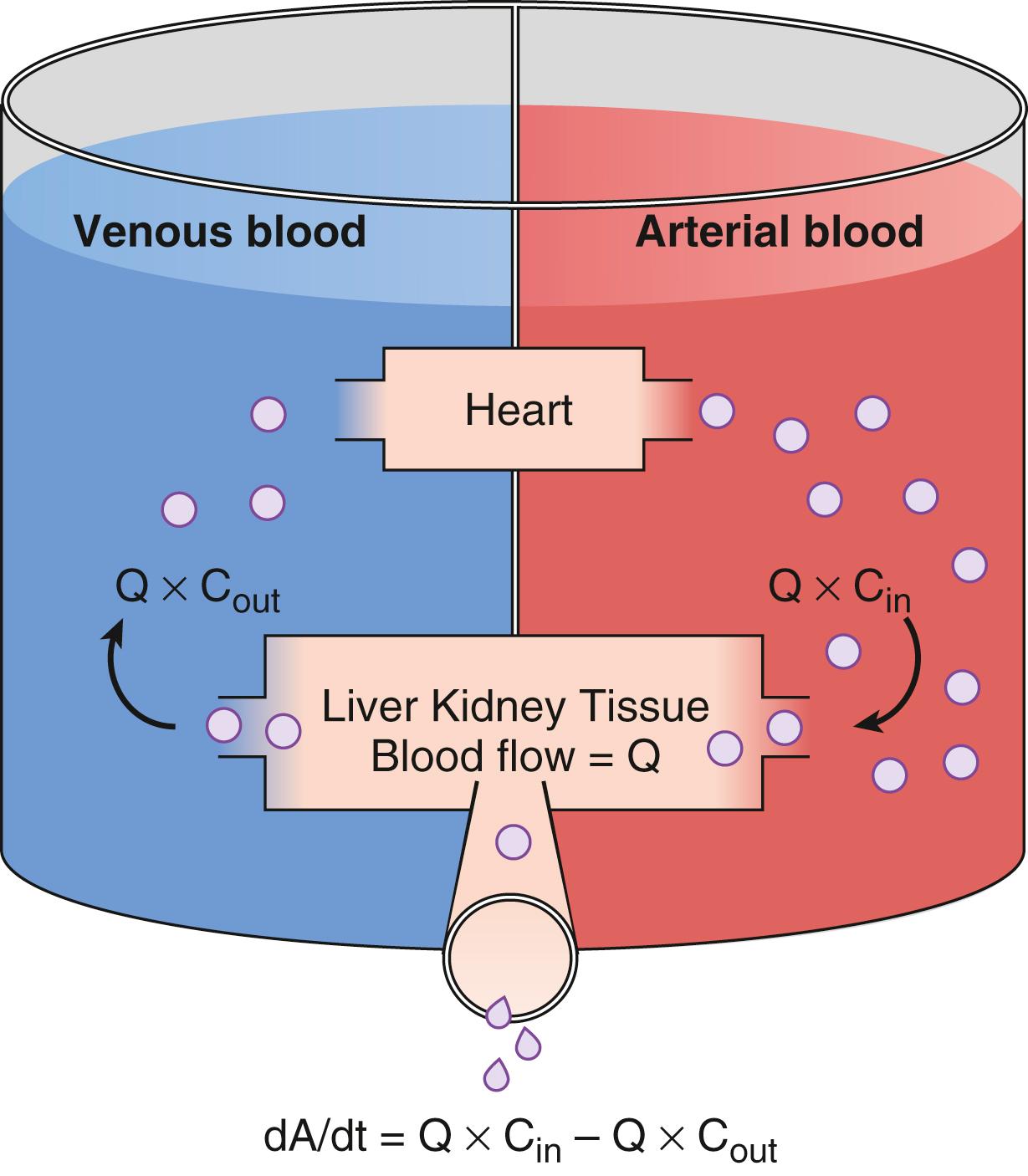
Drug extraction by metabolic organs is illustrated in Fig. 18.9 . This model contains a metabolic organ system responsible for drug elimination. According to mass balance, the rate at which drug flows out of metabolic organs is the rate at which drug flows into them minus the metabolic rate. The elimination rate (dA/dt) can be expressed as Q (C _in – C _out ). Rearranging C(t) in Eq. (18.3) with C _in , clearance can be expressed as

where Q is the blood flow to metabolic organs, C _in is the concentration of drug delivered to metabolic organs, and C _out is the concentration of drug leaving metabolic organs.

The fraction of inflowing drug extracted by the organ is $\frac{{\text{C}}_{\text{in}}-{\text{C}}_{\text{out}}}{{\text{C}}_{\text{in}}}$ . This is called the extraction ratio (ER). Clearance can be estimated as organ blood flow multiplied by the ER. Eq. (18.9) can be simplified to

The total clearance is the sum of each clearance by metabolic organs such as the liver, kidney, and other tissues.

Hepatic clearance has been well characterized. For example, the relationship among clearance, liver blood flow, and the ER is presented in Fig. 18.10 . For drugs with an ER of nearly 1 (e.g., propofol), a change in liver blood flow produces a nearly proportional change in clearance. For drugs with a low ER (e.g., alfentanil), clearance is nearly independent of the rate of liver blood flow. If nearly 100% of the drug is extracted by the liver, this implies that the liver has a very large metabolic capacity for the drug. In this case, the rate-limiting step in metabolism is the flow of drug to the liver, and such drugs are said to be “flow limited.” As a consequence, any reduction in liver blood flow due to circulatory effects of anesthetic agents or changes in circulatory volumes in cases of perioperative bleeding or other situations of excessive fluid loss can be expected to reduce liver-dependent drug clearance. However, moderate changes in hepatic metabolic function per se will have little impact on clearance because hepatic metabolic capacity is overwhelmingly in excess of demand.

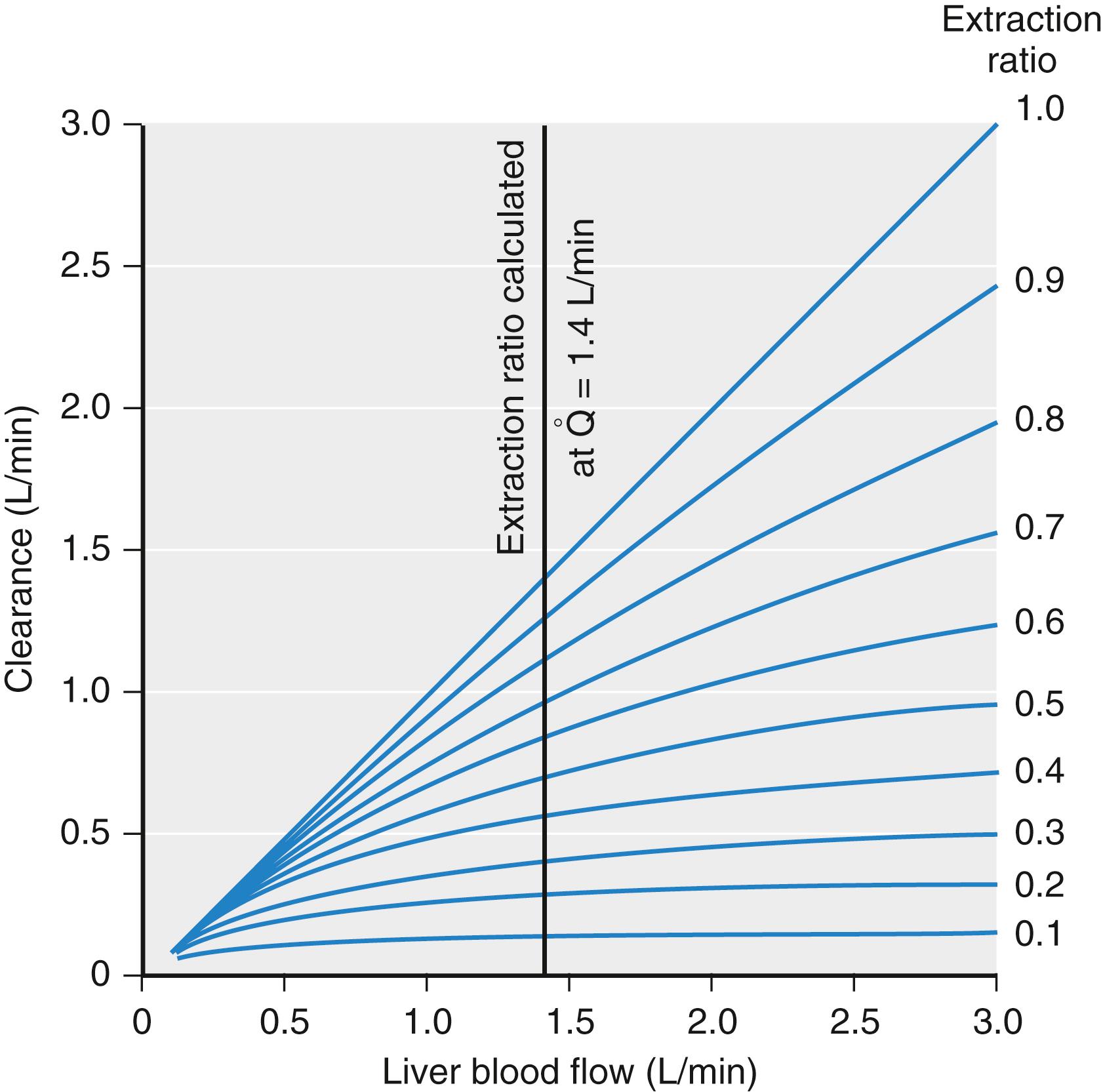
For many drugs (e.g., alfentanil), the ER is considerably less than 1. For these drugs, clearance is limited by the capacity of the liver to take up and metabolize drug. These drugs are said to be “capacity limited.” Clearance will change in response to any change in the capacity of the liver to metabolize such drugs, as might be caused by liver disease or enzymatic induction. However, changes in liver blood flow caused by the anesthetic regimen or other changes in splanchnic circulation usually have little influence on clearance because the liver handles only a fraction of the drug that it sees.

Although most anesthetic drugs are cleared by hepatic metabolism, remifentanil, succinylcholine, and esmolol are cleared in the plasma and tissues by ester hydrolysis, and pancuronium is cleared via the kidney. The relationship between metabolism and clearance is complex.

Hepatic Biotransformation

Most anesthetic drugs are cleared by hepatic biotransformation. The synthetic pathways for biotransformation are covered in detail in many biochemistry texts. Briefly, the liver metabolizes drugs through oxidation, reduction, hydrolysis, or conjugation. Oxidation and reduction occur in the cytochrome P450 system. These enzymes can be induced by exposure to certain drugs (e.g., the herbal remedy St. John’s wort) and increase the liver’s intrinsic metabolic capacity. Other drugs or hepatic disease can inhibit these enzymes (e.g., selected calcium channel blockers and selected antibiotics). Routes of oxidative metabolism include hydroxylation, dealkylation, deamination, desulfuration, epoxidation, and dehalogenation. Conjugation and hydrolysis often occur outside the P450 system, although glucuronidation involves the P450 system as well. The effect of conjugation is to transform hydrophobic molecules into water-soluble molecules through the addition of polar groups and thus render the metabolites easier to excrete via the kidneys. The metabolites generated by the liver are generally inactive, although some drugs (e.g., morphine, midazolam) have metabolites that are as potent as the parent drug. Genetic polymorphism can occur in all of these pathways, and this accounts for part of the variability in clearance in the population.

Physiologic Models

Physiologic models are based on organ and tissue physiologic and anatomic data. Drug concentrations into and out of an organ, organ blood flow, and organ drug distribution volume are required. Capturing these metrics from all organs is nearly impossible in humans and very challenging in animal models. If obtained, this data is used to estimate volumes and clearances for each organ in the body. Individual organ models are assembled into a whole organism physiological model. Once assembled, the combined models are complex and mathematically cumbersome. They may not offer a better prediction of plasma drug concentrations over time than simple compartmental models. If the intent of the model is to explore the ability of possible dosing regimens to achieve therapeutic plasma drug concentrations, compartmental models are usually adequate.

Compartmental Models

Compartmental models are built on the same basic concepts as physiologic models, but with significant simplifications. Compartment pharmacokinetic models are strictly empirical. They are based on fitting equations to measured plasma concentrations following a known dose. Kinetic models are transformed into models that characterize changes over time in terms of volumes and clearances. Part of the continuing popularity of pharmacokinetic models is that they can be transformed from an unintuitive exponential form to a more intuitive compartmental form as shown in Fig. 18.11 .

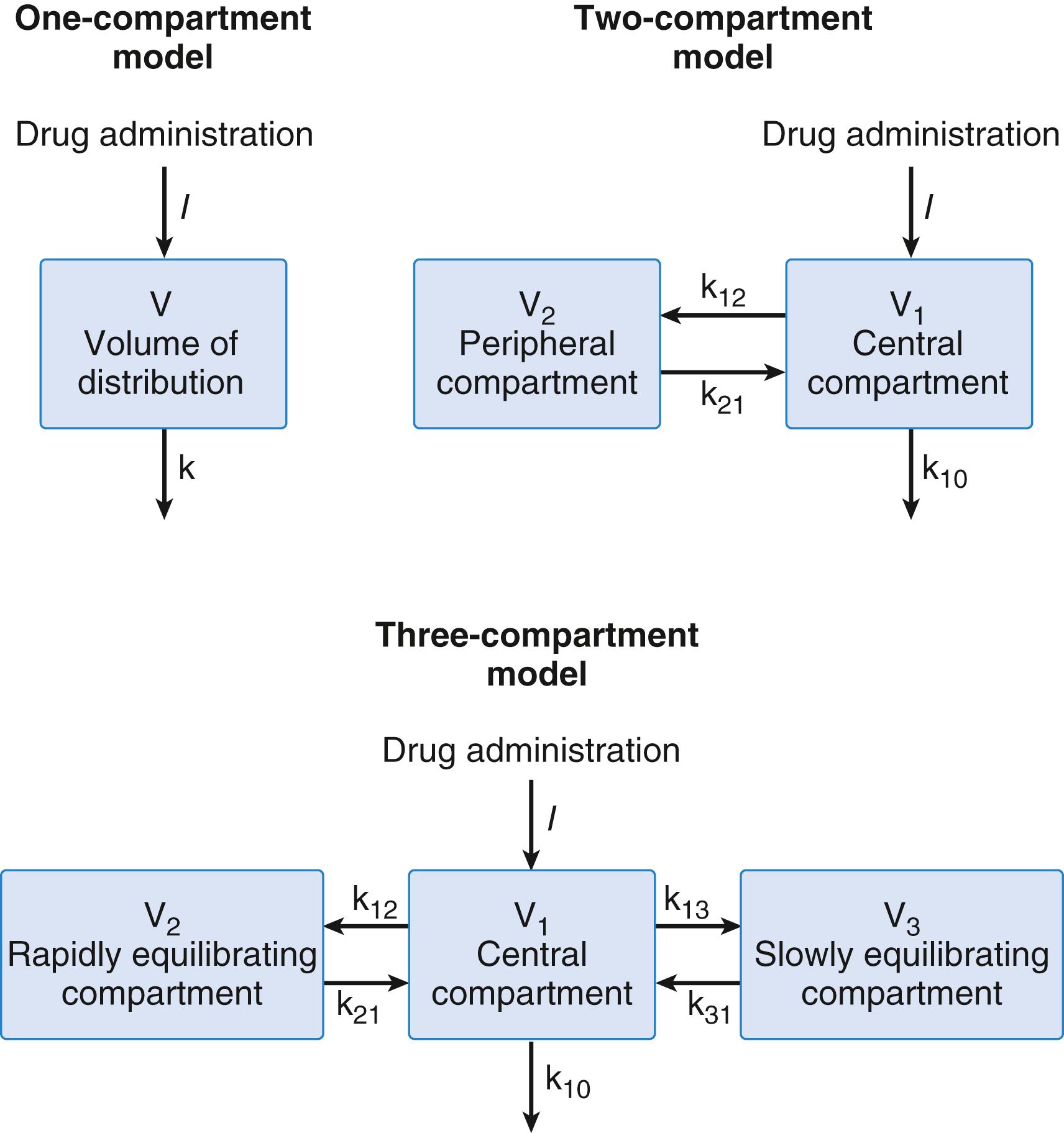
Compartment models used to describe anesthetic drugs typically consist of one, two, or three compartments corresponding to the number of exponents in an equation needed to best fit the plasma versus concentration data (see Fig. 18.11 ). Exponents are difficult to work with and have little clinical meaning. Thus, they are converted into fictitious volumes and clearances. For example, in a three-compartment model, there is a central compartment and two peripheral compartments. The sum of the all volumes is the volume of distribution at steady state. Drug clearance from the central compartment to the outside is the central clearance. Central clearance accounts for both metabolism and excretion. Clearances between the central and peripheral compartments is the “intercompartmental” clearance. Micro rate constants, expressed as k _ij , define the rate of drug transfer from compartment i to compartment j. For example, k ₁₀ is the micro rate constant describing drug transfer from central compartment to the outside. The intercompartmental micro rate constants (k ₁₂ , k ₂₁ , etc.) describe movement of drug between the central and peripheral compartments. Each peripheral compartment has at least two micro rate constants, one for drug entry and one for drug exit. The micro rate constants for the two- and three-compartment models are presented in Fig. 18.11 .

Zero Versus First-Order Kinetics

Drug elimination can have one of two profiles, zero- and first-order kinetics. With zero-order kinetics, drug is eliminated at a constant rate. With first-order kinetics, drug is eliminated at a rate proportional to the amount of drug present at that time. These rates are expressed using the following equations.

where A(t) is the amount of drug at time t, dA(t) is the change in drug amount at time t. −k ₀ is the zero-order elimination rate constant. Its units are mass/time (e.g., mg/min). −k ₁ is the first-order elimination rate constant. Its units are the reciprocal time 1/time, (e.g., min ^–1 ). Most anesthetic drugs have first-order kinetics. When the processes responsible for metabolism are saturated, the kinetics could change from first to zero order.