Physical Address
304 North Cardinal St.
Dorchester Center, MA 02124
Many analytic determinations made in clinical laboratories are based on measurements of radiant energy that is absorbed or transmitted. The devices used to measure absorbed or transmitted light energy are photometers and spectrophotometers.
The basic components of spectrophotometers include a radiant energy source, wavelength selector, cuvette holder, photodetector, signal processors, and readout devices.
A reflectometer is used to measure analytes by measuring the quantity of light reflected by a liquid sample that has been dispensed onto a grainy or fibrous solid support.
Nephelometers are used to detect light that is scattered at various angles, whereas turbidimetry measures a reduction in light transmission due to particle formation.
A flow cytometer measures light patterns produced as particles pass single file through a laser light beam. The flow cytometer is used to count and sort cells. It is also a key component of hematology analyzers and a technology used to differentiate white blood cells.
Electrochemical principles are used to measure numerous analytes in biologic fluids. Specific electrochemical techniques include potentiometry, amperometry, coulometry, conductivity, and anodic stripping voltammetry.
Analytes measured by electrochemical techniques include electrolytes, blood gases, pH, metabolites (e.g., glucose, urea nitrogen), ionized calcium, lead, and chloride in sweat.
Chromatography is a separation technique based on the different interactions of specimen compounds with a mobile phase and a stationary phase, as the compound travels through a support medium.
Mass spectrometers have become increasingly important clinical instruments, especially in emerging fields such as proteomics. Mass spectrometry is based on fragmentation and ionization of molecules. The relative abundance of each of the ions yields a characteristic mass spectrum of the parent molecule. The basic components of a mass spectrometer include an ion source, a mass analyzer, and an ion detector.
A fundamental understanding of the principles of instrumentation used in clinical laboratories is essential. These instruments must provide the clinician with the best possible data to be of value to the patient. Without a thorough understanding of the necessary principles associated with an analyzer, operators will be ill equipped to perform maintenance procedures and calibrations and to troubleshoot problems that may arise.
The goal of this chapter is to provide the reader with a brief and broad description of the essential principles of analytic instruments in the clinical laboratory. For a more comprehensive review of this topic, the reader is referred to references on clinical instrumentation at the end of this chapter.
Absorption spectroscopy has provided scientists with a means to use both qualitative and quantitative methods of measuring analytes in body fluids. Bouguer initially developed the principles of absorption spectroscopy in the early 1700s. Two other scientists, Lambert and Beer, continued to develop the fundamental principles of absorption spectroscopy, commonly known as Beer’s law. Before reviewing the laws of spectroscopy, it is prudent to gain an understanding of light and the effects of its interactions with matter.
Diverse spectrophotometric methods use electromagnetic radiation (EMR), which can take several forms, the most recognizable being light and radiant heat. Other types of EMR include gamma (γ) rays and x-rays, as well as microwaves, radiofrequency radiation, and ultraviolet radiation. The energies involved with specific regions of the electromagnetic spectrum (EMS) and their corresponding wavelengths change dramatically from radio waves to γ radiation. All types of EMR have two component waves that travel at right angles to one another, an electric and a magnetic component, as illustrated in Figure 4.1A .
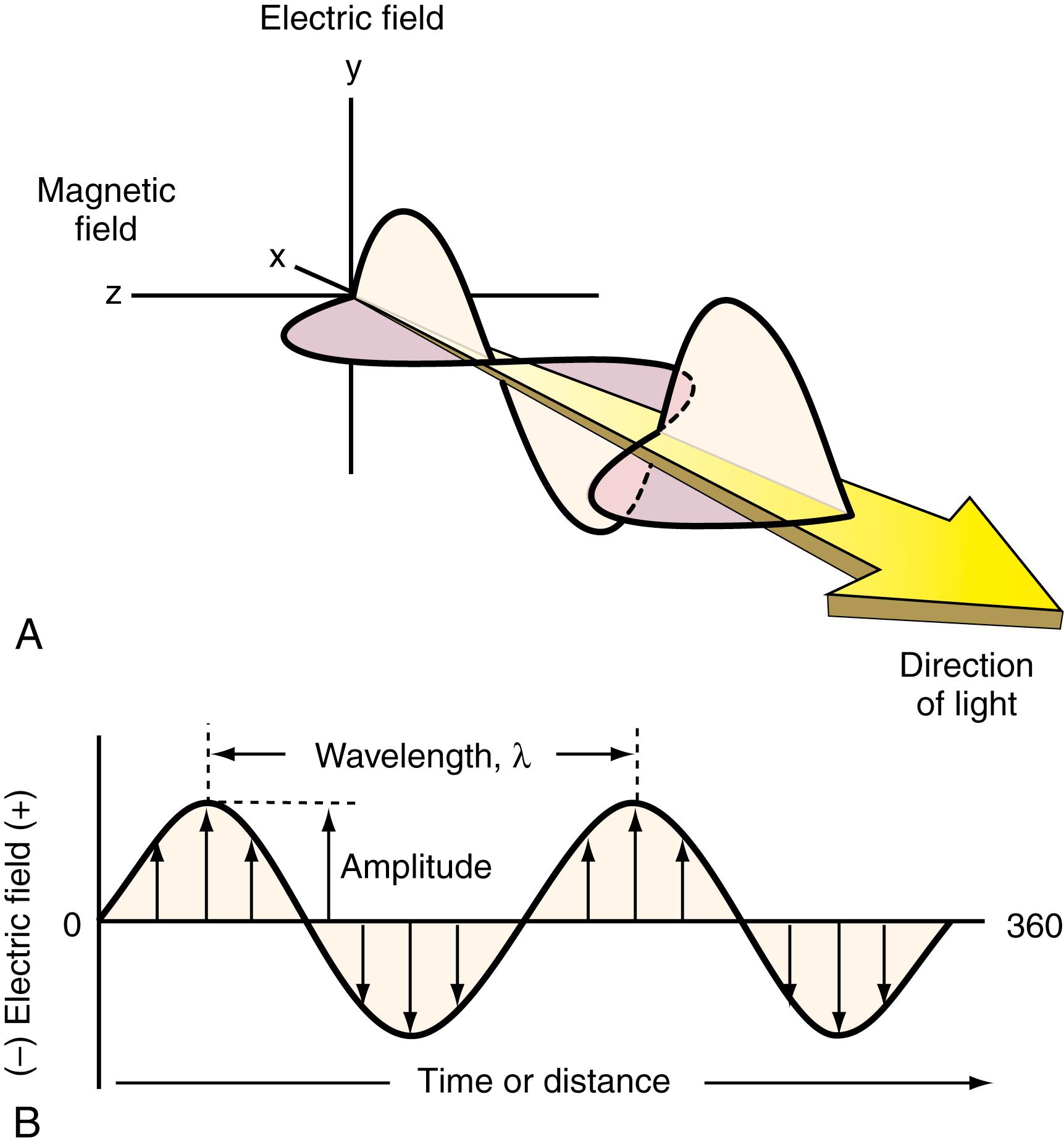
Some properties of EMR can be described by means of a classical sinusoidal wave model. Parameters associated with this waveform include wavelength, frequency, velocity, and amplitude, as shown in Figure 4.1B . EMR requires no supporting medium for its transmission and passes readily through a vacuum.
The sine wave model does not provide the total picture when discussing the absorption and emission of radiant energy. EMR also exists as a stream of discrete particles, or packets, of energy called photons . The energy of the photons is proportional to the frequency of the radiation. This dual nature of EMR is considered complementary and applies to the behavior of streams of electrons, protons, and other elementary particles.
The wave model allows us to represent EMR as both an electrical and a magnetic field that can undergo in-phase, sinusoidal oscillation at right angles both to each other and to the direction of propagation. The electrical and magnetic fields for a monochromatic beam of plane-polarized light (with oscillation of either the electric or magnetic fields within a single plane) ∗ in a specific direction of propagation are shown in Figure 4.1A ( ). The electrical vector or component of the waveform is shown in two-dimensional format. Remember that a vector has both magnitude and direction. The electrical field vector at a certain point in time and space is proportional to its own magnitude.
Time or distance of wave propagation is plotted on the abscissa. Most of the instrument principles widely used in the laboratory involve the electrical component of radiation and will represent the focus of discussion throughout this chapter. An exception will be nuclear magnetic resonance (NMR). With this technique, the magnetic component produces the desired effect.
Several wave parameters will be described. Amplitude of the sine wave is shown as the length of the electronic vector at maximum peak height. A period , p , is defined as the time in seconds required for the passage of successive maxima or minima through a fixed point in space. The number of oscillations of the waveform in a second is called frequency , v . The unit of frequency is hertz (Hz), which corresponds to one cycle per second. Frequency is also equal to 1/ p . A wavelength , λ, is the linear distance between any two equivalent points on a successive wave ( Box 4.1 ). A widely used unit for wavelength in the visible spectrum is the nanometer, nm (10 −9 m) ( Box 4.1 ). Electromagnetic radiation in the x-rays or gamma region may be expressed in terms of angstrom units, Å (10 −10 m). Finally, because of its much longer wavelength, EMR in the infrared region may have units corresponding to the micrometer, μm (10 −6 m).
Velocity of propagation, v i in meters per second, is determined by multiplying frequency by wavelength. Thus:
The frequency of light is determined by the source and does not change, whereas the velocity depends on the composition of the medium through which it passes. Therefore, Equation 4.1 implies that the wavelength of radiation is also dependent on the medium.
The velocity of light traveling through a vacuum is independent of wavelength and is at its maximum. This velocity is represented by the symbol c and is equivalent to 2.99792 × 10 8 m/s. Equation 4.1 can then be rewritten as follows:
In any medium containing matter, the propagation of radiation is slowed by the interaction between the EMR field of the radiation and the electrons bound in the atoms of that matter. Because the radiant frequency does not vary and is fixed by the source, the wavelength must decrease as radiation passes from air to a slower medium ( Box 4.2 ).
One should note the following safety concern: Optical devices that emit high-frequency EMR generate very high energies and can damage the eyes. Examples include deuterium and xenon lamps.
Wavelength and frequency are related to the energy of a photon, E , by Planck’s ∗ constant h , 6.626 × 10 −34 J s, and c , the velocity of light in a vacuum (3.00 × 10 8 ms –1 ):
where E = energy of a photon in joules or eV, h = Planck’s constant (6.626 × 10 −34 J s), and v = frequency in Hz (cycles/s).
Quite often, the energies of photons are stated in terms of an electron volt (eV). An eV is defined as the energy acquired by an electron that has been accelerated through a potential of 1 volt. The conversion factors between joules and eV are as follows:
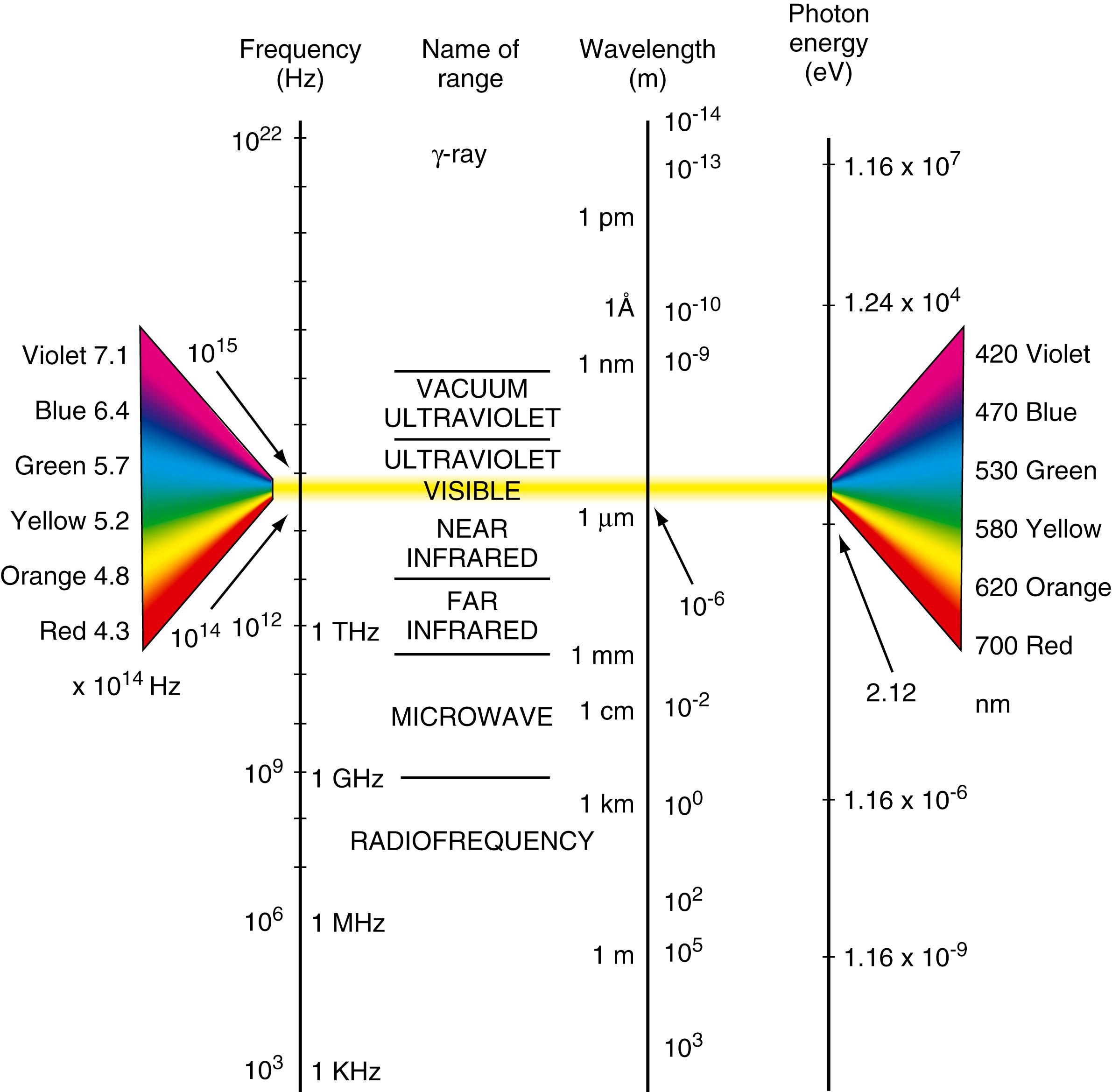
To illustrate the difference in energies of photons in the EMS, compare the energy of photons in the ultraviolet (UV) region versus the visible region of the EMS ( Box 4.3 ).
The relationship of frequency, wavelength, and photon energy throughout the EMS can be seen in Figure 4.2 . This figure illustrates that very-high-energy gamma photons have extremely short wavelengths (top of Fig. 4.2 ), on the order of 1 × 10 -13 m or 1 × 10 -4 nm, and very high frequencies, on the order of 1 × 10 22 Hz ( ). The converse is true for television and radio wave parameters shown toward the bottom of Figure 4.2 .
Transmission of radiation in matter can be viewed as a momentary retention of the radiant energy by atoms, ions, or molecules followed by reemission of the radiation in all directions as particles return to their original state. Destructive interference removes most but not all of the reemitted radiation involving atomic or molecular particles that are small relative to the wavelength of the radiation. The exception is radiation that travels in the original direction of the beam; the path of the beam appears to be unaltered because of the interaction. It has been shown that a very small fraction of the radiation is transmitted at all angles from the original path and that the intensity of this scattered radiation increases with particle size.
Scattering by molecules or aggregates of molecules with dimensions significantly smaller than the wavelength of the radiation is referred to as Rayleigh scattering . The intensity is proportional to the inverse fourth power of the wavelength, the square of the polarizability of the particles, and the dimensions of the scattering particles. In Rayleigh scattering, the wavelengths of absorbed and emitted photons are the same. An example of Rayleigh scattering is the blue color of the sky, which results from increased scattering of the shorter wavelength of the visible spectrum.
The Tyndall effect occurs with particles of colloidal dimensions and can be seen with the naked eye. Measurements of scattered radiation are used to determine the size and shape of polymer molecules and colloidal particles.
Raman scatter involves absorption of photons producing vibrational excitation. Emission or scatter occurs at longer wavelengths. Raman scatter always varies from the excitation energy by a constant energy difference.
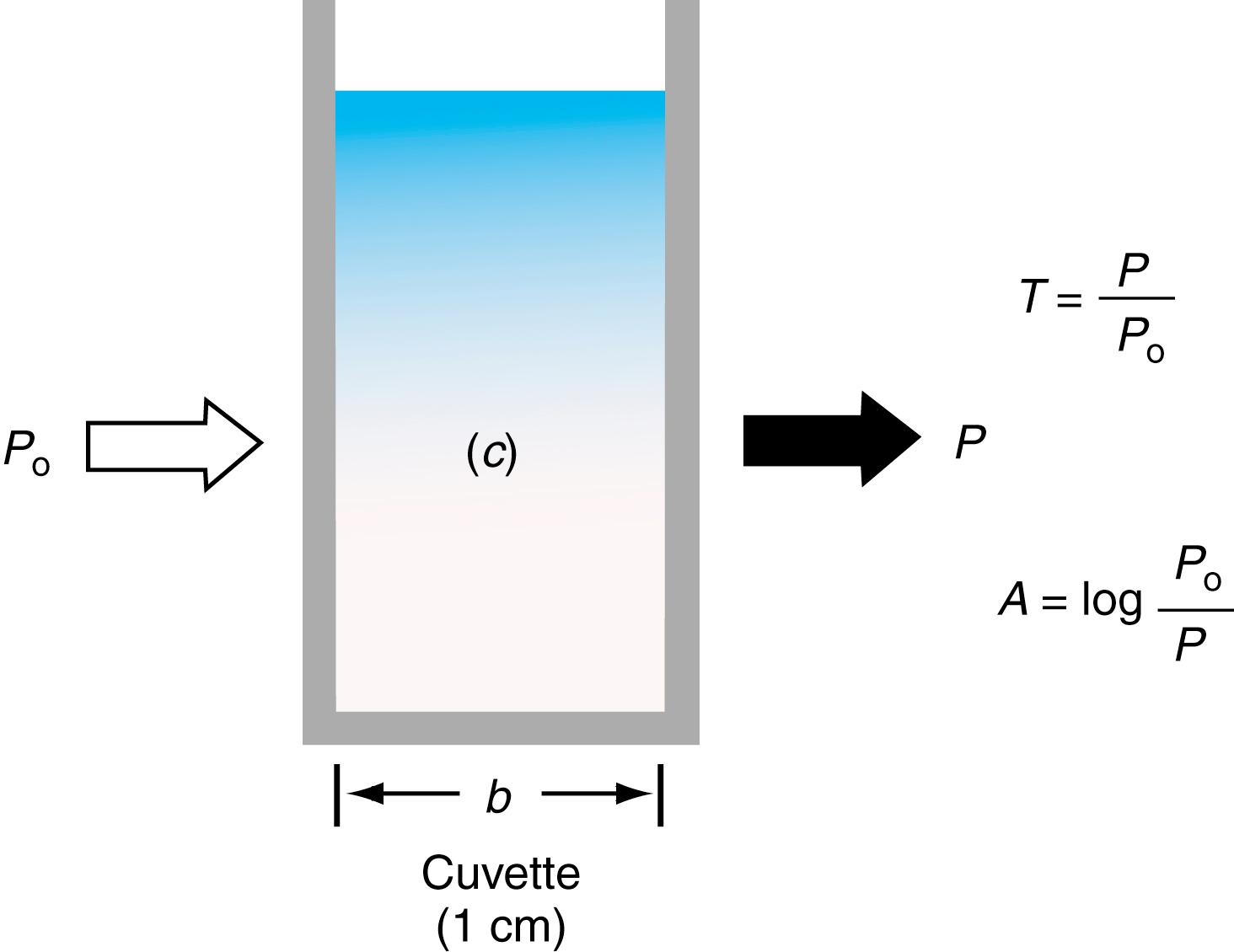
Light that has a single wavelength is referred to as monochromatic light. Suppose that monochromatic light, which has an intensity, P o , is directed toward a cuvette containing a compound that absorbs light at the monochromatic wavelength. Some of the light will be absorbed; the light that is not absorbed will be transmitted through the cuvette. The intensity of this transmitted light is P. We define the transmittance, T, as:
Lambert proved that for monochromatic radiation that passes through an absorber of constant concentration, a logarithmic decrease is seen in the radiant power as the path length increases arithmetically. The absorbance ( A ) of a solution was determined to be equivalent to:
The relationship between transmittance, T , or percent transmittance ( P / P o × 100), and absorbance is shown in Figure 4.3 . Absorbance and percent transmittance ( Box 4.4 ) are inversely related as given by the following:
Beer followed with studies on the relationship between radiant power and concentration. His approach was to keep the path length and wavelength constant while determining the relationship of radiant power, P, and concentrations of the absorbing species. Based on previous work by Lambert, Beer discovered that for monochromatic radiation, absorbance is directly proportional to the path length, b , through the medium and the concentration, c , of the absorbing species. The work culminated in the Beer-Lambert law or, simply, Beer’s law.
The principles established are represented by the following:
where a = absorptivity in L g –1 cm –1 , b = path length of 1 cm, and c = concentration in units of g/L.
When the concentration in Equation 4.7 is expressed in moles per liter and the path length in centimeters, the term applied is molar absorptivity , given the symbol ε, epsilon, and is equivalent to the molar extinction coefficient (“ a ”) times gram molecular weight of the absorbing species.
where units for ε are L mol –1 cm –1 .
The graph of absorbance versus concentration shows the intercept at zero and a linear plot with a slope equivalent to “ ab .” Absorption spectroscopy is used best for solutions whose absorbance values are less than 2.0. Absorbance values greater than 2.0 may yield erroneous results because of other interactions of light with matter (e.g., variations in the refractive index of a solution). This may cause a deviation from Beer’s law, resulting in bending of the linear plot as shown in Figure 4.4 .
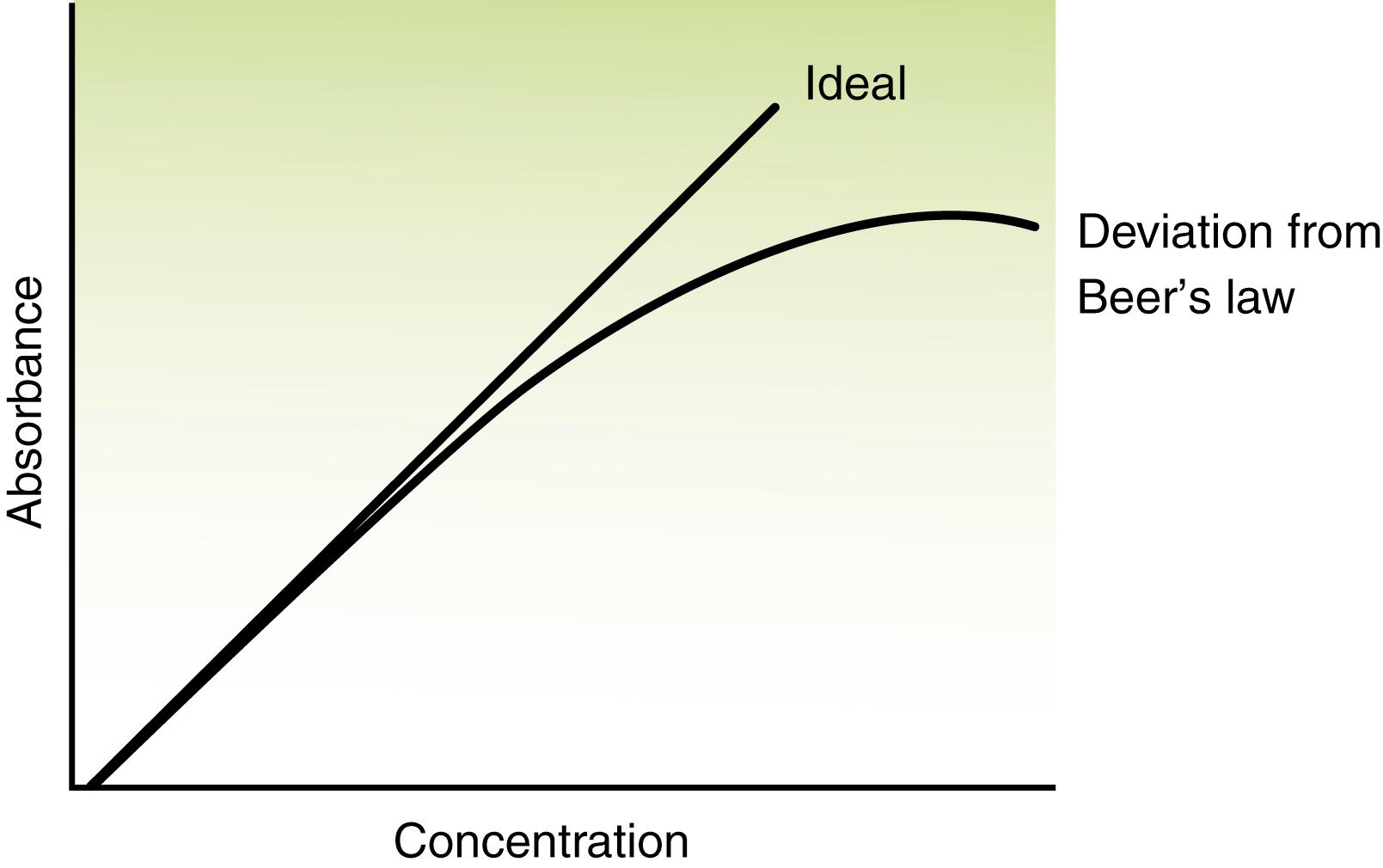
Deviations from Beer’s law may be caused by changes in instrument functions or chemical reactions. Instrument deviation is commonly a result of the finite bandpass of the filter or monochromator. Beer’s law assumes monochromatic radiation, but truly monochromatic radiation is best achieved using only unique line emission sources. If absorptivity is constant over the instrument bandpass, then Beer’s law is followed within close limits.
Deviations from Beer’s law become apparent at higher concentration (i.e., higher absorbance). In Figure 4.4 , the curved line bends toward the concentration axis as opacity is approached. This lack of adherence to Beer’s law in a negative direction is undesirable because of the somewhat large increase in the relative concentration error.
Deviation from Beer’s law can occur with shifts or changes in chemical or physical equilibrium involving the absorbing species. Changes in solution pH, ionic strength, and temperature can result in such deviations.
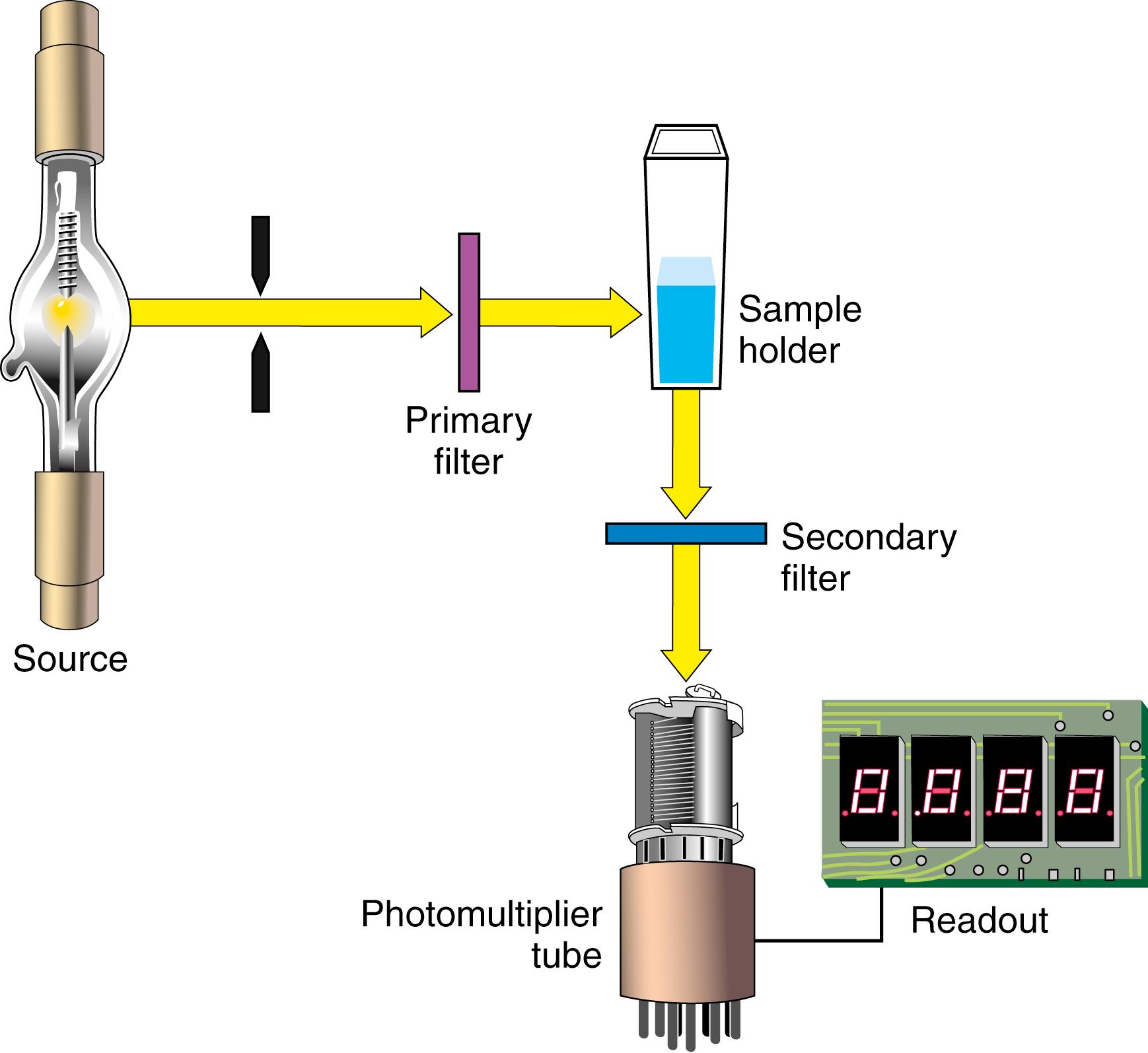
A typical photometer or spectrophotometer contains six basic components in a single- or double-beam configuration. These components include a stable source of radiant energy; a filter that isolates a specific region of the EMS; a sample holder (cuvette); a radiation detector, called the photomultiplier; a signal processor; and a readout device. Each component of a typical photometer is shown in Figure 4.5 .
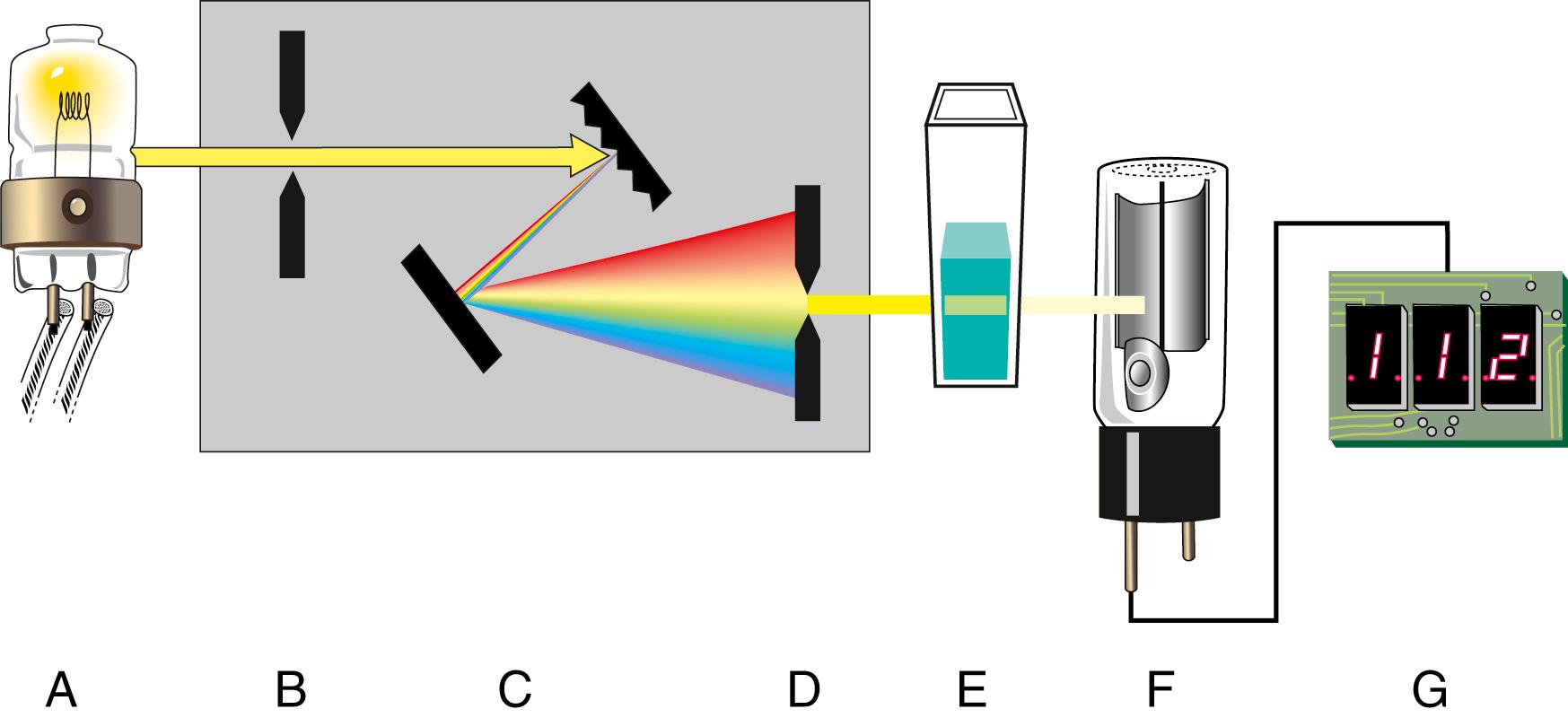
The following steps outline the function of each component in any absorption-type photometer as it detects light and provides information to the operator:
The light source provides the energy that the sample will modify or attenuate by absorption. The light is polychromatic (i.e., all visible wavelengths are present).
A wavelength selector or filter isolates a portion of the spectrum emitted by the source and focuses it on the sample.
The sample in a suitable container (e.g., a cuvette) absorbs a fraction of the incident light and transmits the remainder.
The light that passes through the cuvette and sample strikes the cathode of a photodetector and generates an electrical signal.
The electrical signal is processed electronically (e.g., amplified, digitized).
The processed signal is electronically coupled to the display unit (e.g., light-emitting diode [LED], X-Y strip chart recorder, meter).
Radiant energy sources or lamps provide polychromatic light and must generate sufficient radiant energy or power to measure the analyte of interest. A regulated power supply is required to provide a stable and constant source of voltage for the lamp. Radiant energy sources are of two types: continuum and line . A continuum source emits radiation that changes in intensity very slowly as a function of wavelength. Line sources emit a limited number of discrete lines or bands of radiation, each of which spans a limited range of wavelengths.
Continuum sources find wide applications in the laboratory. Examples of continuum sources include tungsten, deuterium, and xenon. For testing in the visible region of the EMS, tungsten or tungsten-halogen lamps are widely used. The filament in the tungsten-halogen lamp is maintained at a higher temperature than in the normal tungsten lamp. This takes advantage of the Wien and Stefan laws to provide a source with a maximum wavelength near the center of the visible spectrum (whiter), and with a greater energy output (brighter). Introducing a halogen gas into the lamp envelope counteracts the problem of increased atom vaporization from the high-temperature filament.
The deuterium lamp is routinely used to provide UV radiation in analytic spectrometers. The voltage applied is typically about 100 volts, which gives the electrons enough energy to excite the deuterium atoms in a low-pressure gas to emit photons across the full UV range.
The strong atomic interaction in a high-pressure xenon discharge lamp produces a continuous source of radiation, which covers both the UV and the visible range. The discharge light is normally pulsed for short periods with a frequency that determines the average intensity of the light from the source and also its lifetime.
In atomic emission line sources, electrons move between atomic energy levels. If the atom is free of any interaction with other atoms, the amount of energy liberated can be very precise, and all of the photons share a very clearly defined wavelength. This is the characteristic sharp “line” emission from an electronic discharge in a low-pressure gas. Line sources that emit a few discrete lines find wide use in atomic absorption, molecular, and fluorescent spectroscopy. Mercury and sodium vapor lamps provide sharp lines in the ultraviolet and visible regions and are used in several spectrophotometers. For atomic absorption spectroscopy applications, the hollow cathode lamp provides atomic emission from free metal atoms by using the heat of a gaseous discharge (e.g., in neon) to vaporize metal atoms into the discharge. Typically, each lamp is specific to a particular metal. However, some lamps use two, three, or more metals, although the emission from each of these is reduced.
A laser source is very useful in analytic instrumentation because of its high intensity and narrow bandwidth and the coherent nature of its outputs. Several specific uses of lasers include high-resolution spectroscopy, kinetic studies of processes with lifetimes in the range of 10 −9 to 10 −12 s, as light sources for nephelometers, and as ionization inducers in the MALDI and SELDI mass spectrometric techniques (see Chapter 5 ).
A critical component of all spectrometers is the device used to select the appropriate wavelength. Several types of wavelength selectors are available, including filters, prisms, grating monochromators, and holographic gratings. The quality of these selectors is described by their nominal wavelengths, spectral bandwidths, and bandpass. Nominal wavelength represents the wavelength in nanometers at peak transmittance. Spectral bandwidth is the range of wavelengths above one-half peak transmittance. It is sometimes called the half power point or full width at half peak maximum (FWHM). The total range of wavelengths transmitted is the bandpass . Figure 4.6 summarizes these characteristics of wavelength selectors. This figure also summarizes the difference in wavelength selectivity between interference ( Fig. 4.6A , left) and absorbance filters ( Fig. 4.6B , right), as we now discuss.
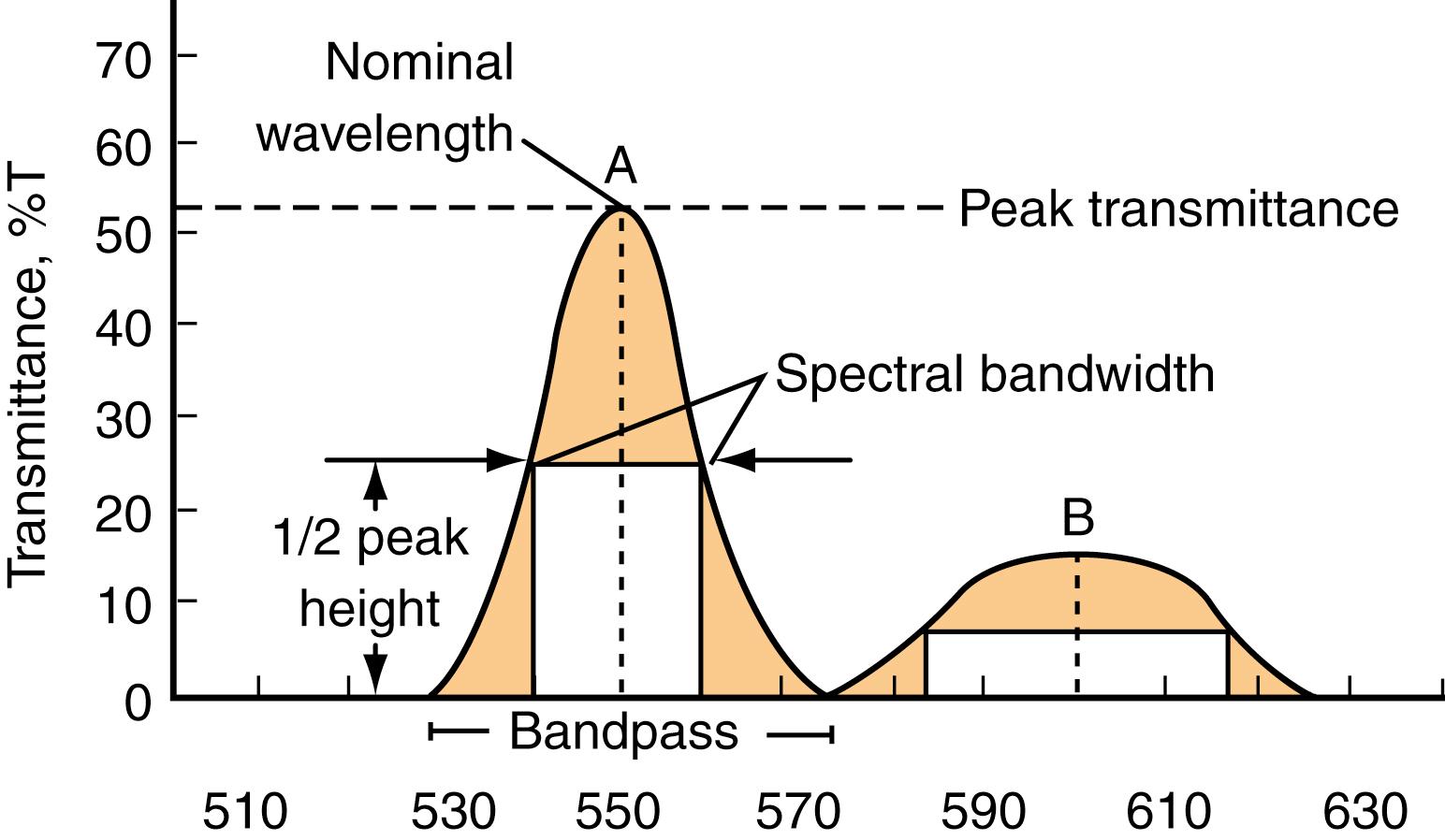
An important component of the spectrophotometer is the wavelength filter. Its purpose is to isolate monochromatic light, or at least as narrow a range of wavelengths of light as possible, and direct it to the sample or to the photodetector. Remember, the purpose of absorption spectroscopy is to detect how much light is absorbed by the sample. This is a measure of the absorbance of an analyte in a sample that is directly proportional to concentration (see Beer’s law discussed earlier). The percent transmittance is the ratio of the amount of light transmitted through the sample to the amount of light transmitted in the absence of the sample (e.g., buffer or solvent without the sample). Theoretically, it should be possible to determine this ratio without any filter at all. However, under these circumstances, most of the light that enters the sample in the cuvette will not be absorbed. Thus, to determine absorbance, it would be necessary to measure a small difference between two large numbers (i.e., the amount of light transmitted in the absence [control] and the presence of the sample). Therefore, to obviate this condition, it becomes necessary to filter out as much “extraneous” light as possible to enable accurate measurements of absorbance.
Two approaches may be taken to filtering light waves. The first is to limit the wavelengths of light that enter the sample with appropriate filters, so-called presample filters. The second is to allow multiwavelength light to pass through the sample and then to break up this light into its component wavelengths using a high-resolution prism and focus each of these component wavelengths on specific detectors, essentially one for each of the component wavelengths. If a sample absorbs light at one wavelength, then the ratio of the light transmittance of this wavelength of the sample to that for the control can be used to compute the percent transmittance and absorbance. This arrangement is termed postsample filtering .
Presample Filters ( ). Fundamentally, two types of these filters are used: absorption and interference filters. Absorption filters simply absorb regions of the EMS and allow only a limited domain of this spectrum to pass through to the sample cuvette. The simplest sort of absorption filters consists of different forms of glass. One form of glass allows transmission only of wavelengths of light greater than 400 nm, while a second form allows transmission only of wavelengths of light less than 600 nm. If both glasses are arranged serially, they allow for transmission of light of wavelengths of only between 400 and 600 nm, with a peak at 500 nm. As a refinement of this approach, the older spectrophotometers utilize colored glass or transparent plastic materials. These colors are arranged in a circle or wheel so that light of a relatively narrow range of wavelengths is transmitted when each colored sector is in the light path. For example, if light in the green portion of the visible spectrum is desired, the wheel is turned so that the green filter is placed in the light path. The spectral bandwidth of these absorption filters ranges from about 30 to 50 nm (see Fig. 4.6 ).
This bandwidth is rather large but is adequate for determining the concentration of compounds in solution; absorbance filters are less adequate for scanning the absorption spectra of individual compounds and macromolecules or for accurately determining low concentrations of molecules. To achieve the latter goals, it becomes necessary to use interference filters.
In the simplest interference filter, light on the path to the sample cuvette enters a magnesium fluoride chamber that is coated with micro-mirrors on the interior. As shown in Figure 4.7A , light enters evenly spaced ports or slits on the left. Only light that enters each slit can continue; the rest is absorbed by the chamber. Light entering a slit, at an angle to the horizontal, travels across the chamber and is reflected back to the opposite wall. In Figure 4.7A , if the path length ab equals bc , and the path length equals the desired wavelength of light, it can be shown from the theory of optics that the light whose wavelength equals that of the path length or integral multiples of this path length will undergo constructive interference (i.e., the waves will add to one another exactly) with incoming light at slits above it. For example, in Figure 4.7A , light of wavelength L = ab , reflected from b to c , will be exactly in phase with light of wavelength L or integral multiples of L , entering the slit at c . All other wavelengths will destructively interfere with light entering slit c . The light is then reflected again at c to d and back again to e where, again, it will add to (constructively interfere with) only light waves of the same wavelength or integral multiples of it. Eventually, only light at the desired wavelength will be transmitted through the opposite wall at slits f and h .

It is important to note that because integral multiples of the wavelengths of incident light will constructively interfere with transmitted light in the chamber, more than one wavelength of light can be transmitted from the chamber. The actual quantitative relationship between the wavelengths ( L ) transmitted by the chamber; the angle of incidence, q , of the light entering a slit; the refractive index, R , of MgF 2 (1.38); and the distance, d , across the chamber is as follows:
where M is any integer. Note that this equation predicts that for any given distance, d , many wavelengths of integral multiples to the fundamental wavelength where M = 1 can be transmitted. In practice, many of these other wavelengths can be excluded by using such techniques as placing appropriate glass filters in series with the interference chamber.
This arrangement successfully transmits (close to) monochromatic light at a particular wavelength. However, it does not allow for scanning of wavelengths to obtain the absorption spectra of different samples. To accomplish this, a similar arrangement is used. In this case, however, advantage is taken of another principle from the theory of optics: the principle of light diffraction. As shown in Figure 4.7B , when monochromatic light is allowed to enter two slits on one surface of a chamber, very much like the one shown in Figure 4.7A except that there is no material (MgF 2 ) in the chamber, the two light waves interfere with each other such that if a screen is placed on the opposite surface of the chamber, discrete regions of light and dark bands will be seen ( ). The light bands are from light waves that have added constructively to one another and are transmitted; the dark bands result from destructive interference of the light waves, and no light waves are transmitted. The position of the bands and the distance between the bands depend on the wavelength of light that enters the two apertures and on the angle of incidence of both beams. Because, for a given angle of incidence, the position of the light bands for transmitted monochromatic light depends exclusively on the wavelength of light, if exit apertures are placed on the opposite surface only where the light bands occur for light of a desired wavelength, only light of that wavelength will be emitted from the chamber. This effect can be enhanced by placing multiple entry apertures next to one another in a sawtooth or grating pattern such that adjacent apertures are at a fixed distance, d , from one another, as shown in Figure 4.7B .
Now, polychromatic light is composed of multiple different monochromatic wavelengths of light. When polychromatic light is allowed to pass through the two apertures, as in Figure 4.7B , each of the wavelengths will constructively and destructively interfere with itself, as described earlier. Two different wavelengths of light will interfere only destructively with each other unless they are integral multiples of each other, as discussed in the previous case of the MgF 2 chamber. This allows us to “trap” the light of the desired wavelength by placing the exit apertures where the light bands are known to occur on the opposite surface for that monochromatic wavelength. A limiting factor for selecting truly monochromatic light is the size of the aperture that can allow some “stray” light from adjacent light bands for very close wavelengths to exit.
As with the MgF 2 interference chamber described previously, another source of contaminating light can occur from light of wavelengths that are integral multiples of the desired wavelength. The relationship that describes the wavelength as a function of the distance is similar to that for the MgF 2 chamber—that is,
Here, however, d is the distance between two adjacent entry slits on the near surface (toward the light source), not the distance across the chamber as with the MgF 2 chamber. Note that for a given distance, d , and angle of incidence, q , this equation has multiple solutions. For example, if the desired wavelength is 800 nm, d sin( q ) = 800. But this is for M = 1, L = 800 nm. Another solution is M = 2, L = 400 nm, and another M = 3, L = 266.67 nm, and so on. As with the MgF 2 chamber, these other wavelengths can be filtered out by the use of appropriate glass or other filters.
Note that the system shown in Figure 4.7B allows for continuous scanning of the absorption spectrum for any analyte. Wavelengths can be continuously changed, most often using electronic devices, by changing the position of the aperture on the exit side of the chamber or by changing the angle of incidence of the entering light by altering the angle of the near surface of the chamber. Filters that have this capacity are called monochromators . Both interference systems in Figure 4.7 have spectral bandwidths of approximately 1.5% of the wavelength at peak transmittance, much lower than for the absorption filters illustrated in Figure 4.6 .
Postsample Filters. A different approach to obtaining monochromatic light and an accurate determination of absorbance is simply to allow the light to pass through the sample unfiltered. The light is then passed through a prism, where it is broken into its constituent wavelengths. The action of a prism depends on refraction of radiation by the prism material. The dispersive power depends on the variation of the refractive index with wavelength. A ray of radiation that enters a prism at an angle of incidence is bent toward the normal (vertical to the prism face); at the prism–air interface, it is bent away from the vertical. As we describe in the section on photomultipliers that follows, each wavelength is focused onto a photodiode array such that each photodiode responds to only a specific set of wavelengths. The amount of transmitted light in the presence of the sample is compared with that in the absence of the sample. Photodiode arrays are examples of devices that convert photon energy into electrical currents in a system where photons excite the outer electrons in metal to move in the so-called conduction band, generating a current.
Sample containers (i.e., cells or cuvettes) are used to hold samples and must be made of material that is transparent to radiation in the spectral region of interest. In the ultraviolet region of the EMS (below 350 nm), cuvettes are made from fused silica or quartz. Silicate glasses can be used in the region between 350 and 2000 nm. Plastic containers have also found application in the visible region. Generally, the path length of cuvettes is 1 cm, although much smaller path lengths are used in automated systems. However, to increase sensitivity, some cuvettes are designed to have path lengths of 10 cm, increasing the absorbance for a given solution by a factor of 10 (Beer’s law; Eq. 4.8 ).
Many double-beam spectrophotometers are designed with two cuvette holders: one for the sample and the other for the solvent. If two cuvettes are used, they should be optically matched for more accurate results. Matched pairs of cuvettes are obtainable from a manufacturer, but their optical performance should be verified before use by measuring the absorbance of identical solutions and comparing their values. One cell should then be reserved for the sample solution and the other for solvent. Cells should be scrupulously cleaned before and after each use.
It is necessary to determine how much light passes through the sample in the cuvette. To accomplish this task, advantage is taken of the photoelectric effect that forms the basis of the quantum theory. Photons of specific wavelength excite the outer shell electrons of metals at their resonance frequencies to high-energy states in which they move through the so-called “conduction band” referred to above, where electrons move through the outer shells of the metal, thereby causing a current. This current, the magnitude of which is directly related to the intensity of the incident light, can then be detected and digitized.
Photomultiplier Tubes (PMTs). Perhaps the most common type of photon detector is the photomultiplier. Photomultiplier tubes are commonly used when radiant power is very low, which is characteristic of very-low-analyte concentrations. The operating principle is similar to the phototube, with one significant difference. Some PMTs are designed to provide an amplified signal nearly 1 million times larger than their phototube counterparts. This is accomplished by using multiple dynodes positioned throughout the PMT. The response of the PMT begins when incoming photons strike a photocathode. Electrons are ejected from the surface of the photocathode. A PMT has several additional electrodes called dynodes , each having a potential that is approximately 90 V higher than the previous one. Upon striking a dynode, each photoelectron causes emission of several additional electrons. These, in turn, are accelerated toward a second dynode, which is 90 V more positive than the previous dynode, which further amplifies the incident signal. This process, shown in Figure 4.8 , continues within the PMT until all electrons are collected at the anode, where the resulting current is passed to an electronic amplifier.
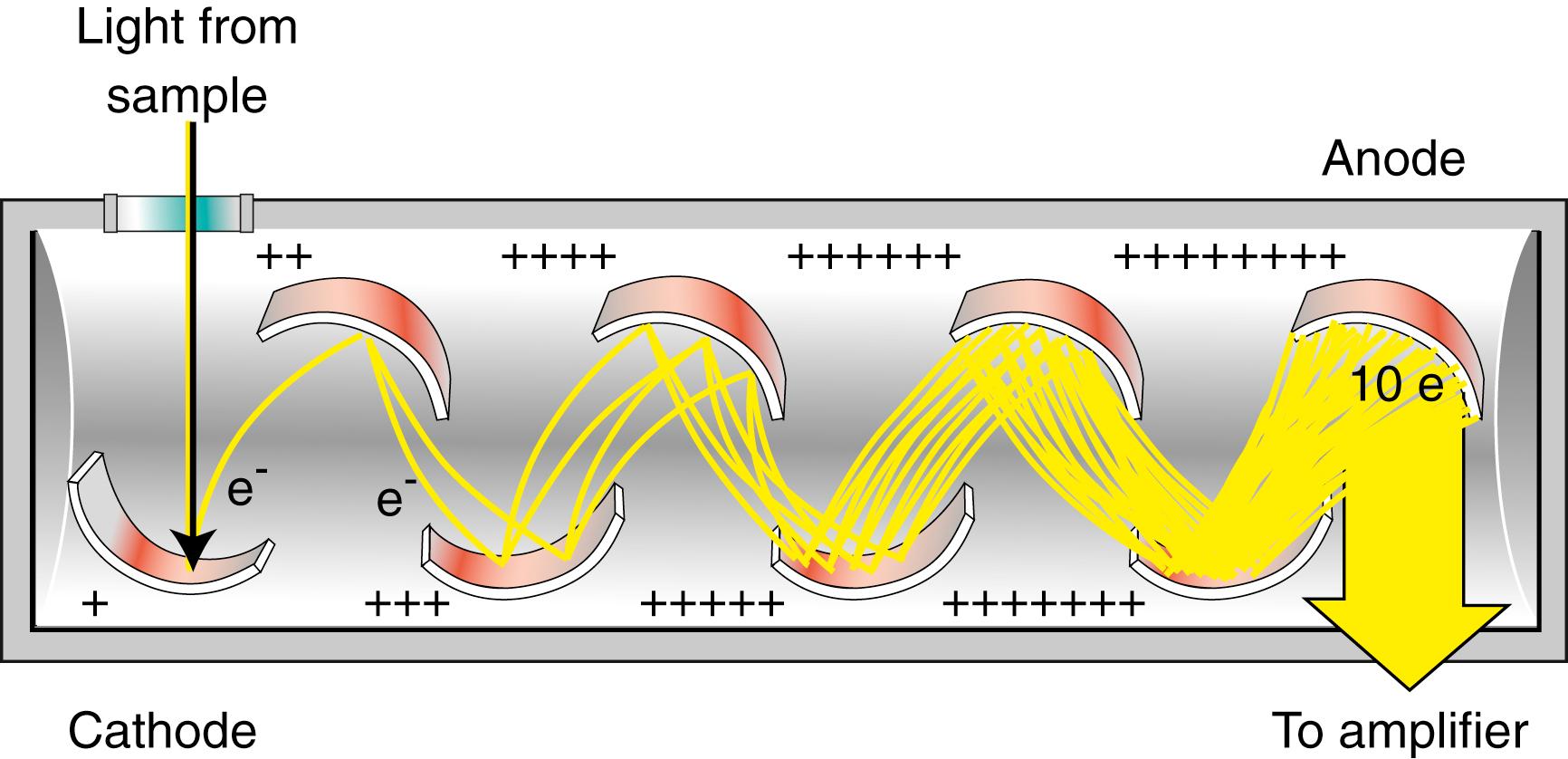
Photomultiplier tubes are highly sensitive to ultraviolet and visible radiation. They also have very fast response times. These tubes are limited to measuring low-power radiation because intense light causes irreversible damage to the photoelectric surface.
Photovoltaic or Barrier Layer Cell. A variant method of detecting photon-induced current is the photovoltaic cell. This is a basic phototransducer that is used for detecting and measuring radiation in the visible region. This cell typically has a maximum sensitivity at about 550 nm, and the response falls off to about 10% of the maximum at 350 and 750 nm. It consists of a flat copper or iron electrode on which is deposited a layer of semiconductor material, such as selenium. The outer surface of the semiconductor is coated with a thin, transparent metallic film of gold or silver, which serves as the second or collector electrode. When radiation of sufficient energy reaches the semiconductor, covalent bonds are broken, with the result that conduction electrons and holes are formed. The electrons migrate toward the metallic film and the holes toward the base upon which the semiconductor is deposited. The liberated electrons are free to migrate through the external circuit to interact with these holes. The result is an electrical current of a magnitude that is proportional to the number of photons that strike the semiconductor surface and a photocurrent that is directly proportional to the intensity of the radiation that strikes the cells.
These types of photodetectors are rugged and low cost, and they require no external source of electrical energy. Low sensitivity and fatigue are two distinct disadvantages of these cells. For routine analyses at optimum wavelengths, these photocells provide reliable analytic data.
Vacuum Phototubes. A vacuum phototube has a semicylindrical cathode and a wire anode sealed inside an evacuated transparent envelope. The concave surface of the electrode supports a layer of photoemissive material that tends to emit electrons when it is irradiated. When a potential is applied across the electrode, the emitted electrons flow to the wire anode, generating a photocurrent that is generally about one-tenth as great as the photocurrent associated with photovoltaic cells for a given radiant intensity. The number of electrons ejected from a photoemissive surface is directly proportional to the radiant power of the beam that strikes the surface. All of the electrons are collected at the anode. Several types of photoemissive surfaces are used in commercial phototubes. These include highly sensitive bialkali materials made up of potassium, cesium, and antimony; red-sensitive material using multialkalis—for example, Na/K/Cs/Sb; ultraviolet sensitive with UV transparent windows; and flat response-type substances using Ga/As compositions.
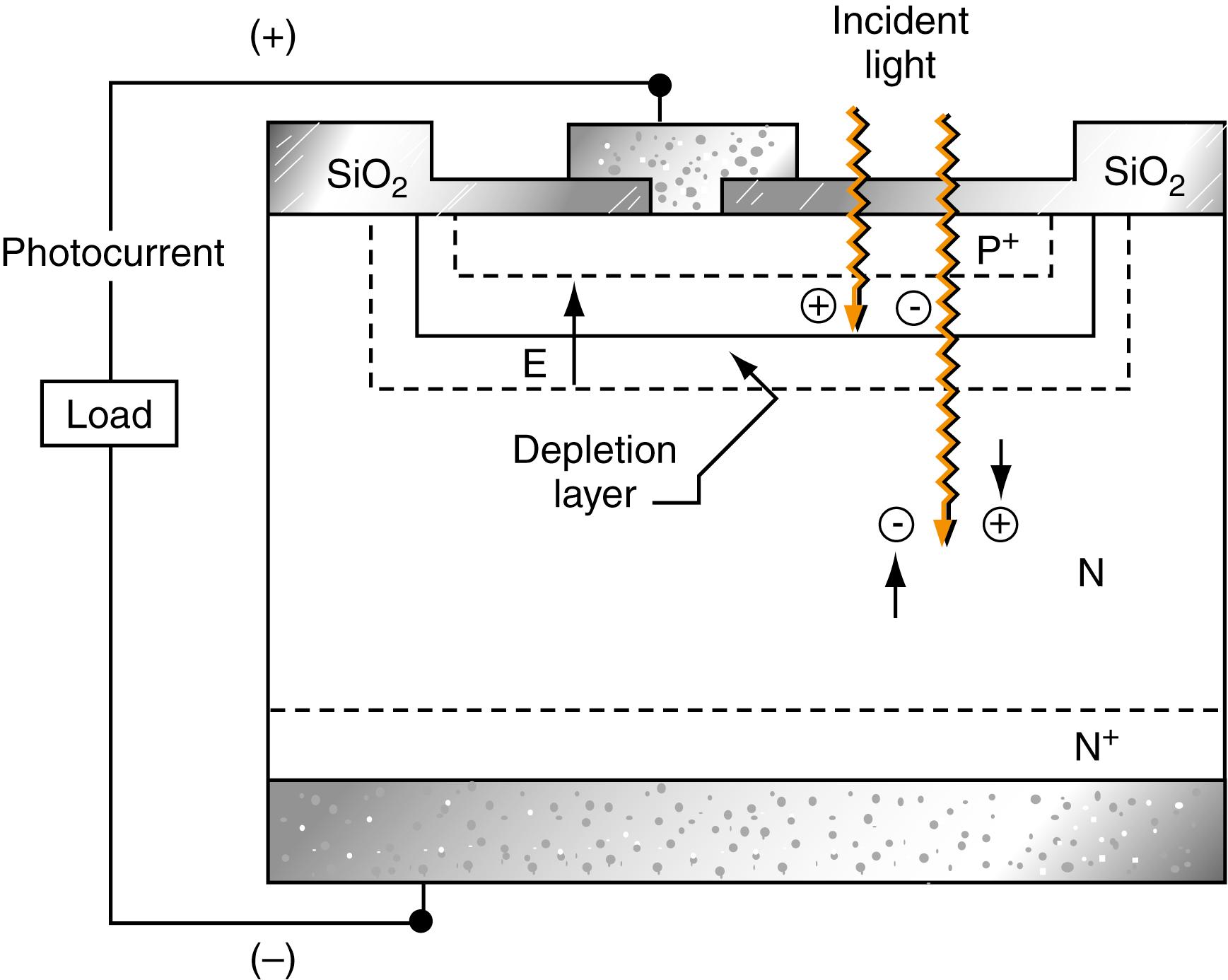
Silicon Diode Transducers. Silicon diode transducers are more sensitive than vacuum phototubes but less sensitive than the PMTs described earlier. Photodiodes have spectral ranges from about 190 to 1100 nm. These devices contain positively (P) and negatively (N) charged semiconductive materials adjoining one another, embedded on a silicon chip. A power supply is attached to this arrangement so that its positive pole connects to the N -type and its negative pole to the P -type material. This arrangement results in a depletion layer that reduces the conductance of the junction to nearly zero. If radiation is allowed to impinge on the chip, holes and electrons formed in the depletion layer are swept through the device to produce a current that is proportional to radiant power ( ). This process is shown in Figure 4.9 .
Multichannel Photon Transducers. In the discussion of postsample filters, it was noted that unfiltered light emerging from the sample could be broken up into its constituent wavelengths using prisms. These different wavelengths are then directed to electronic detectors such that the intensity of light of each of the constituent wavelengths can be quantitated. Here, we describe some of these multiwavelength photodetectors.
A multichannel transducer consists of an array of small photoelectric-sensitive elements arranged linearly or in a two-dimensional pattern on a single semiconductor chip. The chip, which is usually silicon and typically has dimensions of a few millimeters on a side, also contains electronic circuitry that makes it possible to determine the electrical output signal from each of the photosensitive elements sequentially or simultaneously. The alignment of a multichannel transducer is generally in the focal plane of a spectrometer; thus, various elements of the dispersed spectrum can be converted and measured simultaneously. Several types of multichannel transducers currently are used; they may include photodiode arrays (PDAs), charge-injection devices (CIDs), and charge-coupled devices (CCDs).
By using the modern fabrication techniques of microelectronics, it is now possible to produce a linear (one-dimensional) array of several hundred photodiodes set side-by-side on a single integrated circuit (IC), or “chip” ( Fig. 4.10 ). Each diode is capable of recording the intensity at one point along the line—together, they provide a linear profile of the light variation along the array.

A multiplex method is used to sort all of the signals received from the PDA. It records each signal individually and then feeds the signals sequentially to a single amplifier. The output of the PDA is a histogram profile, along the array, of the charge leaked by each photodiode. This mirrors the variation of light intensity across the array. Thus, the PDA detection occurs in three main stages:
Initialization
Accumulation of charge at each pixel-integration time
Read-out signals
In comparison with the PMT, the PDA has a lower dynamic range and higher noise. It is most useful as a simultaneous multichannel detector ( ).
Recent developments in solid-state detection techniques have now produced very effective two-dimensional array detectors that operate on a charge-transfer process as an alternative to photodiodes. A charge-transfer device (CTD) is a detection system in which a photon, striking the IC semiconductor material, releases electrons from their bound state into a mobile state. The released charge, consisting of negative electrons and positive holes, then drifts to, and accumulates at, surface electrodes. An array of these surface electrodes divides the detector into separate, light-sensitive “pixels.” The charge that accumulates at each electrode is proportional to the integrated light intensity falling on that particular pixel.
Two distinct classes of CTDs are known: charge-injection devices (CIDs) and charge-coupled devices (CCDs). In a CCD, all of the charge packets are moved “in-step” along the array row from one pixel to the next, as in a “bucket chain.” At the end of the row, the charge packets are fed sequentially into an on-chip low-noise amplifier, which then converts the charge into a voltage signal. The overall signal profile across the two-dimensional array is recorded one row at a time, thus giving a series of voltage signals corresponding to all of the pixels in the detection area.
In a CID, the charge accumulated in each pixel can be measured independently and nondestructively by using a network of “sensing” electrodes, which can monitor the presence of the accumulated charge. This is an important factor that differentiates CID systems from CCD and PDA systems, in which the whole of the detection area is “read” destructively in a single process.
The processing of an electrical signal received from a transducer is accomplished by a device that amplifies, rectifies AC to DC (or the reverse), alters the phase of the signal, and filters it to remove unwanted components. In addition, the signal processor may need to perform mathematical operations on the signal such as differential calculations, integration, or conversion to a logarithm. Several readout devices have been used and include digital meters, d’Arsonval meters, recorders, LEDs, cathode-ray tubes (CRTs), and liquid crystal displays (LCDs).
Several photometric parameters must be monitored periodically by users. Monitoring these parameters is recommended by most regulatory agencies and accrediting organizations. The parameters routinely monitored include wavelength or photometric accuracy, absorbance check, linearity, and stray light.
Accuracy is the closeness of a measurement to its true value. Wavelength accuracy implies that a photometer is measuring at the wavelength that it is set to. Photometric accuracy can be assessed easily using special glass-type optical filters. Two examples of commonly used filters include didymium and holmium oxide. Didymium glass has a broad absorption peak around 600 nm, and holmium oxide has multiple absorption peaks with a sharp peak occurring at 360 nm.
An absorbance check is performed using glass filters or solutions that have known absorbance values for a specific wavelength. The operator simply measures the absorbance of each solution at a specified wavelength and compares the results with the stated values. Each user should establish a tolerance for the measurements based on accepted criteria.
Linearity is defined as the ability of a photometric system to yield a linear relationship between the radiant power incident on its detector and the concentration (i.e., Beer’s law). The linearity of a spectrometer can be determined using optical filters or solutions that have known absorbance values for a given wavelength. Linearity measurements should be evaluated for both slope and intercept.
Stray light is described as any light that impinges on the detector that does not originate from a polychromatic light source. Stray light can have a significant impact on any measurement made. Stray light effect can be evaluated by using special cutoff filters.
Several instrument designs and configurations may be used for absorption photometry. Each has unique terminology associated with its design. The terminology is not universal among users but is presented here as a guide. A spectroscope is an optical instrument used for visual identification of atomic emission lines. It has a monochromator, usually a prism or diffraction grating, in which the exit slit is replaced by an eyepiece that can be moved along the focal plane. The wavelength of an emission line can then be determined from the angle between the incident and dispersed beam when the line is centered on the eyepiece.
A colorimeter uses the human eye as the detector. The user compares the observed color of the unknown sample against a standard or a series of colored standards of known concentrations. Photometers consist of a light source, a filter, and a photoelectric transducer, as well as a signal processor and readout. Some manufacturers use the term colorimeter or photoelectric colorimeter for a photometer. These photometers use filters for isolation of specific wavelengths, not gratings or prisms.
A spectrometer is an instrument that provides information about the intensity of radiation as a function of wavelength or frequency. Spectrophotometers are spectrometers equipped with one or more exit slits and photoelectron transducers that permit determination of the ratio of the power of two beams as a function of wavelength, as in absorption spectroscopy. Most spectrophotometers use a grating monochromator to break up the light into a spectrum, as discussed earlier in the section on presample filters.
Single-beam spectrophotometers are the simplest types of absorption spectrometers. These instruments are designed to make one measurement at a time at one specified wavelength. The absorption maximum of the analyte must be known in advance when a single-beam instrument is used. The wavelength is then set to this value. The reference material (solvent blank) is transferred into a suitable cuvette and is placed in the path of the monochromatic light. The spectrometer is adjusted to read 0%T when a shutter is placed to block all radiation from the detector and is adjusted to read 100%T when the shutter is removed. After these adjustments have been made, the sample is placed in the light path, the absorbance is measured, and the concentration is determined using calculations based on Beer’s law or by constructing a calibration curve for the analyte.
A double-beam spectrophotometer splits or chops the monochromatic beam of radiation into two components. One beam passes through the sample and the other passes through a reference solution or blank. In this design, the radiant power in the reference beam varies with the source energy, monochromator transmission, reference material transmission, and detector response, making the difference between the sample and the reference beam largely a function of the sample. The output of the reference beam can be kept constant, and the absorbance of the sample can be recorded directly as the electrical output of the sample beam.
Two fundamental instrument designs are used for double-beam spectrophotometers: double beam in space and double beam in time. A double beam in space design uses two photodetectors: one for the sample beam and the other for the reference beam. The two signals generated are directed to a differential amplifier, which then passes on the difference between the signals to the readout device. A schematic of the double beam in space system is shown in Figure 4.11 .
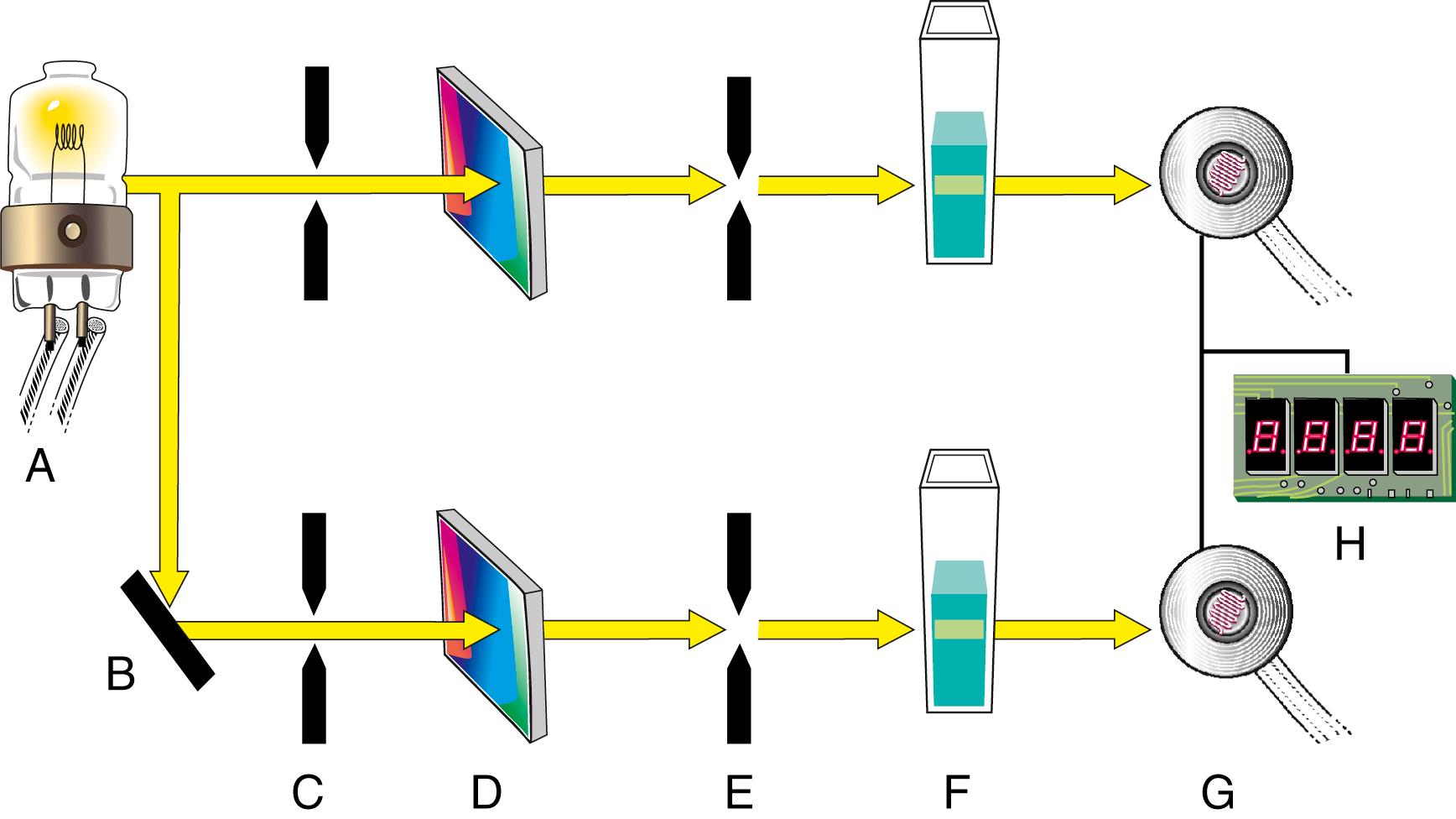
A double beam in time instrument uses one photodetector and alternately passes the monochromatic radiation through the sample cuvette and then to the reference cuvette, using a chopper . A chopper is the term used for a device such as a rotating sector mirror that breaks up or rotates radiation beams. Each beam, consisting of a pulse of radiation separated in time by a dark interval, is then directed onto an appropriate detector. A schematic of the double beam in time system is shown in Figure 4.12 .
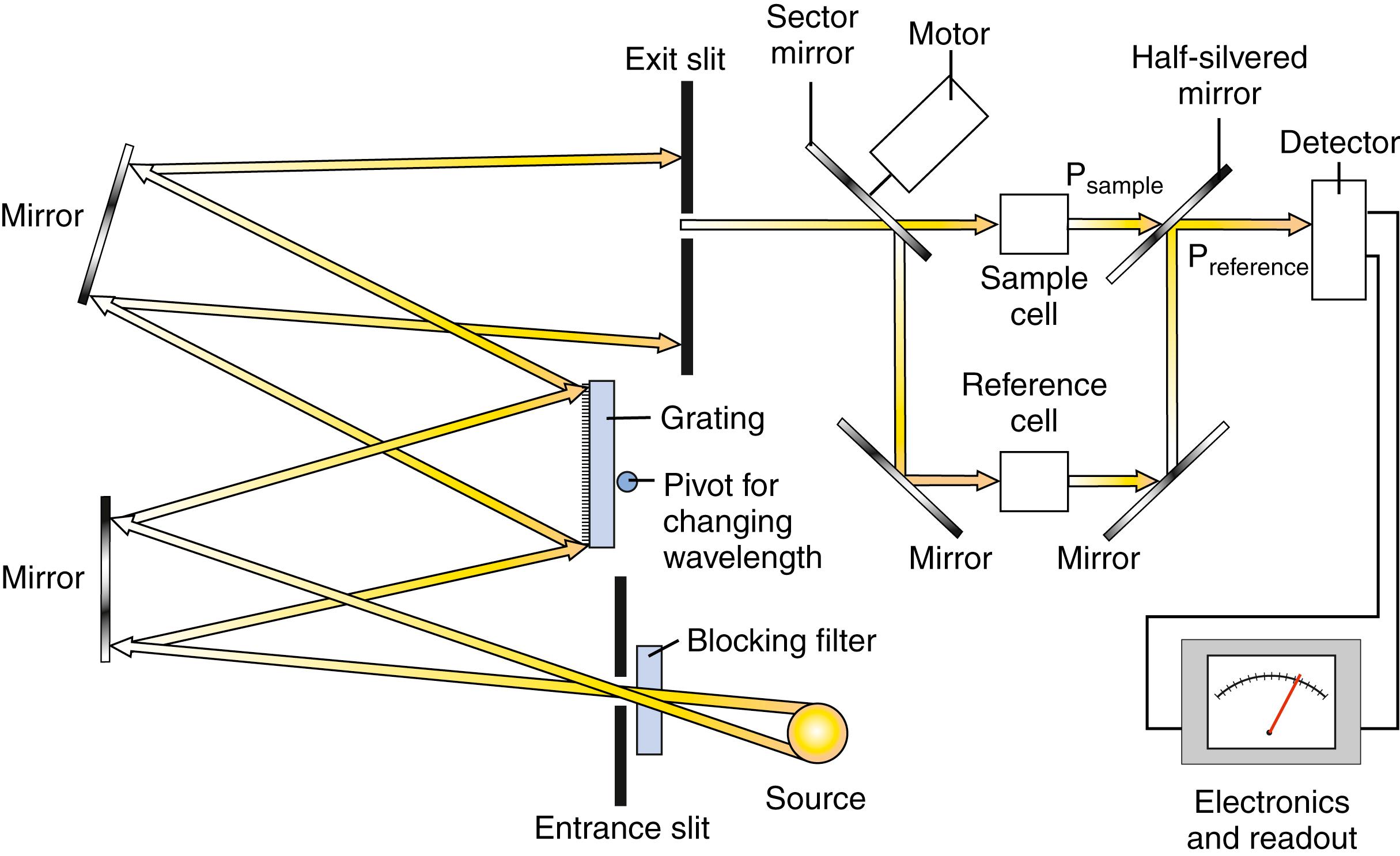
A scanning double-beam system includes a double-beam spectrophotometer and a recorder that can provide an X-Y plot of absorbance versus wavelength for a given test sample. This type of configuration is ideal for determining the wavelength spectrum of an analyte in solution. The spectrophotometer has an automatically driven wavelength cam that can rotate at a predetermined speed. The recorder can be calibrated to the wavelength of the monochromator to facilitate the identification of each peak maximum.
Measurement of analytes in biologic fluids using reflectometry has been used for decades. Two clinical applications include urine dipstick analysis and dry slide chemical analysis. The instruments used for these applications include a reflectometer. A reflectometer is a filter photometer that measures the quantity of light reflected by a liquid sample that has been dispensed onto a grainy or fibrous solid support.
The two types of reflectance are specular and diffuse. Specular reflectance occurs on a polished surface where the angle of incidence of the radiant energy is equal to the angle of reflection. Polished surfaces—for example, a mirror—can be used to direct and manage radiant energy but not to determine concentration. Diffuse reflectance occurs on nonpolished surfaces (i.e., a grainy or fibrous surface), as noted earlier. The reflected radiant energy tends to go in many directions. Diffuse reflection occurs within the layers and depends on the properties and characteristics of the layers themselves. A colored substance absorbs the wavelength of its color and reflects all other wavelengths at many different angles. Therefore, the amount of a substance present can be measured as an indirect function of the reflected light.
A typical reflectometer used in a clinical laboratory detects only a constant fraction of the diffuse reflected light. Thus, the reflectance of a sample is represented by the following:
The amount of light reflected by a solution dispensed onto a white granular or grainy surface is inversely related to the concentration of the sample, as given by the following:
where R density = corrected reflectance density of the sample, R sample = measured reflectance of the sample, R black = reflectance of a black reference, R white = reflectance of a white reference, and R standard = reflectance of a standard solution.
The ideal reflectance value of a pure white standard of ceramic material is one (i.e., all light is reflected). Conversely, the ideal reflectance value of pure black material is zero (i.e., all light is absorbed).
The relationship of percent reflectance and concentration of an analyte is nonlinear. Several algorithms have been developed for linearizing this relationship. The specific algorithm used depends on the reflection characteristics of the composition material of the pad or film, the nature of the illumination, and the geometry of the instrument.
The components of a reflectometer are very similar to those of a photometer, as shown in Figure 4.13 . A tungsten–quartz halide lamp serves as a source of polychromatic radiation. A monochromator (e.g., stationary filter or filter wheel for multiple analytes) is used to isolate the wavelength of interest. Next, the monochromatic light passes through a slit and is directed onto the surface of a urine dipstick pad or dry slide, depending on the instrumentation used. Solid-state photodiodes are typically used to detect reflected radiant energy. Special optical devices—for example, fiberoptics or ellipsoidal mirrors—can be used to direct radiant energy onto the detector. A computer or microprocessor is used to convert nonlinear reflectance signals into direct readout concentration units.
![Figure 4.13, Components in a typical reflectometer used to measure analytes on urine dipstick. The different-colored blocks represent different tests performed in urinalysis. Blue represents the quantitation of analyte using reflectance (i.e., the reflectance of light focused on the sample and reflected at an angle [yellow beam] , where it is detected). Figure 4.13, Components in a typical reflectometer used to measure analytes on urine dipstick. The different-colored blocks represent different tests performed in urinalysis. Blue represents the quantitation of analyte using reflectance (i.e., the reflectance of light focused on the sample and reflected at an angle [yellow beam] , where it is detected).](https://storage.googleapis.com/dl.dentistrykey.com/clinical/AnalysisPrinciplesOfInstrumentation/12_3s20B9780323673204000043.jpg)
Luminescence is based on an energy exchange process that occurs when certain compounds absorb electromagnetic radiation, become excited, and return to an energy level lower than or equal to their original level. Because some energy is lost before emission from the excited state by collision with the solvent or other molecules, the wavelength of the emitted light is longer than that of the exciting light. Most uncharged molecules contain even numbers of electrons in the ground state. These electrons fill molecular orbits in pairs with their spins in opposite directions. No electron energies can be detected by application of a magnetic field to such spin patterns; this electronic state is called the singlet state . Similarly, if an electron becomes excited by electromagnetic radiation and its spin remains paired with the ground state, this creates a singlet excited state. The lifetime of the excited state is the average length of time the molecule remains excited before emission of light. For a singlet excited state, the lifetime of the excited state is on the order of 10 −9 to 10 −6 s. The light emission from a singlet excited state is called fluorescence . When the spins of the electrons in the excited state are unpaired, the electron energy levels will be split if a magnetic field is applied; this electronic state is called a triplet state . Triplet state lifetimes range from 10 −4 to 10 s ( ). Light emission from an excited triplet state is called phosphorescence .
Luminescence is widely used because of its high sensitivity; the signal-to-noise ratio is very high because light is measured against a nearly dark background. High specificity is a function of using two spectra, the excitation and emission spectra, and the possibility of measuring the lifetime of the fluorescent state. Two compounds that are excited at the same wavelength but emit at different wavelengths are readily differentiated with this technique.
Instruments designed for fluorescence measurement have the following basic components: a light source, an excitation (primary) monochromator, a cuvette, an emission (secondary) monochromator, and a photodetector ( Fig. 4.14 ). The exciter lamp is a high-intensity light source, such as a mercury vapor lamp or a xenon arc lamp. Simple instruments use mercury vapor lamps that do not require any special power supply. Mercury vapor lamps produce discrete and intense resonance lines that are not ideal for compounds with absorption bands at wavelengths not coinciding with these emission bands. For such compounds, xenon arc lamps producing an intense continuous spectrum between 300 and 1300 nm are appropriate. These lamps are used in nearly all commercial spectrophotofluorometers. In fluorescence measurements, the emitted light is detected at a right angle to the incident light to eliminate potential interference by the excitation signal. Phototubes or PMTs are required for fluorescence measurements because the signals are generally of low intensity. Newer fluorometers on the market today use diode array and CTDs, which allow the rapid recording of both excitation and emission spectra and are particularly useful in chromatography and electrophoresis.
Time-resolved fluorescence assays minimize problems inherent in other fluorescent assays, such as overlapping excitation or emission spectra of compounds present in the sample with the fluorophore. The label most commonly used is a chelate of europium (Eu 3+ ). Energy is absorbed by the organic ligand, leading to an excited state as the electrons migrate from the ground state singlet to the excited singlet state. The excitation may lead to any vibrational multiplet of the excited state S 1 . The molecule rapidly returns to the lowest energy levels in S 1 by a nonradiative process. The energy is transferred to the metal ion, which becomes excited and subsequently emits characteristic radiation. The radiative transition of excited Eu 3+ after an energy transfer from triplet state results in an emission wavelength of 613 nm. The fluorescence lifetime of Eu 3+ chelate is 10 to 1000 μs compared with nanoseconds for the most commonly used fluorophore. Therefore, Eu 3+ with its longer emission lifetime makes it more attractive to use over a fluorophore-like fluorescein with a lifetime of 4.5 ns. Time-resolved fluorescence instruments are similar to a typical fluorometer except that time-resolved fluorometers use a time-gated measure on only a portion of the total emission spectra.
Chemiluminescence applications have increased dramatically owing to the increased sensitivity over fluorescence. In chemiluminescence, the light signal is measured against a completely dark background because no excitation light is required. The primary application has been in the area of immunoassays where several chemiluminescence compounds have been used as antigen labels. Chemiluminescence differs from fluorescence and phosphorescence in that the emission of light is created from a chemical or electrochemical reaction and not from absorption of electromagnetic energy. The chemical reaction yields an electronically excited compound that emits light as it returns to its ground state or that transfers its energy to another compound, which then produces emission. Chemiluminescence involves the oxidation of an organic compound (e.g., dioxetane, luminol, acridinium ester) by an oxidant (hydrogen peroxide, hypochlorite, or oxygen). These oxidation reactions may occur in the presence of catalysts, such as enzymes that include alkaline phosphatase, horseradish peroxidase, and microperoxidase, metal ions (Cu 2+ or Fe 3+ phthalocyanine complex), and hemin. The excited products formed in the oxidation reaction produce chemiluminescence on return to the singlet state. A luminometer is used to detect chemiluminescence; it contains a PMT that provides a very strong electrical output signal. A typical signal from a chemiluminescent compound rises rapidly with time and reaches a maximum when reagent and analyte are completely mixed. An exponential decay of the signal follows until baseline is reached.
Nephelometry and turbidimetry are used to measure the concentrations of large particles such as antigen–antibody complexes, prealbumin, and other serum proteins. Nephelometry detects light that is scattered at various angles; scattered light yields a small signal that must be amplified. In contrast, turbidimetry measures a reduction in light transmission due to particle formation; thus, it detects a small decrease in a large signal ( Fig. 4.15 ).
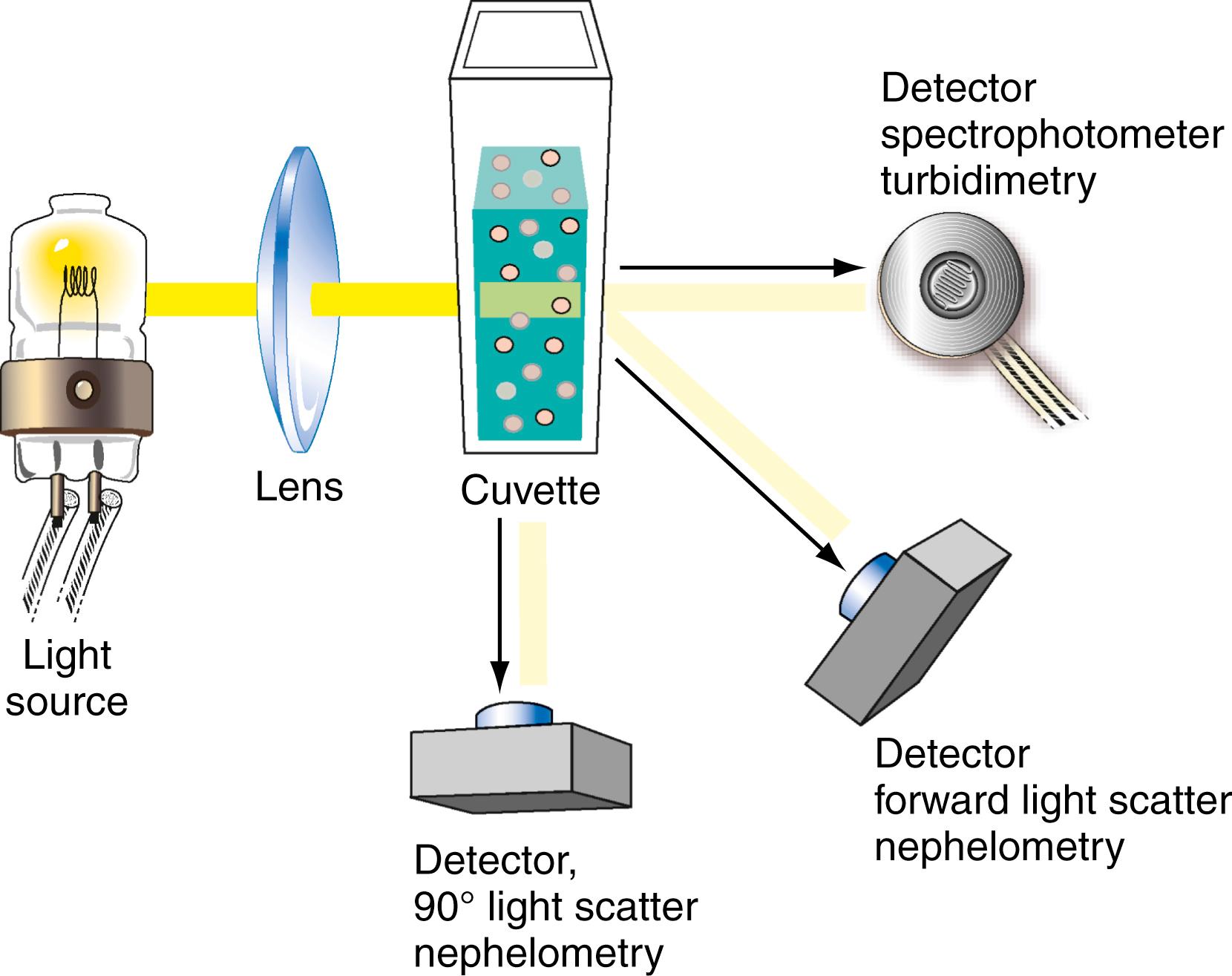
Nephelometry and turbidimetry are based on the scattering of radiation by particles in suspension. When a collimated light beam strikes a particle in suspension, portions of the light are absorbed, reflected, scattered, and transmitted. Nephelometry is the measurement of the light scattered by a particulate solution. Three types of light scatters occur according to the relative size of the light wavelength ( ). If the wavelength (λ) of light is much larger than the diameter ( d ) of the particle ( d < 0.1λ), the light scatter is symmetrical around the particle. Minimum light scatter occurs at 90 degrees to the incident beam and was described by Rayleigh ( ). If the wavelength of light is much smaller than the particle diameter ( d < 0.1λ), then the light scatters forward owing to destructive out-of-phase backscatter, as described by the Mie theory. If the wavelength of light is approximately the same as the particle size, more light scatters in the forward direction than in other directions, as defined by the Rayleigh-Debye theory. A common application of nephelometry is the measurement of antigen–antibody reactions. Because most antigen–antibody complexes have a diameter of 250 to 1500 nm and the wavelengths used are 320 to 650 nm, light is scattered forward (Rayleigh-Debye type).
A typical nephelometer consists of a light source, a collimator, a monochromator, a sample cuvette, a stray light trap, and a photodetector. Light scattered by particles is measured at an angle, typically 15 to 90 degrees to the beam incident on the cuvette (see Fig. 4.15 ). Light scattering depends on the wavelength of incident light and particle size. For macromolecules with size close to or larger than the light wavelength, measurement of forward light scatter increases the sensitivity of nephelometry. Light sources include a mercury-arc lamp, a tungsten-filament lamp, a light-emitting diode, and a laser.
Lasers produce stable, nearly ideal monochromatic light of narrow bandwidth and emit radiant energy that is coherent, parallel, and polarized. A laser beam can be maintained as a very slim cylinder only a few micrometers in cross-section. A typical helium-neon laser lamp consists of a helium-pumping electrode (cathode) and a hollow glass laser core surrounded by a laser plasma tube (anode). Both the plasma tube and the core are filled with free helium and neon gases. The electrical discharge between the cathode and the anode is confined to the hollow glass core to keep it concentrated for maximum energy transfer. Two mirrors are positioned at the ends of the laser tube. One of them is fully reflective and the other partially transparent. When the electrode is charged, the helium atoms are excited to a higher energy state and then transfer this energy to the neon atoms by collision. In turn, the excited neon atoms emit photons. Photons bounce back and forth between the two end mirrors, stimulating other atoms to emit additional photons, resulting in an amplification process. The amplified light eventually emerges as a laser beam through the partially transparent mirror. With the high-intensity monochromatic beam, a substantial increase in sensitivity has been seen with lasers over conventional light sources. Disadvantages of laser sources include cost, safety and cooling requirements, and limited availability of wavelengths.
The measurement of the reduction in light transmission caused by particle formation is termed turbidimetry . Light transmitted in the forward direction is detected. The amount of light absorbed by a suspension of particles depends on the specimen concentration and on the particle size. Solutions requiring quantitation by turbidimetry are measured using visible photometers or visible spectrophotometers. Higher sensitivity has been achieved using photodetectors that can detect small changes in photon signals. Sensitivity comparable with nephelometry can be attained using low wavelengths and high-quality spectrophotometers. Many clinical applications exist for turbidimetry. Various microbiology analyzers measure the turbidity of samples to detect bacterial growth in broth cultures. Turbidimetry is routinely used to measure the antibiotic sensitivities from such cultures. In coagulation analyzers, turbidimetric measurements detect clot formation in the sample cuvettes. Turbidimetric assays have long been available in clinical chemistry to quantify protein concentration in biologic fluids, such as urine and cerebrospinal fluid (CSF).
Scattered light can further be used to determine the size and shape of cells or macromolecules in a suspension using the technique of flow cytometry. The principles of flow cytometry are discussed at length in Chapter 46. Here, we present a brief description of flow cytometry to illustrate how scattered light can be used to image cells.
A flow cytometer measures multiple properties of cells suspended in a moving fluid medium. As each particle passes single file through a laser light source, it produces a characteristic light pattern that is measured by multiple detectors for scattered light (forward and 90 degrees) and fluorescent light (if the cell is stained with a fluorochrome). Flow cytometry is used to count and sort cells as well as viral particles, DNA fragments, bacteria, and latex beads. It is a core component of hematology cell counters and the technology used to differentiate white blood cells.
In flow cytometry, the term particle describes any object flowing through the instrument. An event is anything that is interpreted by the instrument to be a single particle. An event may be determined correctly or incorrectly by a flow cytometer. Methods have been developed to compensate for measurement of unwanted events. An example is the correction for measuring the simultaneous passing of two particles. Particles must be in suspension as single cells to be analyzed. If not, they can be made suitable for flow cytometry by the use of mechanical disruption or enzymatic digestion. Size restrictions also apply; cells or particles must be from 1 to 30 μm in diameter. Specialized flow cytometers are designed to handle smaller particles such as DNA fragments or bacteria.
The system shown in Figure 4.16 has all of the design features of a flow cytometer with cell-sorting capabilities. The cell suspension aliquots are introduced into the flow chamber using air pressure. As cells pass through the flow chamber, a low-pressure sheath fluid surrounds them. This outer fluid stream creates a laminar flow that forces the specimen to the center and results in a single-file alignment of the individual cells. This process is called hydrodynamic focusing . A laser beam passes through each cell as it flows through the chamber. Forward light scatter is proportional to cell size, and 90-degree or right-angle scatter is related to cell granularity and nuclear irregularity. If the cells are labeled with appropriate fluorochromes, fluorescent signals proportional to the amount of bound label can be measured. Green fluorescence usually means that the dye fluorescein was used as a marker; red fluorescence usually means that a dye such as phycoerythrin was used as the contrasting marker. These dyes are usually attached to antibodies to specifically target selective antigens on cells or particles.
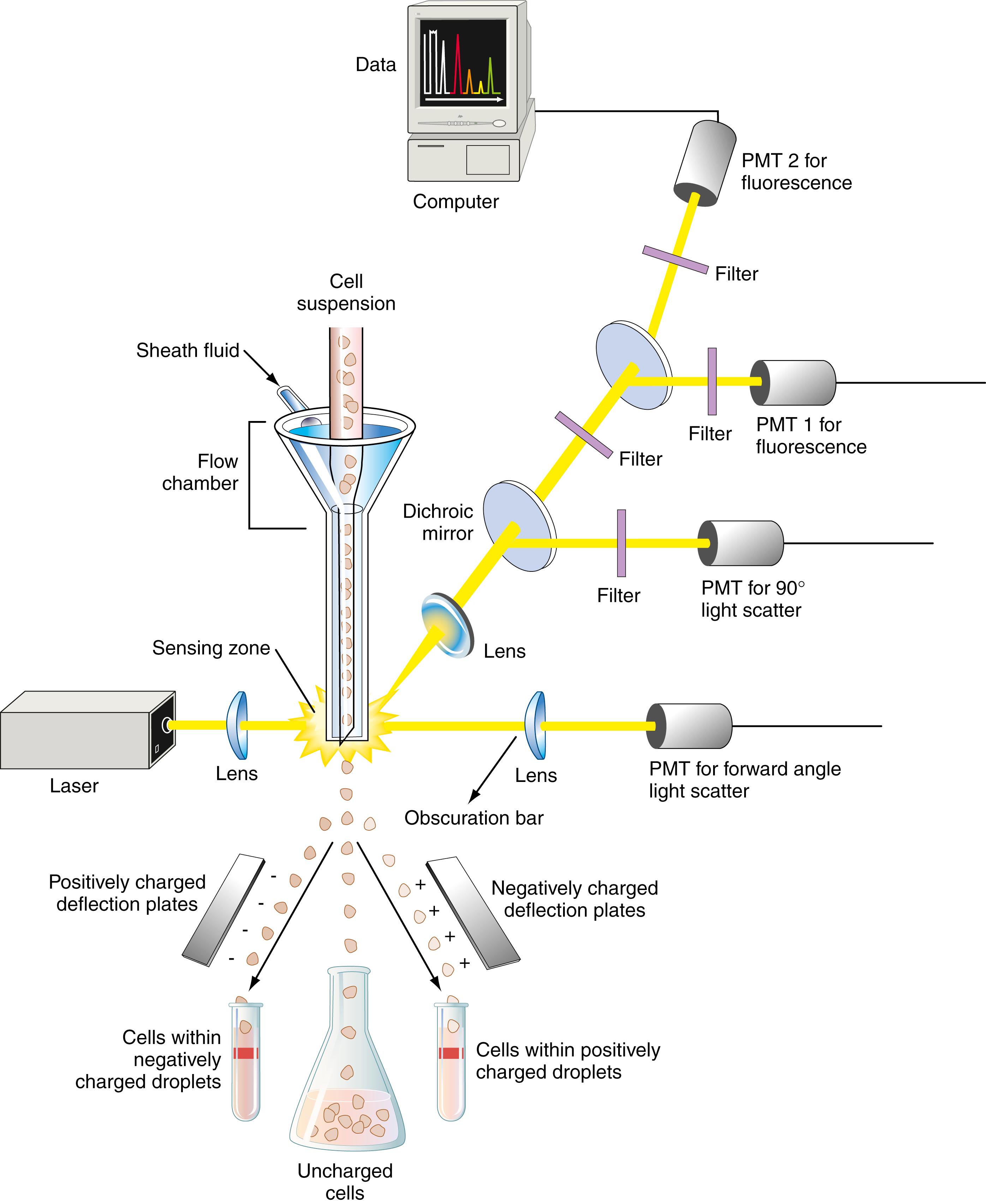
Forward light scatter is directed to the forward scatter photodetector. At right angles to the laser beam are mirrors that divide the right-angle light scatter among the remaining photodetectors (e.g., a right-angle scatter detector and two fluorescence detectors). Across the forward lens is an obscuration bar that blocks the laser beam after it passes through the stream. Only light from the laser that has been refracted or scattered as it strikes a particle in the stream is diverted enough from its original direction to avoid the obscuration bar and strike the forward positioned lens and the photodiode behind it. Granulocytes, monocytes, and lymphocytes are separated on the basis of size and granularity patterns as determined by simultaneously analyzing forward and right-angle light scatter. For example, granulocytes with irregular nuclei scatter more light to the side than do lymphocytes with their spherical nuclei. Cell subpopulations can be identified by using electronic gating and analyzing fluorescence patterns (based on labels used for specific cells).
Fluorescence-activated cell sorter (FACS) describes the ability of a flow cytometer to physically sort cells in a liquid suspension. To do so, the instrument design has to be modified to electrically charge cells of interest. This is done by first vibrating the sheath stream to break it into drops. The stream of drops flows past two charge (high-voltage) plates, where the cells of interest are electrically charged with a voltage pulse. Then the flow stream enters an electrical field, where the charged cells are deflected into suitable collection containers. Unwanted cells are not charged and are not deflected upon passing through the field.
Refractometry is based on light refraction. When light passes from one medium into another, the light beam changes its direction at the boundary surface if its speed in the second medium is different from that in the first. The ability of a substance to bend light is called refractivity , and it is measured as a difference between the angle of incidence and the angle of refraction . The refractivity of a liquid depends on the wavelength of the incident light, the temperature, the nature of the liquid medium, and the concentration of the solute dissolved in the medium. If the first three factors are held constant, the refractivity of a solution is an indirect measurement of total solute concentration. Refractometry can be used to measure protein concentration, specific gravity of urine, and concentration of solutes in column effluent of high-performance liquid chromatography analysis.
Osmometry is the measurement of the osmolality of an aqueous solution such as serum, plasma, or urine. As osmotically active particles (e.g., glucose, urea nitrogen, sodium) are added to a solution, causing its osmolality to increase, four other properties of the solution are also affected. These properties are osmotic pressure, boiling point, freezing point, and vapor pressure. They are called colligative properties of the solution because they can be related to one another and to the osmolality. As the osmolality of a solution increases, (1) the osmotic pressure increases, (2) the boiling point is elevated, (3) the freezing point is depressed, and (4) the vapor pressure is depressed. Osmometry is based on measuring changes in the colligative properties of solutions that occur owing to variations in particle concentration. Freezing-point depression osmometry is the most commonly used method for measuring the changes in colligative properties of a solution. It is based on the principle that the addition of solute molecules lowers the temperature at which a solution freezes.
Become a Clinical Tree membership for Full access and enjoy Unlimited articles
If you are a member. Log in here