Total Intravenous Anesthesia and Target-Controlled Infusion

THE MOST COMMON INDICATIONS for total intravenous anesthesia (TIVA) techniques in children are as follows: those at risk for malignant hyperthermia (in whom inhalational agents are contraindicated); children with a high risk of postoperative nausea and vomiting, brief radiologic or painful procedures when rapid recovery is needed (e.g., magnetic resonance imaging, bone marrow aspiration, gastrointestinal endoscopy); frequent repeated anesthesia (e.g., radiation therapy); major surgery to control the stress response; neurosurgical procedures to assist with control of intracranial pressure and for cerebral metabolic protection; spinal instrumentation requiring evoked motor and auditory brain potentials; and children in need of airway procedures (e.g., bronchoscopy). Total intravenous (IV) sedation and anesthesia are used in pediatric intensive care, but propofol is contraindicated for prolonged use in very sick or young children because of the risk of developing propofol infusion syndrome (PRIS).

Pharmacokinetic and Pharmacodynamic Principles

The goal of pharmacologic treatment is a desired response, known as the target effect. An understanding of the concentration-response relationship (i.e., pharmacodynamics [PD]) can be used to predict the target concentration required to achieve this target effect in a typical child. Pharmacokinetic (PK) knowledge (e.g., clearance [CL], volume [V]) then determines the dose that will achieve the target concentration. Calculation of the dose for a drug where the PK disposition can be described using a one-compartment model with first-order elimination can be readily calculated:

Loading dose = V \times Target Concentration

$Loading dose = V \times Target Concentration$

Maintenance Dose = C L \times Target Concentration

$Maintenance Dose = C L \times Target Concentration$

However, most drugs used in anesthesia require two or more compartments to describe their disposition and although the principles for drug dosing calculation are the same, calculations are complicated by two or more clearances and volumes (see below).

Although the parameters used in PK and PD equations (known as models) for many drugs are published, the values may vary substantively from child to child. Several covariates have been identified to more accurately predict the dose for a particular child, including weight, age, sex, pathology, drug interactions, and pharmacogenomics. Current pumps used for TIVA incorporate weight and age to predict the typical drug dose and infusion rate for a specific patient.

The use of TIVA for propofol is a good example of “pharmacology in action.” The target effect (level of general anesthesia) for propofol has been defined (e.g., bispectral index [BIS] 50–55), the target (plasma) concentration to achieve this level of anesthesia is known (e.g., propofol 4 mg/L) and its PK in children is well described. Advanced concepts in PK modeling and computer technology have led to sophisticated delivery systems that facilitate anesthesia given by the IV route. Further advances involving feedback from receptor organs have also been developed for children. Target-controlled infusion (TCI) devices or “smart pumps” are an example of a sophisticated delivery system that may be directed at either plasma (Cp) or effect-site (Ce) drug concentration. These computerized pumps are a considerable advance over earlier manual techniques for children that targeted only the Cp of the drug. However, they require input of both PK and PD parameters and a lack of robust PK-PD estimates and variability in the parameter estimates limit the current accuracy of TCI in children under 3 years of age.

Pediatric Pharmacokinetic Parameter Sets

TCI techniques use propofol and remifentanil as the principal drugs for induction and maintenance of anesthesia. Popular pediatric programs used for propofol infusion targeting a plasma concentration are based on data from Marsh and Gepts, Kataria, Short, Rigby-Jones, Schuttler, Murat, Saint-Maurice, Coppens or Absalom (Paedfusor). These parameter sets are commonly termed “models” and named after the author who reported them (e.g., the Kataria model). Parameter estimates (e.g., CL; intercompartment clearance, Q; central volume of distribution, V1; peripheral volume of distribution, V2) are different for each parameter set ( Table 8.1 ). Although parameter estimates are different for each author, most predict similar concentrations for the same infusion regimen ( Fig. 8.1 ).

TABLE 8.1

Propofol Parameter Estimates for a 20-kg Child

Parameter	Kataria et al.	Marsh & Gepts	Paedfusor	Short et al.	Schuttler & Ihmsen	Rigby-Jones et al.	Murat et al.	Saint Maurice et al.	Coppens et al.
V1 (L)	10.4	4.56	9.16	8.64	7.68	11.68	20.6	14.44	3.48
V2 (L)	20.2	9.28	18.98	10.8	20.74	26.68	19.4	35.6	4.68
V3 (L)	164	58.04	116.58	69.4	264.82	223.86	121.74	168	19.02
CL (L/minute)	0.68	0.542	0.568	0.836	0.56	0.444	0.98	0.62	0.78
Q2 (L/minute)	1.16	0.51	1.044	1.22	1.036	0.32	1.34	1.24	2.04
Q3 (L/minute)	0.52	0.192	0.384	0.34	0.46	0.268	0.4	0.22	0.66

Performance of these models differed markedly during the different stages of propofol administration. Most models underestimated propofol concentration 1 minute after the bolus dose, suggesting an overestimation of the initial volume of distribution. Not all models tested were within the accepted limits of performance (median performance error, bias <20% and median absolute performance error, precision <30%). The model derived by Short and colleagues performed best in children 3 to 26 months.

FIGURE 8.1, Simulated time-concentration profiles for propofol using differing parameter sets are shown. A 3-mg/kg bolus was administered and the infusions were administered as for an adult (10-8-6 regimen, see text).

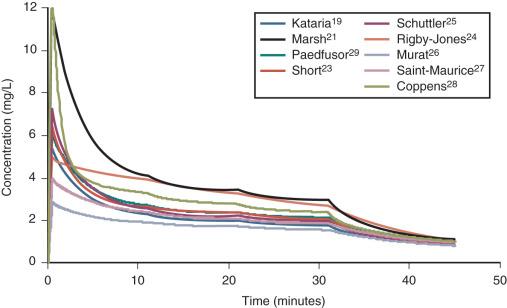
Covariate influences that contribute variability to the parameters, such as the severity of illness are often unaccounted for—for example, the volume of the central compartment is increased in children after cardiac surgery. Even weight or age, the most common sources of variability, may be omitted from parameter estimates. Both the administration method (IV bolus or infusion) and the collection of venous blood for assay rather than arterial blood will influence the PK parameter estimates in the early phase when the drug is moving into the effect-site compartment. Time-concentration profiles and context-sensitive half-lives will differ depending on which parameter set is used.

There is a paucity of validation studies for these differing parameter sets. The Paedfusor model is reported to have a median performance error, bias (MDPE) of 4.1% and a median absolute performance error, precision (MDAPE) of 9.7% in children between 1 and 15 years of age. A more recent study concluded that all parameter sets except that based on the Marsh model performed acceptably in children between 3 and 26 months. Others have described a poor fit for the Kataria model, despite the fact that it is the most widely used model. However, clearance (expressed as liters per hour per kilogram) decreases with age and MDPE is minimized at low CL and exaggerated at greater values. Evaluating models outside the age range in which their parameter sets were determined will increase the bias and worsen the precision of the model.

Adult remifentanil PK parameters continue to be used in TCI devices for patients of all ages, despite an increasing knowledge regarding the pharmacology of this drug in children. There is an element of safety with this approach because both volume of distribution and clearance (expressed as milliliters per minute per kilogram) decrease with increasing age and the elimination half-life is small with a constant context-sensitive half-life. The greater volume of distribution in children reduces the peak concentrations of remifentanil after bolus dosing; the increased clearance in children results in a smaller plasma concentration when infused at adult rates expressed as mg/minute per kilograms. Remifentanil PK can be described in all age groups by simple application of an allometric size model (see Chapter 7 ). This standardized clearance of 2970 mL/minute per 70 kg is similar to that reported by others in children and adults. The smaller the child, the greater the clearance when expressed as milliliters per minute per kilogram. Owing to these enhanced clearance rates, smaller (younger) children will require larger infusion rates of remifentanil than larger (older) children and adults to achieve equivalent blood concentrations.

The Target Concentration

The target concentration is the concentration desired at the effect site. The plasma and effect-site concentrations are the same at steady state. The target concentration depends on the desired effect. This effect is determined by an understanding of the concentration-response relationship of the drug. This will differ with age, pathology, drug interactions, and stimulus. The target concentration may vary depending on the magnitude of the desired effect. A remifentanil target of 2 to 3 µg/L is adequate for laryngoscopy, 6 to 8 µg/L for laparotomy and 10 to 12 µg/L might be sought to ablate the stress response associated with cardiac surgery.

A propofol concentration of 2 to 3 mg/L is an appropriate target for sedation and 4 to 6 mg/L is adequate for anesthesia. The target effect-site propofol concentrations for both the loss and return of consciousness in children, 2.0 ± 0.9 mg/L and 1.8 ± 0.7 mg/L, respectively, (mean ± standard deviation) are similar to those reported in adults. The relation between drug concentration and effect is commonly described by the Hill equation (see Eq. 7.19 ) :

Effect = E 0 - \frac{Emax \cdot {Ce}^{N}}{(E C_{50}^{N} + C e^{N})}

$Effect = E 0 - \frac{Emax \cdot {Ce}^{N}}{(E C_{50}^{N} + C e^{N})}$

where E 0 is the baseline level of consciousness (e.g., BIS = 100), Emax is the maximum response, EC ₅₀ the concentration at half this maximum response, Ce the concentration in the effect compartment, and N defines the steepness of the slope.

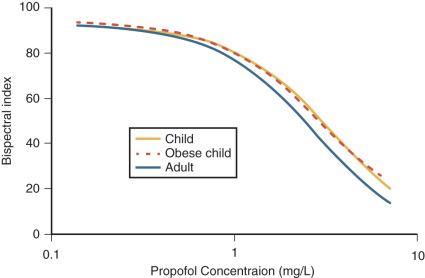
Jeleazcov et al. have described propofol PD in children 1 to 16 years using BIS where E 0 was estimated as 93.2, E max 83.4, EC ₅₀ 5.2 mg/L, and N 1.4. This relationship is very similar to that described in obese children. The equilibration rate constant ( keo ) between the plasma and effect compartment was 0.6/minute ( T _1/2 keo 1.15 minutes). Children may have a slightly lower sensitivity to propofol than adults ( Fig. 8.2 ), although this difference may be due to PK rather than PD factors. The Kataria parameter set is known to underpredict concentration as age increases, consistent with allometric scaling. When this parameter set is used to estimate PD parameters, older children appear to require a smaller concentration to maintain anesthesia ; this is a PK effect and not a PD effect.

FIGURE 8.2, The propofol concentration and its relationship with bispectral index in children and adults.

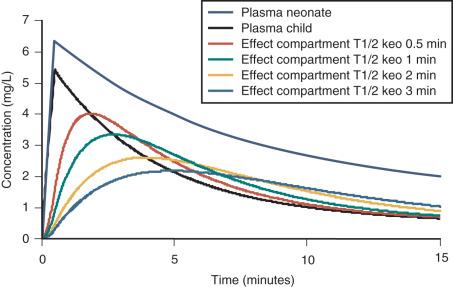
Maintenance infusion requirements for propofol in neonates differ substantively from those in older infants and children. These may be attributed to differences in PK and/or PD. In terms of the kinetics, the clearance of propofol in neonates is reduced compared with older infants; clearance decreases with decreasing postmenstrual age owing to immature enzyme clearance systems. To develop a dosage scheme for propofol infusion rates in infants and children, the adult dosage scheme was adapted to the requirements in the younger population by observing the total number and time of administration of boluses and time to awakening from propofol in infants younger than 3 years ( n = 2271) ( Table 8.2 ). The predicted infusion rates for the first 10 minutes in neonates are large (24 mg/kg per hour; 400 µg/kg per minute), values that should be used cautiously because of the danger of an overdose if the infusion continues beyond 10 minutes. Delayed awakening, hypotension, and an increased incidence of bradycardia have been reported in neonates and infants. Propofol can cause profound hypotension in neonates and PK-PD relationships in this age group remain elusive.

TABLE 8.2

Propofol Dose Requirements in Children Younger Than 3 Years of Age

(Adapted from Steur RJ, Perez RS, De Lange JJ.. Dosage scheme for propofol in children under 3 years of age. Paediatr Anaesth. 2004;14:462-467.)

Time (minutes)	0-3 months	3-6 months	6-9 months	9-12 months	1-3 years
0–10	24.3	19.7	15.3	14.8	12.1
10–20	20.4	15.2	12.3	11.9	9
20–30	15.1	12	9	9	6
30–40	12	9	6	6	6
40–50	9	6	6	6	6
50–60	6	6	6	6	6

Infusion rates are expressed as milligrams per kilogram per hour.

Linking Pharmacokinetics With Pharmacodynamics

A simple situation in which drug effect is directly related to concentration does not mean that drug effects parallel the time course of concentration. This occurs only when the concentration is low in relation to EC ₅₀ . In this situation, the half-life of the drug may correlate closely with the half-life of drug effect.

A plasma concentration-effect plot can form a hysteresis loop because of a delay in effect. Hull et al. and Sheiner et al. introduced the effect compartment concept for muscle relaxants. A single first-order parameter (T _1/2 keo) describes the equilibration half-time:

T_{1 / 2} keo = \frac{L n (2)}{keo}

$T_{1 / 2} keo = \frac{L n (2)}{keo}$

This mathematical trick assumes the concentration in the central compartment is the same as that in the effect compartment at equilibration, but that a time delay exists before drug reaches the effect compartment. The concentration in the effect compartment is used to describe the concentration-effect relationship.

Adult T _1/2 keo values are well described—for example, morphine 16 minutes; fentanyl 5 minutes; alfentanil 1 minute; propofol 3 minutes. This T _1/2 keo parameter is commonly incorporated into TCI pumps to achieve a rapid effect-site concentration.

There are few estimates of the T _1/2 keo for propofol in children using simultaneous PK-PD modeling. An estimate of 1.86 min (95% confidence interval [CI] 1.16–2.31) was reported in healthy children 2 to 12 years, and 1.2 minutes (95% CI 0.85–2.1) in obese children. We might expect a smaller T _1/2 keo with decreasing age based on size models. Faster half-times in children can be accounted for by considering the physiologic time that scales to a power of :

T_{1 / 2} {keo}_{Child} = T_{1 / 2} {keo}_{ADULT} \times {(\frac{WT}{70})}^{1 / 4}

$T_{1 / 2} {keo}_{Child} = T_{1 / 2} {keo}_{ADULT} \times {(\frac{WT}{70})}^{1 / 4}$

An increasing T _1/2 keo with age (linked to weight) has been described for propofol in children ( Fig. 8.3 ). Similar results have been demonstrated for sevoflurane and BIS in patients 3 to 71 years. If unrecognixed, this will result in excessive dose in a young child if the effect site is targeted and peak effect (Tpeak) is anticipated to be later than it actually is because it was determined in a teenager or adult ( Fig. 8.4 ).

FIGURE 8.3, This figure shows the equilibration rate constant of propofol and time to peak propofol concentration as a function of age. The keo decreases with age; that is. the T 1/2 keo increases with age. This results in a later time to peak concentration (Tpeak) as age (and weight) increases.

FIGURE 8.4, This figure shows simulated plasma time-concentration profiles for a typical 20-kg child given propofol 3 mg/kg using the Kataria parameter set. The T 1/2 keo used will affect predicted effect-site concentrations—that is, the greater the T 1/2 keo, the longer it takes to achieve the target concentration.

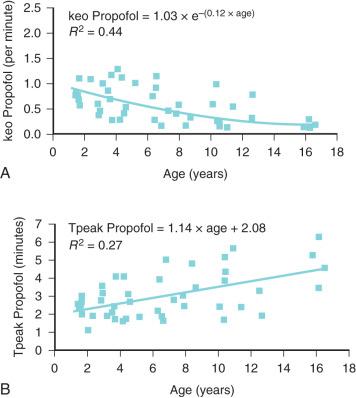
When both PK and PD data are collected simultaneously and parameters for both models are estimated together, then the model is described as “integrated.” PK estimates should not be used in conjunction with PD estimates from a different data set without a few “fudge factors.” Tpeak methodology (see Chapter 7 ) is commonly used to estimate T _1/2 keo that then links separate PK and PD data sets. Tpeak will increase with age (see Fig. 8.3 ). Model dependence of the T _1/2 keo was demonstrated by an estimate of 1.7 min with the Kataria parameter set and 0.8 minute with the Paedfusor model (Graseby Medical Ltd., Hertfordshire, UK) parameter set.

Pharmacodynamic Interaction Models

Drugs may interact with each other at multiple levels. These are discussed in Chapters 6 and 7 . Drug interactions for those drugs used in TIVA are commonly described using PD interaction models.

Traditional Methods

Traditional methods of evaluating PD interactions include using isoboles (graphs illustrating equi-effective combinations of drugs), shifts in dose (or concentration) response curves (see Chapter 7 ), or interaction indexes based on parameters of potency derived from separate monotherapy and combination therapy analyses. Such methods provide an estimation of the magnitude of effect for dose or concentration combinations, but they do not inform us on the time course of that effect or its associated variability.

Competitive antagonists reduce receptor availability by competing for occupancy at the same receptor site. Drugs that elicit an effect are called agonists, whereas those that do not are called antagonists, so the occupancy of some receptors by the antagonists results in less effect. In general, competitive antagonists shift the effect-concentration curve to the right by altering the EC ₅₀ . Non-competitive antagonists shift the observed maximum effect (Emax) rather than the EC ₅₀ .

Response Surface Models

Emax models for individual drugs can be combined and extended to incorporate PD interactions between two or more drugs. The “response surface” models are an extension of empirical, single-drug models that can be used to describe and predict the combined effects between two or more drugs ( Fig. 8.5 ). Horizontal lines within the response surface hold equivalent information to that given by isoboles. Surface parameters are estimated using data points pertaining to all areas of the concentration and effect range for both drugs simultaneously (as opposed to considering individual concentration pairs or effect levels in isolation, as is done with isobolographic analyses). Resulting PD models can be used to characterize the type of interaction across the entire range of concentrations and responses and make predictions about response (effect) for any ratio of the studied drugs.

FIGURE 8.5, Methods of investigating interactions. A, Shift in response curve analyses involve plotting the concentration (or dose)-effect relationship for one drug alone and in the presence of steady-state concentrations of a second drug. B, Isoboles are constructed using iso-effect lines with curves derived from observations assessed against the expected (or “additive”) response line (B*). Supra-additivity is depicted by curves bowing toward the plot origin (C*), while infra-additivity is shown with outward curves (D*). Information from both methods is represented within response surfaces with isoboles displayed as horizontal planes and individual concentration-response curves as vertical slices (indicated by arrows on surfaces for A* single concentration-response curve drug A, B* additive isobole, and C* supra-additive isobole). C shows the additive response surface for two drugs. D shows the synergistic response surface for two drugs, with synergy depicted through outward bowing of the surface.

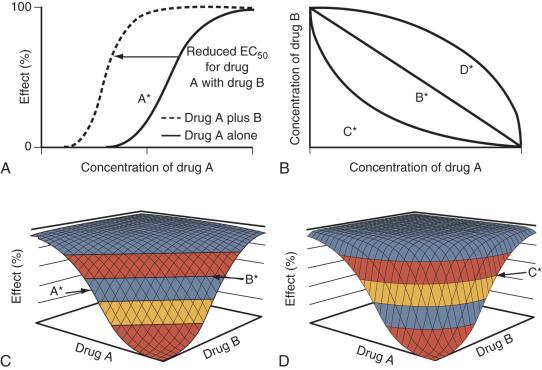
Two equations are commonly used: those of Greco et al. and Minto and Vuyk (see Chapter 7 ). The Greco equations have been used to describe additive effects for propofol, remifentanil, and fentanyl on BIS response in children aged 1 to 16 years undergoing general surgery. These authors reported EC ₅₀ estimates of propofol 5.20 µg/mL, remifentanil 24.1 ng/mL, and fentanyl 8.6 ng/mL, and suggested a propofol and remifentanil pair of 2.3 µg/mL and 4.3 ng/mL, respectively, to maintain hemodynamic parameters and sedation scores within ranges suitable for surgery. The Greco model has also been used to describe loss of response to various noxious stimuli under propofol-remifentanil anesthesia. Synergistic surfaces for sedation and response to laryngoscopy were reported.

The Minto equations have been used to assess synergy for hypnosis among three commonly combined drugs for anesthesia: propofol, midazolam, and alfentanil. Computer simulations based on interactions at the effect site predicted that a synergistic three-drug combination (midazolam, propofol, and alfentanil) tripled the duration of effect compared with propofol alone. Response surfaces can describe anesthetic interactions, even those among agonists, partial agonists, competitive antagonists, and inverse agonists.

Synergism between propofol and alfentanil has been demonstrated using response-surface methodology. Remifentanil alone had no appreciable effect on response to shaking and shouting or response to laryngoscopy, whereas propofol could ablate both responses. Modest remifentanil plasma concentrations dramatically reduced the concentrations of propofol required to ablate both responses. When comparing the different combinations of midazolam, propofol, and alfentanil, the responses varied markedly at each endpoint assessed and could not be predicted from the responses of the individual agents. Similar response-surface methodology has been used to investigate the combined administration of sevoflurane and alfentanil and remifentanil and propofol on control of ventilation. These combinations have a strikingly synergistic effect on respiration, resulting in severe respiratory depression in adults.

The ability of propofol to ablate responses to noxious stimuli has been studied in children between 3 and 10 years undergoing esophagogastroduodenoscopy. The EC ₅₀ for 50% probability of no response was reduced from a propofol concentration of 3.7 µg/mL to 2.8 µg/mL when it was combined with a remifentanil infusion at 0.025 µg/kg per minute. Remifentanil infusions at greater infusion rates did not further reduce the propofol requirements, but they did increase the risk of remifentanil-related adverse respiratory events. The concurrent administration of opioids during TIVA techniques in children has a significant “propofol-sparing” effect while providing analgesia and stress control. It is sensible to take advantage of this synergism to avoid excessive propofol dosing and long-chain triglyceride loads, particularly with concerns about PRIS during prolonged surgeries or sedations. Remifentanil provides the most effective propofol-sparing effect, but fast recovery means alternative techniques of analgesia must be well established before the remifentanil is discontinued. Other analgesics and anesthetics are also effective in reducing the propofol dose required. Fentanyl, alfentanil, and sufentanil are effective, as are local and regional analgesia techniques, once the block becomes established. Nitrous oxide as well as low concentrations of inhalational anesthetics also act synergistically with propofol and opioids to attenuate the dose required.

Depth of Anesthesia Monitoring

A common effect measure used to assess depth of anesthesia is the electroencephalogram (EEG) or a modification of detected EEG signals (spectral edge frequency, BIS, entropy). Physiologic studies in adults and children indicate that EEG-derived anesthesia depth monitors can provide an imprecise and drug-dependent measure of arousal. Although the outputs from these monitors do not closely represent any true physiologic entity, they can be used as guides for anesthesia and in so doing have improved outcomes in adults. In older children the physiology, anatomy, and clinical observations indicate the performance of the monitors may be similar to that in adults. The BIS showed a close relationship with the modeled effect-site propofol concentration and serves as a measure of anesthetic drug effect in children older than 1 year. Their use in infants anesthetized with propofol cannot yet be supported in theory or in practice. During anesthesia, the EEG in infants is fundamentally different from the EEG in older children; there remains a need for specific neonate-derived algorithms if EEG-derived anesthesia depth monitors are to be used in neonates.

Depth of anesthesia monitors were used in 1% of all general anesthetics in the United Kingdom in 2015. However, these monitors were used in 33% of children undergoing general anesthesia using a TIVA technique with a neuromuscular blocking drug, but not at all in infants. Despite limitations in infants, BIS is useful in older children, particularly when neuromuscular blocking drugs are used in conjunction with TIVA; modified EEG has also been successfully used for closed-loop anesthesia. These systems may prove superior to open-loop systems that rely on a calculated effect Ce that are associated with large variability.

Awareness has been more commonly reported after TIVA than after gaseous anesthesia. However, many reports of awareness occur after the switch from gaseous anesthesia to TIVA where a loading dose was not given. Clearance of the gaseous anesthesia agent occurs before attainment of effective steady-state propofol concentrations. The future ability for point-of-care propofol assay using either breath or plasma may be useful to reduce this complication.

Common TIVA Drugs

The commonly used medications include propofol, remifentanil, alfentanil, and sufentanil; ketamine is occasionally used but has a long context-sensitive half-time with consequent delayed awakening. Delivery can be achieved using either a manual infusion scheme ( Table 8.3 ) or PK model–driven infusion devices with software developed specifically for use in children. Unfortunately, the commercially available software packages usually limit the applicable age to 1 to 3 years or older or weight to 10 to 15 kg or greater, and the PK parameters are derived from studies of a relatively few healthy children. Propofol programs that allow for age-, weight- and gender-related changes in central compartment volume, clearance, and distribution have been developed and perform well in healthy children. However, there are considerable gaps in knowledge for some drugs, for ill children, and for young children, infants, and neonates. Consequently, caution is needed when applying such programs to these populations. The anesthesiologist can use these preprogrammed devices as a basis for initiating a TIVA technique but must also use skill, knowledge, and experience to titrate the IV agents to effect to avoid awareness, pain, and adverse effects.

TABLE 8.3

Manual Infusion Schemes

Drug	Loading Dose	Maintenance Infusion	Notes
Propofol	1 mg/kg	10 mg/kg per hour for 10 minutes, then 8 mg/kg per hour for 10 minutes, then 6 mg/kg per hour thereafter	Adult regimen to achieve blood concentration of 3 µg/mL
Propofol	1 mg/kg		Underdelivers to children and achieves lower blood concentration of 2 µg/mL
Propofol	1 mg/kg	13 mg/kg per hour for 10 minutes, then 11 mg/kg per hour for 10 minutes, then 9 mg/kg per hour thereafter	Concurrently with alfentanil infusion
Alfentanil	10–50 µg/kg	1–5 µg/kg per minute	Results in blood concentration of 50–200 ng/mL
Remifentanil	0.5 µg/kg per minute for 3 minutes	0.25 µg/kg per minute	Produces blood concentrations of 6–9 ng/mL
Remifentanil	0.5–1.0 µg/kg over 1 minute	0.1–0.5 µg/kg per minute	Produces blood concentrations of 5–10 ng/mL
Sufentanil	0.1–0.5 µg/kg	0.005–0.01 µg/kg per minute	Results in blood concentration of 0.2 ng/mL for sedation and analgesia
Sufentanil	1–5 µg/kg	0.01–0.05 µg/kg per minute	Results in blood concentrations of 0.6–3.0 ng/mL for anesthesia
Fentanyl	1–10 µg/kg	0.1–0.2 µg/kg per minute
Ketamine	1–2 mg/kg	0.1–2.5 mg/kg per hour	Smaller dose and infusion rate for analgesia and sedation. Larger dose and infusion rate for anesthesia titrated to effect
Midazolam	0.05–0.1 mg/kg	0.1–0.3 mg/kg per hour

Achieving the Target Concentration in a Multicompartment Model

For a fixed infusion rate in a single-compartment model, it takes five half-lives to reach a steady-state concentration (>96% of the target) in the blood ( Fig. 8.6 ). A loading dose is required to more rapidly achieve the target concentration. This dose rapidly fills the volume of distribution, after which the calculated infusion maintains the blood concentration ( Fig. 8.7 ). Calculation of the loading dose is relatively easy for a simple one-compartment model. Unfortunately, drugs such as propofol require two- or three-compartment models to describe their disposition. A loading dose may be too large if calculated using the volume of distribution at steady state (Vss, where Vss = V1 + V2 + V3 for a three-compartment model) for a drug that is described by multiple compartments because it is initially administered into the smaller central compartment (V1) to effect the desired response: loss of consciousness. A loading dose based on the Vss will cause adverse effects, hemodynamic instability, and/or toxicity. Even a remifentanil bolus should be administered over several minutes to reduce the risk of bradycardia, hypotension, and/or difficult mask ventilation. An anticholinergic drug may prove useful if remifentanil 3 µg/kg is used for rapid-sequence intubation.

FIGURE 8.6, A fixed-rate infusion of propofol at 10 mg/kg per hour with no bolus dose in a healthy 10-kg, 1-year-old infant. Note that a steady state is not reached even after 1 hour. There is a lag of effect-site concentration behind blood concentration both during infusion and after stopping infusion. Effect-site concentration reaches blood concentration at about 1 hour. The context-sensitive half-time is 9 minutes (simulated using Tivatrainer; available at http://www.eurosiva.org/TivaTrainer/tivatrainer_main.htm ).

FIGURE 8.7, This figure illustrates the manual infusion technique in a healthy 70-kg 40-year-old. Note the importance of the early higher initial infusion rates to ensure that the target concentration is more constant. (Data from the Diprifusor pharmacokinetic data set.) Bolus dose (propofol 1%) was 1 mg/kg, then 10 mg/kg per hour for 10 minutes, 8 mg/kg per hour for 10 minutes, then 6 mg/kg per hour thereafter until 60 minutes when the infusion is discontinued. The maximum blood concentration is 4.5 µg/mL. Effect-site concentration reaches 3 µg/mL after around 10 minutes but drifts down to around 2.6 µg/mL and then very gradually rises. The context-sensitive half-time after 1-hour infusion is 7 minutes.

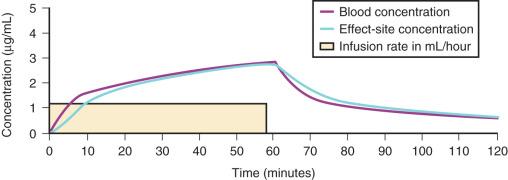
To more clearly understand the disposition of drugs after an IV dose, it is useful to consider a three-compartment model ( Fig. 8.8 , Table 8.4 ). The drug is delivered and eliminated from a central compartment V1 (which includes the blood) but also distributes to and redistributes from two peripheral compartments, one representing well-perfused organs and tissues (fast compartment, V2) and the other representing more poorly perfused tissues such as fat (slow compartment, V3). The transfer of the drug between V1 and the two peripheral compartments (V2, V3), in addition to the elimination of the drug from V1, is described by a series of clearances (CL, Q2, Q3) indicating the distribution back and forth between paired compartments, such as V1 to V2 and then V2 back to V1. The primary target organ that intravenous anesthetic agents affect is the brain. Therefore, an additional rate constant is added to describe the equilibration between the central compartment and the effect site in the brain (keo = k1e at equilibrium). This compartment is not represented by a volume but rather by the time required to equilibrate. Consequently, there is a time lag before changes in the blood concentration are reflected in the effect site (shown on Figs. 8.6 and 8.7 ).

FIGURE 8.8, A three-compartment model with an additional compartment used to describe concentration in the effect compartment. A single first-order parameter (k1e = keo at steady state) describes the equilibration rate between the central (V1) and effect compartment. This compartment model is conceptualized in Fig. 8.9 , where hydraulics are used to illustrate compartment interactions. The arrows in this figure could be considered “pipes” that deliver drug into the central compartment, out rapidly to V2 (and back to V1) and out more slowly to V3 (and back to V1) and are then eliminated through simultaneous clearance. k1e is the rate constant describing drug movement from compartment 1 to the effect compartment.

TABLE 8.4

Nomenclature for TCI Systems

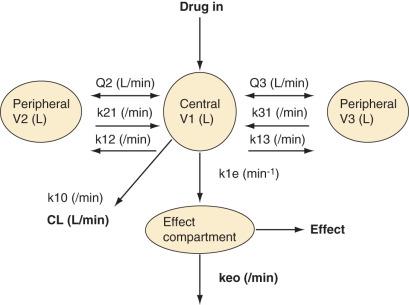
Term	Meaning	Units
TCI	Target-controlled infusion
Vc or V1	Central compartment volume	L
V2	Fast compartment volume (vessel-rich group) = V1 × k ₁₂ /k ₂₁	L
V3	Slow compartment volume (vessel-poor group) = V3 × k ₁₃ /k ₃₁	L
Cl 1 or CL	Elimination clearance = V1 × k ₁₀	L/hour
Cl 2 or Q2	Clearance between V1 and V2 = V2 × k ₂₁	L/hour
Cl 3 or Q3	Clearance between V1 and V3 = V3 × k ₃₁	L/hour
Cp	Blood concentration
Ce	Effect-site concentration
T	Target concentration
CALC	Concentration calculated by TCI software
MEAS	Concentration measured
k ₁₀	Elimination rate constant	/minute
keo	Rate constant for equilibration between blood and effect-site	/minute
T _1/2 keo	Half-time for equilibration between blood and effect site	minutes
k ₁₂ , k ₂₁	Rate constants for movement between V1 and V2	/minute
k ₁₃ , k ₃₁	Rate constants for movement between V1 and V3	/minute

A hydraulic model is useful for understanding these concepts. The central compartment is connected to the peripheral compartments and effect site by a series of pipes of different diameters and also a drainage pipe to represent elimination ( Fig. 8.9A-F ). The height of the columns of fluid, which represents the concentration of drug, illustrates the gradient down which the drug travels between the central and peripheral compartments; this can be animated over time to show filling and emptying of compartments relative to each other. The diameter of the interconnecting pipes between the central and peripheral compartments represents the intercompartment clearances (Q2, Q3), and the size of the drainage channel represents elimination (CL). This hydraulic analogy is used in the TIVA Trainer simulation program.

FIGURE 8.9, A, Hydraulic model representation of effect-site TCI. Compartment volumes and intercompartmental clearance values for the Paedfusor model in a healthy 10-kg, 1-year-old infant. In the first few minutes the central compartment to effect-site concentration gradient is marked to “overpressure” the transfer of propofol to the effect site. The peak blood concentration is 7.1 µg/mL. Distribution from the central compartment (C1) to the first peripheral compartment (C2) occurs rapidly also, which slows the rise in effect-site concentration and blood concentration. Elimination from C1 and distribution from C1 to the second peripheral compartment (C3) is also occurring. B, At 4.5 minutes, the effect-site concentration has reached the target of 3 µg/mL and has equilibrated with the concentration in C1. The infusion device, which has been off after the initial loading infusion, now switches back on to maintain the effect-site target concentration at 3 µg/mL. C, After 1 hour of maintenance at an effect-site concentration of 3 µg/mL, the target is set to 0 µg/mL and the pump switches off. A total of 14 mL of propofol (1%) has been administered, or 14 mg/kg. There is now a considerable accumulation of propofol in C2 and C3, while C1 and the effect site are still in equilibrium. D, Approximately 10 minutes after the effect-site target concentration is set to 0, the blood concentration has halved; thus the context-sensitive half-time is 10 minutes. The lag in the decline in effect-site concentration is clearly seen, and there is now a gradient between effect site and C1. There is now also a concentration gradient from C2 to C1 and to C3 and this slows the decline in the concentration in C1. E, One hour after the infusion is stopped, the blood and effect-site concentrations have fallen to of the maintenance effect-site concentration. There are still considerable quantities of propofol in compartments C2 and C3 that slow the decline of the concentration of C1 and effect-site concentrations. F, Even after 4 hours, the depot of propofol in C2 and C3 is considerable, although blood and effect-site concentrations are extremely low. However, these low concentrations may still be exerting significant antiemetic and anxiolytic effects (data from the Paedfusor pharmacokinetic data set).

Fixed Infusion Rate and a Three-Compartment Model

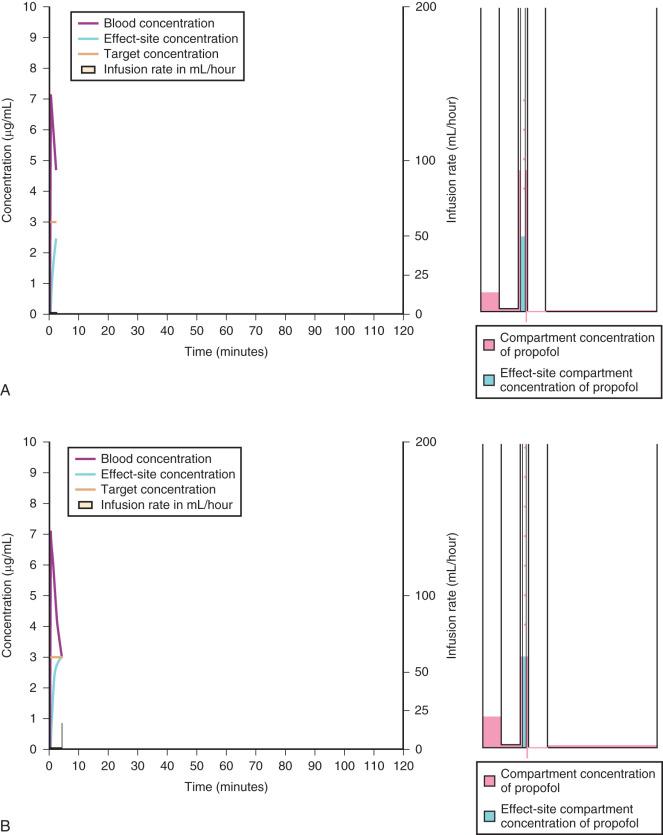
When a fixed infusion rate is started (see Fig. 8.6 ), the blood concentration will increase but, almost simultaneously, distribution of the drug to the fast compartment and elimination both begin. Distribution of drugs throughout the body contributes more to the removal of drug from blood than elimination for most medications. Remifentanil is an exception; it has extremely rapid esterase clearance and elimination of this drug is far greater than redistribution from the central compartment. As the concentrations within each compartment equilibrate, the concentration gradient between compartments lessens (slowing drug transfer between compartments) but distribution to the slow compartment continues along with elimination. The net effect is that the blood concentration continues to increase, albeit at a slower rate. As the blood concentration increases toward equilibrium, elimination becomes relatively more important; Fig. 8.6 illustrates how far behind the effect-site concentration lags. Eventually, after several hours (or in some cases, days), a steady state is reached where the infusion rate is directly proportional to clearance.

Bolus and Variable Rate Infusion in a Three-Compartment Model

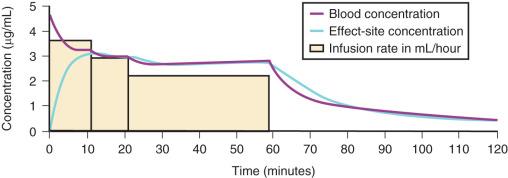
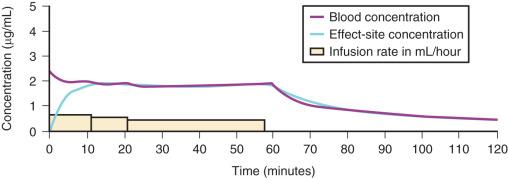
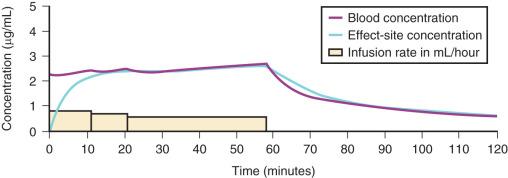
A loading dose can start to fill the central compartment and ideally should attempt to create an effect-site concentration at a specific target concentration without overshoot. Then the rate of infusion should decrease in a stepwise manner to maintain a constant effect-site concentration until a steady state is reached. As drug is delivered into the central compartment, it continuously distributes to the peripheral compartments while it is also continuously eliminated. The infusion rate must vary because it has to match the concurrent changes in the contribution of distribution and elimination with time ( Figs. 8.10 and 8.11 ). When the infusion is stopped, then elimination will continue to drain the central compartment, and drug will continue to distribute to V2 and V3 along concentration gradients from V1 for some time. Equilibrium may be reached, but the drug now begins to move back from the peripheral compartments into the central compartment, maintaining the central compartment drug concentration. This can continue for a protracted interval, particularly for highly lipid-soluble drugs that have a very large slow compartment V3 contributing a reservoir or depot effect (e.g., fentanyl; see Fig. 8.9F ). Eventually the central compartment concentration will decrease. For most anesthetics, the longer the duration of an infusion, the more the drug has distributed into the peripheral compartments and the larger the reservoir of drug to be redistributed back into the central compartment and eliminated once the infusion ceases. The half-time of the decrease in drug concentration in blood is related to the duration of the infusion for most drugs (except remifentanil). This is termed the context-sensitive half-time (CSHT), where the context is the duration of the infusion and relates to a pseudo-steady state maintained by a TCI. For an individual drug in an individual patient, CSHTs can be plotted against the duration of the infusion ( Fig. 8.12A and B ). The CSHT will eventually asymptote and this is when the pseudo-steady state becomes a true steady state. At that time, the infusion has become context in sensitive. This pattern is observed for nearly all IV anesthetics. In the case of propofol, the slope of the context-sensitive half-life with time increases from adults to children to infants to full-term and then preterm neonates, with preterm neonates having the greatest half-life of all age groups. The exception is remifentanil, whose half-time becomes context insensitive almost immediately after initiation of the infusion because its elimination is rapid and complete; the capacity of the red cell, plasma, and tissue esterase enzyme systems are enormous.

FIGURE 8.10, This figure illustrates the manual infusion technique of propofol in a healthy 1-year-old, 10-kg child. Note the importance of the early higher initial infusion rates to ensure that the target concentration is more constant. The adult dose regimen is illustrated. The figure shows a bolus dose of 1 mg/kg, then 10 mg/kg per hour for 10 minutes, then 8 mg/kg per hour for 10 minutes, then 6 mg/kg per hour thereafter. The infusion was stopped at 60 minutes. The effect-site concentration dose does not equilibrate until 11 minutes. The blood and effect-site concentrations stabilize around 1.8 µg/mL. However, this is unlikely to represent a sufficient depth of anesthesia for surgery. The context-sensitive half-time after 1 hour infusion is 9 minutes (data from the Paedfusor pharmacokinetic data set).

FIGURE 8.11, Manual infusion of propofol in 1-year-old, 10-kg child. The figure shows a bolus dose of 1 mg/kg, then 13 mg/kg per hour for 10 minutes, then 11 mg/kg per hour for 10 minutes, then 9 mg/kg per hour thereafter. The infusion was stopped at 60 minutes. The effect-site concentration does not equilibrate until 20 minutes. The blood and effect-site concentrations stabilize around 2.4 µg/mL but gradually rise over the next hour to 2.6 µg/mL. This concentration is larger than that achieved using the adult 10-8-6 regimen but may still be inadequate. Larger infusion doses are required in a 1-year-old child.

FIGURE 8.12, A, Context-sensitive half-times (CSHTs) after short-duration infusions. B, CSHTs after longer-duration infusions. For very lipid soluble drugs such as fentanyl and propofol, V3 is very large compared with V1. Intercompartmental clearance between V1 and V3 is given by the equation V1 χ k 13 = V3 χ k 31 , which implies that if V1 is much smaller than V3, rapid distribution from V1 to V3 is associated with very slow redistribution from V3 to V1. This is indeed seen with propofol and fentanyl, which have a slow offset of effects after prolonged infusions. Propofol has a CSHT that varies between approximately 3 minutes for a short-duration infusion to approximately 18 minutes after a 12-hour infusion. This is because elimination is quite rapid compared with the rate of redistribution from V3. For alfentanil, the concentration of the un-ionized form is 100 times greater than that of fentanyl (pKa alfentanil 6.4, fentanyl 8.5). Alfentanil therefore has a more rapid onset time and shorter half-life keo, a smaller V1, lower volume of distribution at steady state, and lower clearance than fentanyl. Fentanyl does, however, have a shorter CSHT than alfentanil after a short-duration infusion lasting less than 2 hours (A) ; but for longer-duration infusions, alfentanil reaches a maximum CSHT after about 90 minutes, whereas for fentanyl the CSHT continues to increase after 12 hours (B) . This is because fentanyl has a huge V3, and redistribution back to V1 maintains the blood concentration when the infusion stops. (Simulated using Tivatrainer; available at www.eurosiva.org/TivaTrainer_main.htm .)

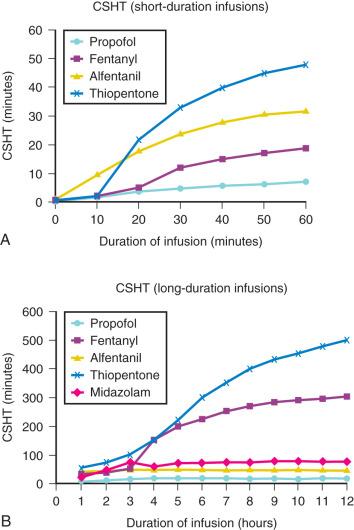
PK parameters for remifentanil, alfentanil, and sufentanil are summarized in Table 8.5 . The differences in context-sensitive half-times are illustrated in Table 8.6 and Fig. 8.12A and B . Fentanyl has a small CSHF when given by infusion for a short time, but this dramatically increases as the duration of the infusion increases. Alfentanil's CSHF reaches a plateau after approximately 90 minutes.

TABLE 8.5

Pharmacokinetic Parameters for Short-Acting Opioids

From Absalom AR, Struys MMRF. An Overview of TCI and TIVA . Ghent, Belgium: Academia Press; 2005.

	Remifentanil	Alfentanil	Sufentanil
V1	5.1–0.0201 × (age − 40) + 0.072 × (LBM − 55)	Male: 0.111 × weight Female: 1.15 × 0.111 × weight	0.164 × weight
V2	9.82–0.0811 × (age − 40) + 0.108 × (LBM − 55)	12.0	0.359 × weight
V3	5.42	10.5	1.263 × weight
k ₁₀	2.6–0.0162 × (age − 40) + 0.0191 × (LBM − 55)/V1	0.356/V1	0.089
k ₁₂	2.05–0.0301 × (age − 40)/V1	0.104	0.35
k ₂₁	2.05–0.0301 × (age − 40)/V2	0.067	0.16
k ₁₃	0.076–0.00113 × (age − 40)/V1	0.017	0.077
k ₃₁	0.076–0.00113 × (age − 40)/5.42	0.0126	0.01
keo	0.595–0.007 × (age − 40)	0.77	0.12

Age in years; weight in kilograms.

LBM, lean body mass.

TABLE 8.6

Context-Sensitive Half-Times (minutes) of Opioids in Children

	Infusion Duration (minutes)
Opioid	10	100	200	300	600
Remifentanil	3–6	3–6	3–6	3–6	3–6
Alfentanil	10	45	55	58	60
Sufentanil		20	25	35	60
Fentanyl	12	30	100	200

You're Reading a Preview

Become a Clinical Tree membership for Full access and enjoy Unlimited articles

Become membership

If you are a member. Log in here