Physical Address
304 North Cardinal St.
Dorchester Center, MA 02124
The assessment of left ventricular (LV) function is a fundamental requirement in many clinical situations in cardiology. Numerous techniques are used for this purpose, and a number of parameters can be employed, although ejection fraction (EF) is by far the most established. Echocardiography is the most widely used technique for the assessment of LV function because it is widely available and relatively inexpensive and can be taken to the patient’s bedside. The problem is that echocardiographic assessment of EF is subject to a number of limitations, both technological and physiologic ( Table 1.1 ), which particularly compromise the test-retest variation of this method. The development of strain as a clinical tool over the past 30 years has provided a technique that is accurate and reliable. The year 2020 represented a culmination of this work to produce an additional assessment of LV function because a new Current Procedural Terminology (CPT) code (93356) was introduced for the application of strain in clinical practice. The purpose of this chapter is to provide the technical background regarding this modality, summarize its potential applications, and prepare the reader for subsequent chapters about the main clinical applications of myocardial strain.
| Global (EF) | Regional (WMSI) |
|---|---|
| Variability | Variability |
| Insensitive to mild dysfunction | Distinction of infarcted and ischemic myocardium |
| Inexact in LVH | Inadequate in LBBB |
| Less precise in tachycardia | Less accurate in severe LVD |
| Inability to assess RV and atrial function | |
| Inability to assess diastolic function |
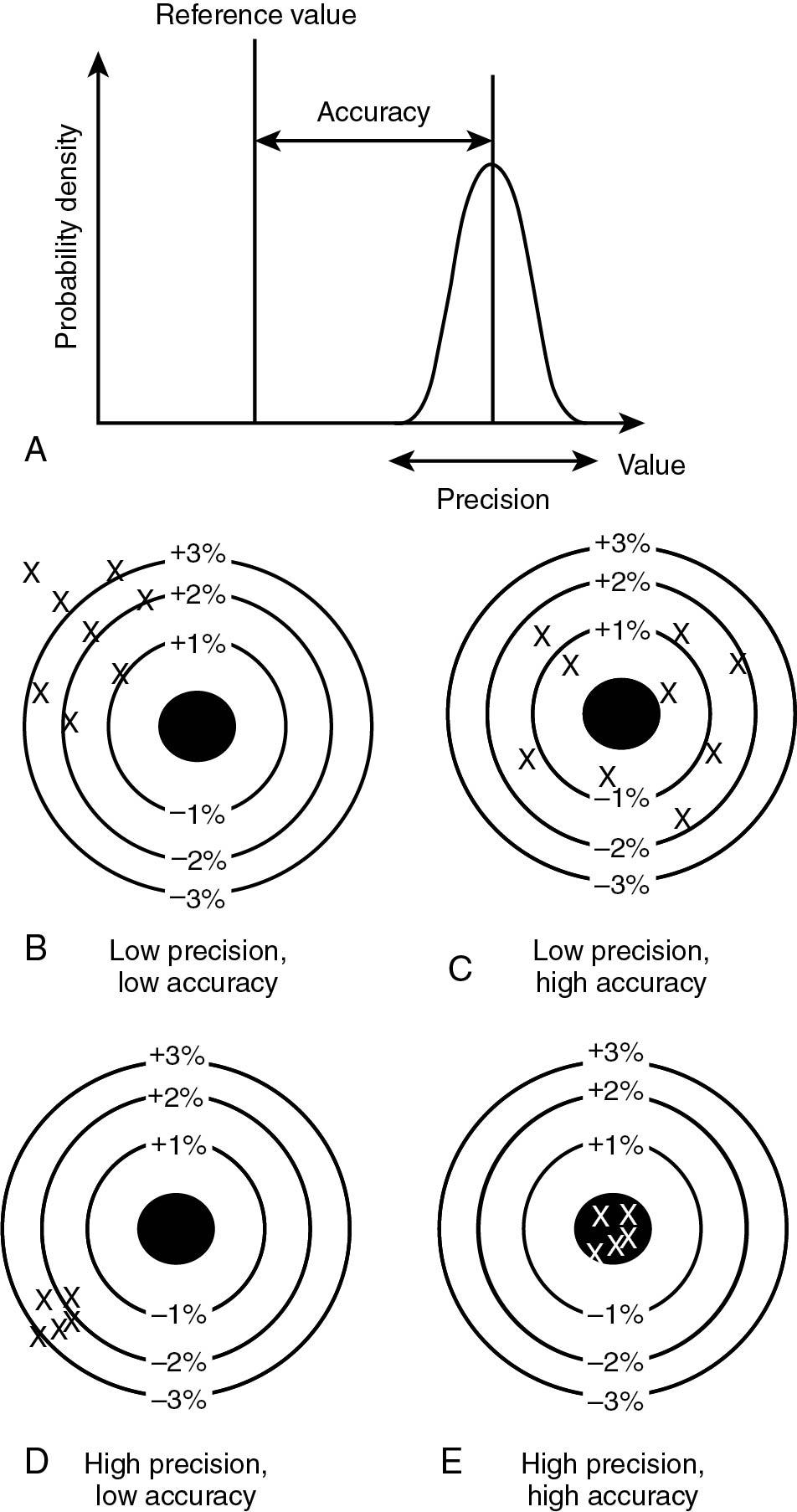
The adoption of a new tool can be difficult. Fundamental to finding the motivation for this effort is a recognition of the limitations of assessment of both global and regional function using current techniques (see Table 1.1 ). There are two aspects of a desirable measurement: validity and reliability ( Fig. 1.1 ). Validity (or accuracy) pertains to the comparison of the measurement against some external standard. The accuracy of strain has been validated experimentally against in vivo measurement of tissue excursion with sonomicrometry and clinically against magnetic resonance tagging techniques. Although validity is important to clinicians, in most circumstances, we are less worried about consistent bias (when the measurement is within, for example, 5% of the reference standard) but more concerned about random variation of the measurement that would preclude our ability to compare individuals or compare measurements at different points of time. This is a reflection of reliability or precision. In sequential testing, the tool has to be sufficiently sensitive to pick up small differences, and reliability can be measured as the ratio of true variance and true+error variance. What is important to realize is that both validity and reliability of existing techniques are inadequate and especially of limited reproducibility. For example, the 95% confidence intervals of two-dimensional (2D)–EF measurement exceed 10%, implying that an apparent reduction of EF from 54% (normal) to 45% (mildly impaired) may simply represent the variability of the measurement. Although three-dimensional (3D)–EF has less variability, it probably still exceeds 5%.
Strain represents a fundamental property of matter, namely its deformation in response to an applied force. This force might be extrinsic to the heart, which is fundamental to the performance of elastography ( Chapter 10 ). However, most commonly, it is intrinsic to the heart, based on myocardial contraction associated with every heartbeat. Because the myocardium is assumed to be incompressible, shortening in one direction is associated with thickening in the orthogonal plane.
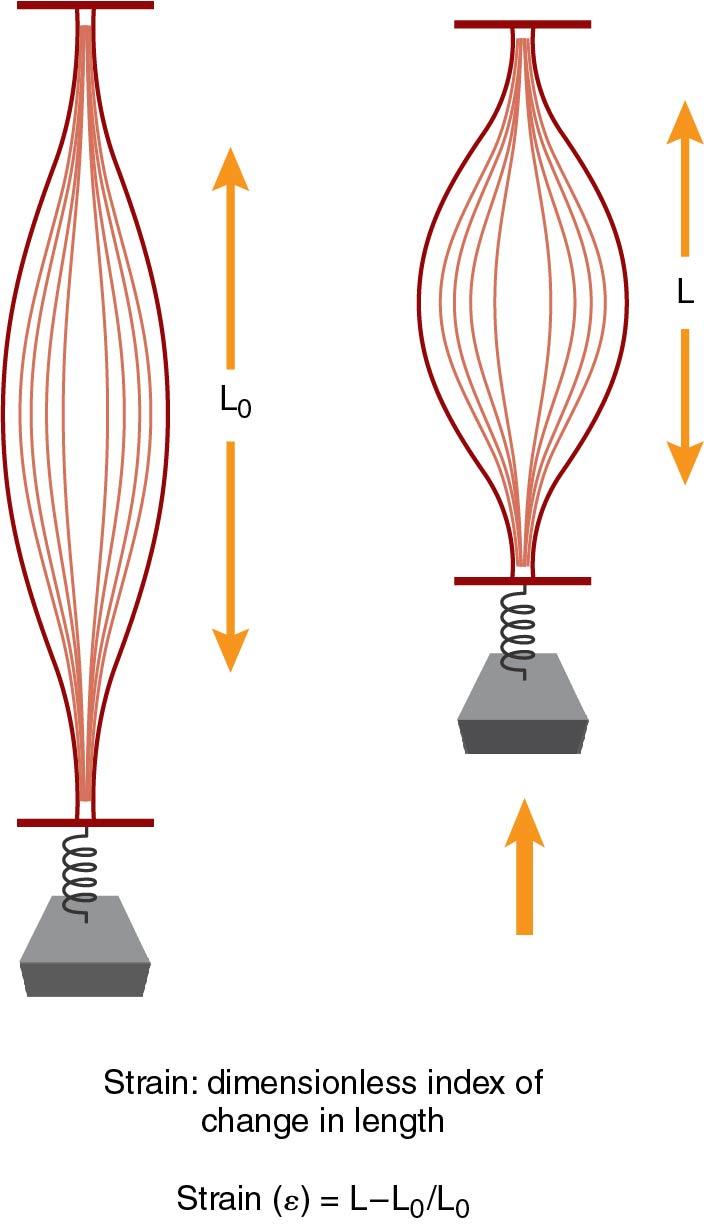
Lagrangian strain (ε) is defined as the difference between myocardial length at baseline (L 0 , usually end diastole) and myocardial length at the end of a time interval (L, usually at end systole) divided by L 0 . This corresponds to the strain measured by speckle strain ( Fig. 1.2 ):
In contrast, if strain is calculated by comparing instantaneous length change during deformation over an infinitesimal small time interval (dt), this strain is termed natural strain, and it corresponds to tissue velocity strain, in which initial segment length is not used as a reference :
In this case, the total amount of strain represents an aggregate of all infinitesimal strain contributions between t 0 and t.
If the strains are small, Lagrangian strain approximately equates to natural strain, but deformations >10% (such as are observed in the cardiac cycle) using each approach are different. The majority of currently used imaging techniques (speckle-tracking echo cardiography, cardiac magnetic resonance, nuclear) measure Lagrangian strain.
Strain is a dimensionless parameter, expressed as a percentage. When applied to longitudinal or circumferential shortening during systole, the resulting values are negative, since L is shorter than L 0 . Conversely, radial thickening is denoted by a positive value.
The measurement of strain takes no cognizance of the timeframe of myocardial motion, although this can be accounted for by assessing strain rate (SR), which represents the average rate of deformation, having the unit s –1 :
Individual myocardial segments may be measured, or strain can be assessed along the length of an entire wall or for the chamber as a whole, the latter being the basis of the most widely used parameter, global longitudinal strain (GLS). Even though this parameter is the most commonly used clinically, it is essential that strain is not used as a black box and that the underlying strain curves are assessed.
Although the expression of longitudinal strain (shortening) as a negative number is completely correct from the standpoint of the underlying physics, it has led to significant confusion in the interpretation of strain by nonexperts. As strain moves from being the domain of researchers and cardiologists to other clinicians (e.g., oncologists in the setting of testing for cardiotoxicity), this has posed a significant problem. Recently, some authors have started to use an absolute (positive) value of GLS in reporting their data. , A recent opinion piece from the editors of an imaging journal proposed that to increase the understanding of GLS, it should be quoted as an absolute, positive value, perhaps labeled as global longitudinal shortening. There is a precedent in the expression of GLS as a positive number because if EF were calculated in the same way, it also would be a negative parameter. This is a matter of ongoing discussion, but at present, the guidelines support its expression as a negative number.
The three dimensions of LV and right ventricular (RV) strain calculation are longitudinal (obtained from the apical views), circumferential (obtained from short axis views), and a measure of myocardial thickening (radial in the short axis and transverse in the apical views). Of these three measurements, longitudinal strain is the most robust and most widely used. There seems to be little incremental information from using circumferential strain, although it has been used to predict LV remodeling. Radial and transverse strain are the least reliable, and one of the reasons for this might be that the amount of myocardium used for this calculation is significantly less than the others. Accordingly, the use of radial strain is not recommended. Moreover, although total deformation is quantified by measuring the three sheer strain components, sheer is currently neglected in clinical practice, with the possible exception of torsion.
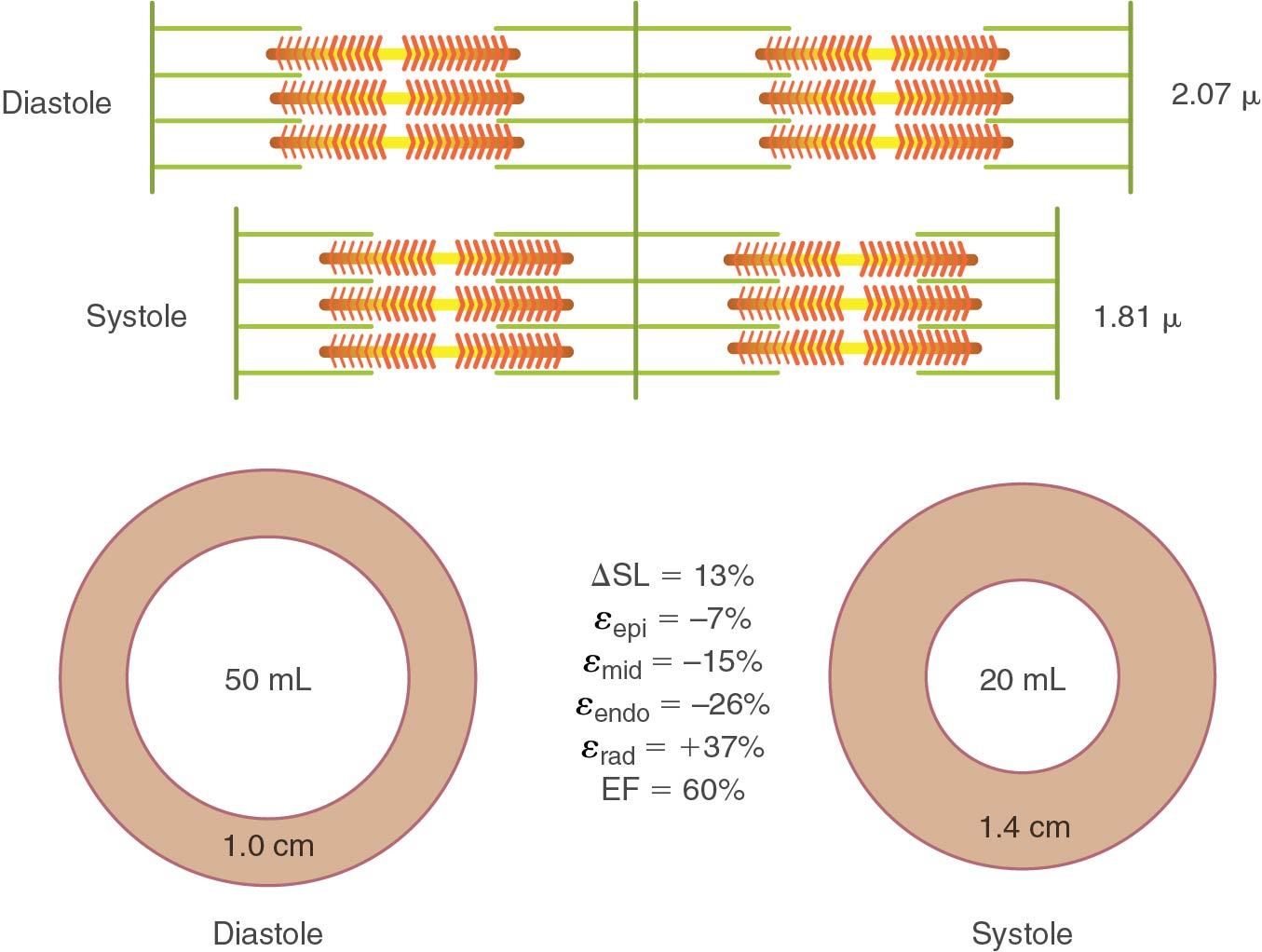
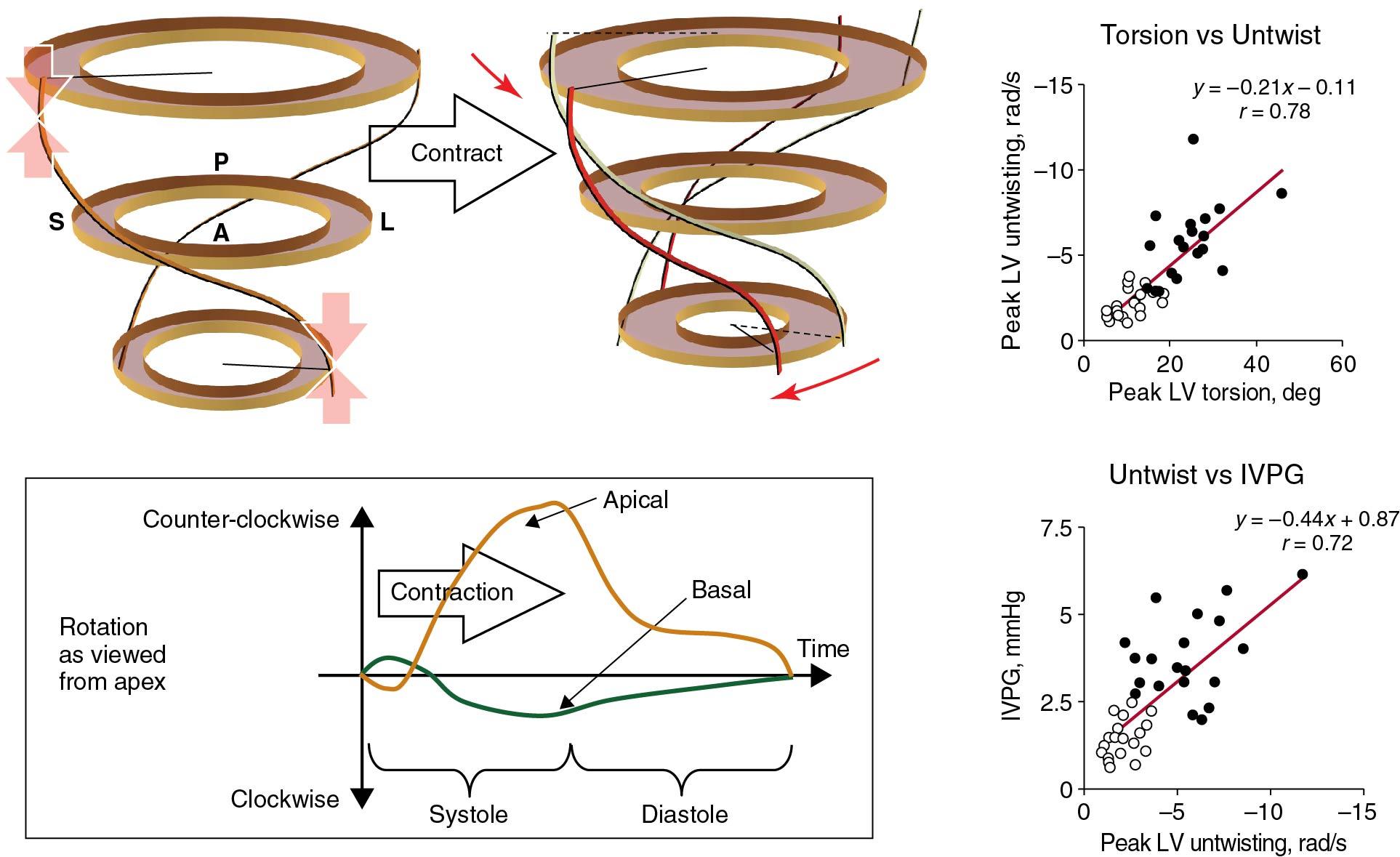
Myocardial fibers are arranged in a helix so that contraction leads to longitudinal shortening as well as radial thickening. This orientation provides a mechanical advantage, whereby approximately 10% shortening of each myofibril is able to translate to a 50% EF ( Fig. 1.3 ). There are multiple myocardial layers, with predominantly longitudinal fibers in the subendocardium and epicardium, and predominantly radial fibers in the midmyocardium. These obliquely orientated myofibers generate an apical counterclockwise twist and a basal clockwise twist driving torsional ventricular contraction. , Torsion correlates with untwist, which contributes to isovolumic pressure decay, generating an intraventricular pressure gradient responsible for diastolic suction and facilitation of LV filling ( Fig. 1.4 ). Two observations are important in relation to this. First, the direction of measurement of strain pertains to the direction of the wall rather than the direction of the fibers themselves, so it is important to consider that these measurements are an approximation. Second, although there is much interest in the separate measurement of subendocardial and subepicardial function, this is dependent on the assumption that there could be differential movement of these components, which is unproven.
Strain is an ejection phase index and is therefore susceptible to loading, particularly afterload, and to a lesser degree to preload and heart rate, both of which involve LV cavity size. Because of this contribution from loading, strain is not an optimal index of contractility. In fact, the closest deformation parameter to this is strain rate, which has been shown to correspond to dP/dt and thus better reflect the inotropic state of myocardium.
Although LV-GLS and LVEF are both ejection phase markers, changes in each do not necessarily correlate. The reason is that EF is associated with GLS, global circumferential strain (GCS), wall thickness, LV size, and diastolic volume. Although LVEF is proportional to the square of GCS, it is only linearly related to GLS. LVEF is inversely related to LV radius, so smaller LV cavities and LV hypertrophy (LVH) are associated with preserved EF, even if GLS is impaired. The implications of these relationships are particularly important in valvular heart disease and are discussed in more detail in Chapter 7 .
Become a Clinical Tree membership for Full access and enjoy Unlimited articles
If you are a member. Log in here