Physical Address
304 North Cardinal St.
Dorchester Center, MA 02124
Acoustic radiation force impulse (ARFI) is a continually developing technology that provides biomechanical information concerning tissue elasticity (stiffness) by emitting a push pulse, which generates laterally propagating shear waves (SWs). In addition to elasticity, viscosity provides biomechanical information concerning the pathologic state of tissue, which is a different property than elasticity alone. However, most ultrasound (US) elastographic models use a linear elastic model to describe tissue mechanical properties, and only tissue elasticity is quantified. Dispersion is related to the frequency dependence of the shear wave speed (SWS) and is affected by the attenuation of SWs because of the viscous component of the tissue. If a tissue is dispersive, the SWS and the attenuation of SWs will increase with frequency. Analysis of the dispersion properties of SWs can therefore serve as an indirect method for measuring viscosity. As of early 2022, dispersion imaging, a new imaging technology, has been developed by a few US vendors including Shear Wave Dispersion Imaging (SWD; Canon Medical Systems, Japan), Viscosity PLUS (Vi PLUS; Hologic, U.S.A.) and Elastance (Columbus, Ohio, U.S.A.). , In this chapter, the feasibility of liver viscosity evaluation is reviewed based on the findings of both animal studies and clinical evaluation in humans.
A homogeneous biological tissue can be modeled using viscoelastic models such as the Voigt and Maxwell models, which consist of a spring and a damper, and the Zener model, which consists of two springs and a damper. Using the Voigt model as an example to illustrate the frequency dependence of SWS, the SWS
at SW frequency ω calculated by the Voigt model is as shown below , :
where ρ , μ , and η are the density, shear elasticity, and shear viscosity of the medium, respectively.
When zero is substituted for shear viscosity η in equation (1) , that is, a perfectly elastic tissue is assumed and viscosity is ignored, SWS
can be transformed to a simple relationship with shear elasticity:
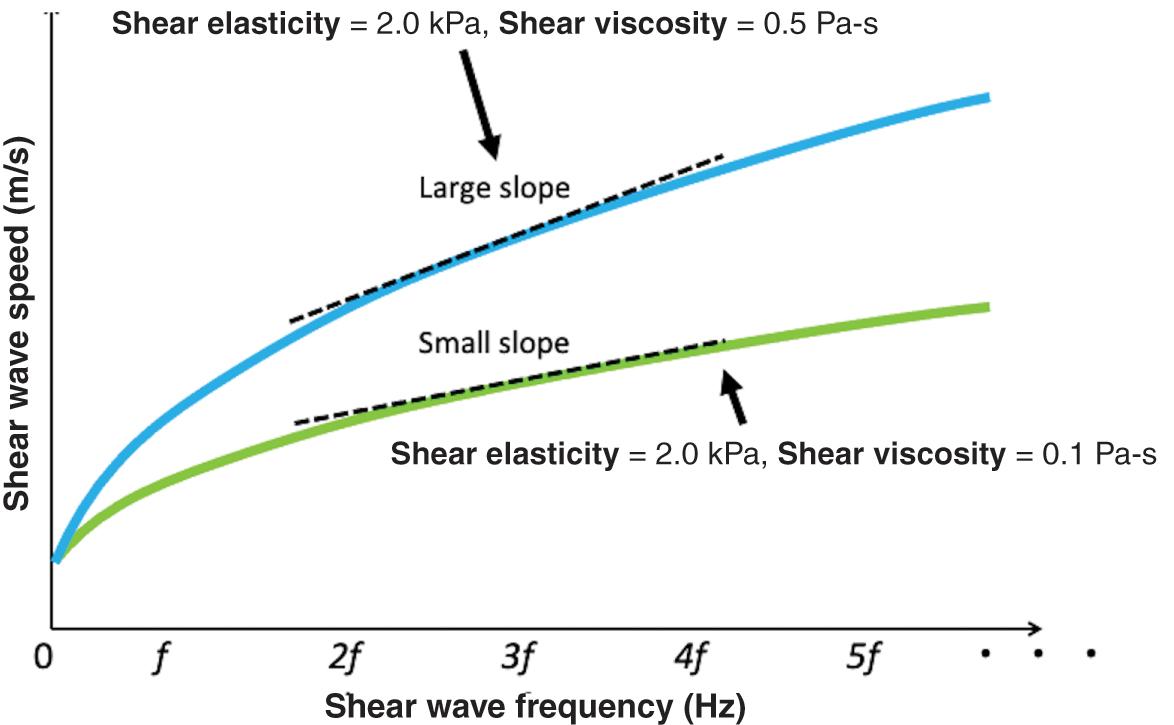
On the other hand, as shown in equation (1) , SWS depends on the frequency of the SWs in a viscoelastic tissue and therefore exhibits frequency dispersion. , The gradient of SWS, that is, the slope of the graph of SWS versus frequency, varies according to the shear viscosity value. Therefore the shear viscosity value can be estimated from the slope over the SW frequency bandwidth ( Fig. 12.1 ). It should be noted that the slope does not measure viscosity directly, but this method provides the quantification of a parameter that is directly related to viscosity without the need to use a rheological model. It must be highlighted that the shear wave dispersion slope (SWDS) value is based on the frequency range; hence different ranges give different values.
For a uniform and device-independent set of measurements, it is necessary to address differences and sources of disagreement, including the different shear wave frequencies used to measure the SWD along with other experimental factors.
A SWD map can be created using an imaging technique that has been incorporated into commercially available US systems (Aplio i-series). This technique can be used to estimate the dispersion slope of SWS versus frequency to evaluate changes in tissue viscosity.
SWD processing involves four steps ( Fig. 12.2 ). In the first step the displacements induced by the SWs are obtained using a technique based on color-Doppler US scanning. Second, the displacement at each location is transformed from the time domain to the frequency domain by a Fourier transform to estimate the phase changes in the SWs at several frequencies. Third, SWS is calculated using the phase-difference method. SWS at each frequency
( ω ) is as shown below:
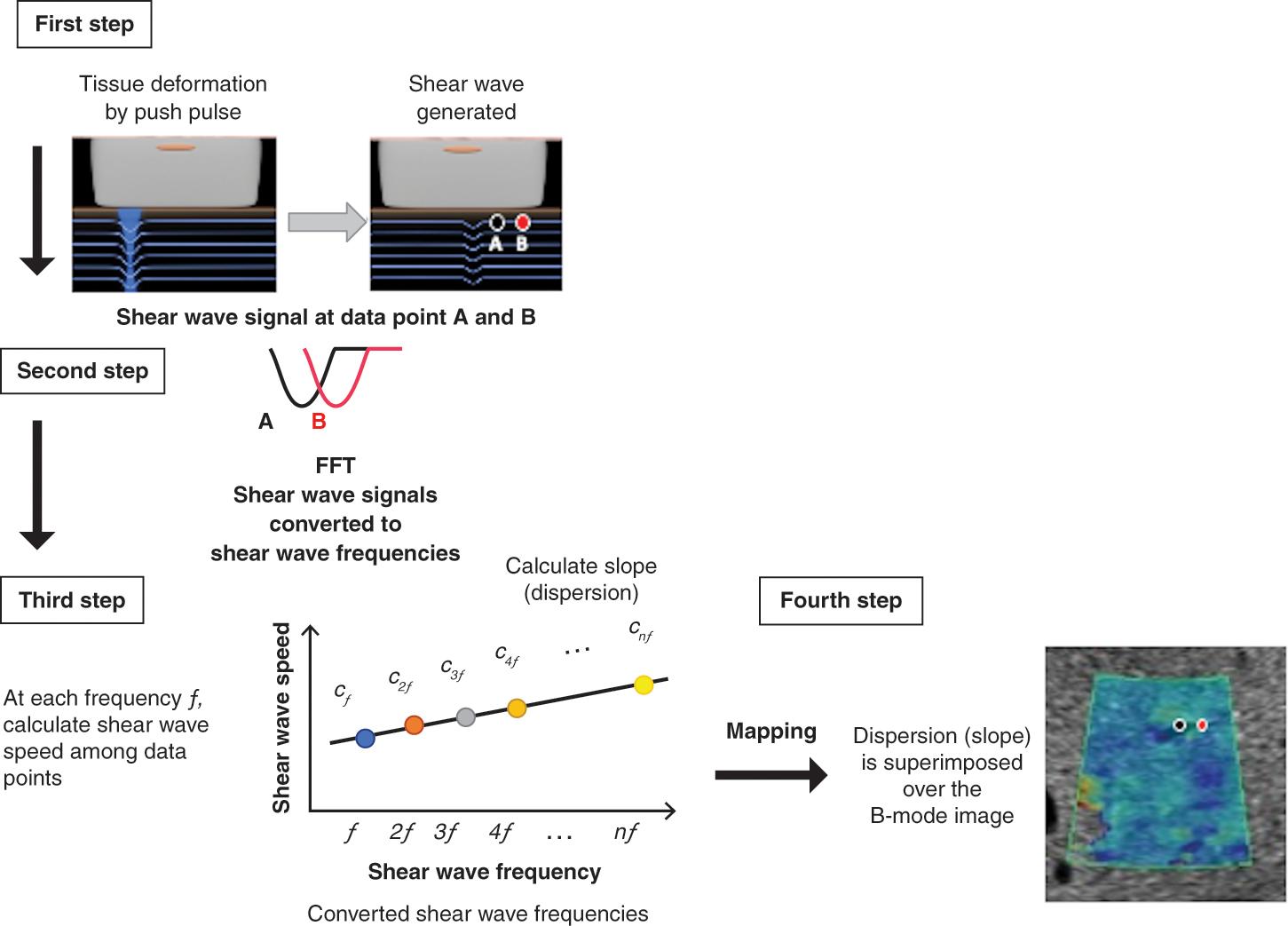
where ∆ ϕ(ω) is the phase change over the distance traveled ( ∆ L) between two measurement locations in the direction of SW propagation. Fourth, the gradient of SWS is calculated based on the distribution of SWS versus frequency. The calculated gradient values are then superimposed on the measurement locations to create a dispersion map.
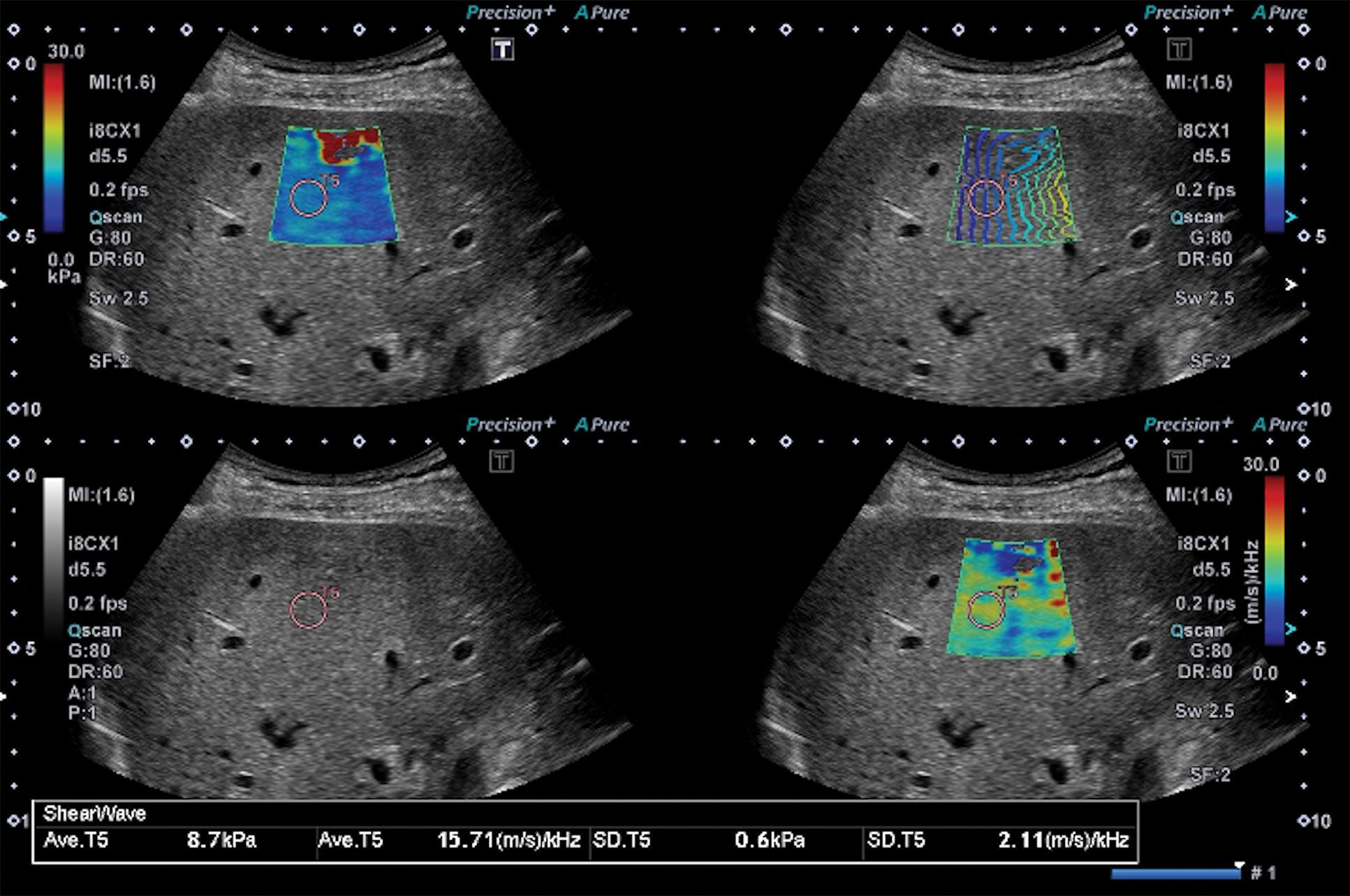
The details of the actual acquisition using the Aplio i-series are as follows. SWD can be activated automatically in shear wave elastography (SWE) mode. The dispersion map shows the dispersion slope, which is a parameter directly related to viscosity. The calculated dispersion slope value ([m/s]/kHz) and its standard deviation are displayed. In SWE quad view mode, SWS or stiffness (speed map, elasticity map), SW arrival time contours (propagation map), gray scale, and dispersion slope (dispersion map) can be displayed simultaneously ( Fig. 12.3 ).
Become a Clinical Tree membership for Full access and enjoy Unlimited articles
If you are a member. Log in here