Physical Address
304 North Cardinal St.
Dorchester Center, MA 02124
Based on a previous chapter by Karl Schulze, MD.
This chapter is an updated revision of Dr. Kurt Brück’s comprehensive treatise on neonatal thermal regulation presented in earlier editions; it is not an original synthesis by the current author. Dr. Brück remains the senior contributor despite his death in 1995 in recognition of and with immense admiration for his many contributions to our understanding of the physiology of human neonates. His classic study of temperature regulation provided fundamental knowledge that was validated and refined in subsequent years. Using continuous measurements of heat production and cutaneous blood flow, before, during, and after discrete and timed environmental cold stress, Brück defined the fundamental features of the neonatal response to chilling and its dependence on gestational and postnatal ages. These important observations concerning the basic responses of infants to cold—increased heat production and decreased heat loss, as well as the timing of these events and their development pattern—remain central to our understanding of how best to care for human neonates.
It is useful to model the temperature control system of newborn infants using the basic biocybernetic concept of a passive (i.e., controlled) system, the temperature of which depends on metabolic heat production and heat transfer to the environment. The controller consists of sensor, integrator, and effector components ( Fig. 42.1 ). Current understanding is that various central and peripheral thermal sensors monitor information about heat storage and heat gradients within the body continuously. These multiple inputs are transmitted to an integrating neural control network, located in the hypothalamus and limbic systems, which, in turn, is linked by means of efferent neuronal pathways to effector mechanisms that are capable of controlling heat storage. The earlier concept that the controlled variable is a single temperature at a single site within the body has been refined to take into account evidence that temperatures at multiple sites provide important input to the controller.
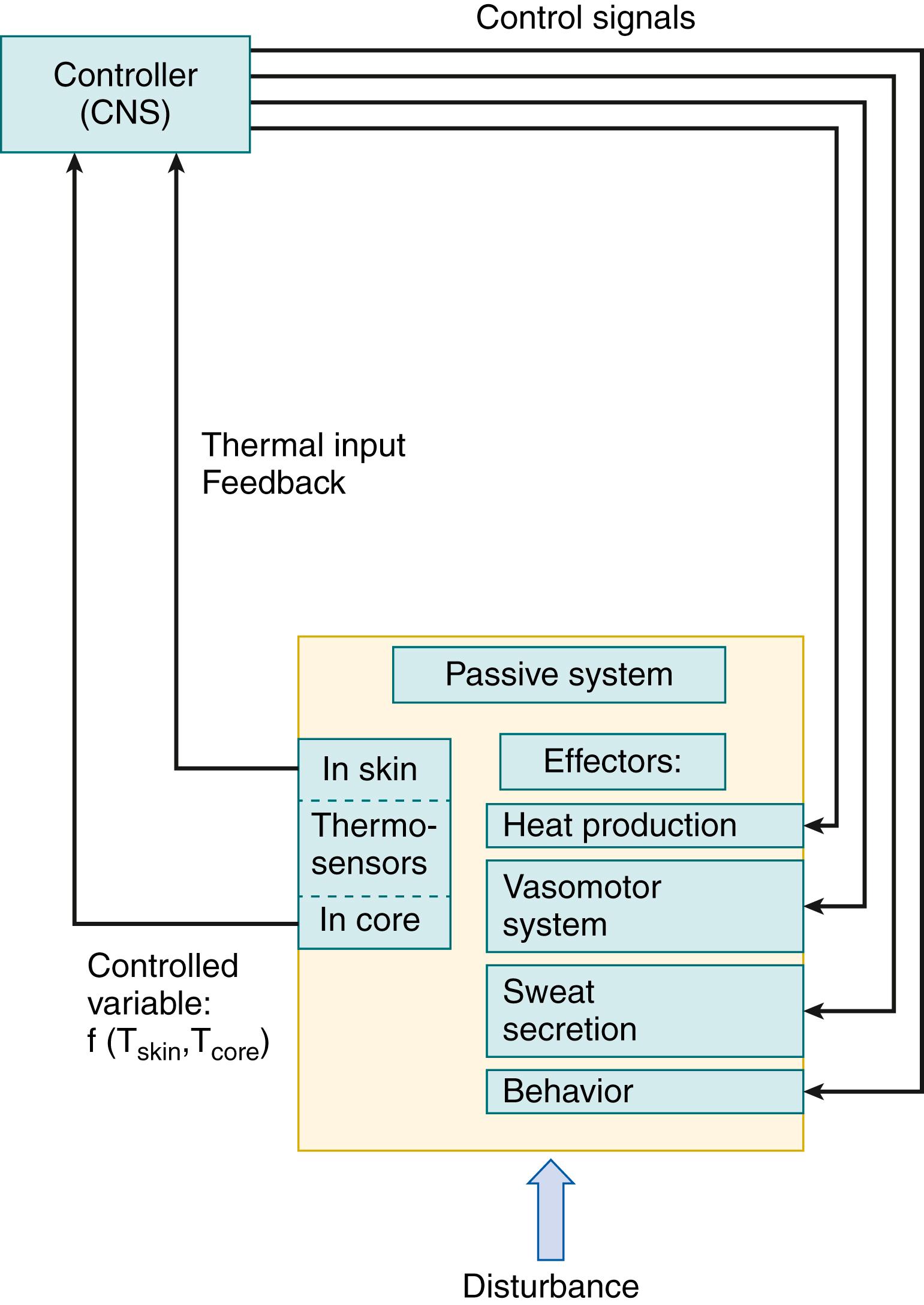
For clarity, the following discussion is structured according to this controller model, reviewing information about the sensors, integrator, and effectors as separate components followed by a consideration of how the integrated control system functions in response to environmental thermal transients, how the system changes during development, and how it operates under special circumstances, such as fever, heart failure, and hypoxia.
The maintenance of a stable body temperature that is much warmer than the environmental temperature is a property of the two higher classes of the animal kingdom: birds and mammals. These classes form the group of homeothermic beings; all others are designated as poikilothermic . A prerequisite of homeothermy is a basal rate of metabolism several times higher than that in poikilothermic animals. The former are thus referred to as tachymetabolic and the latter as bradymetabolic organisms. Furthermore, homeothermy requires a balance among heat production, skin blood flow, sweating, and respiration in such a way that changes in heat loss or gain from the environment are precisely compensated.
Metabolic processes that provide energy for maintenance of homeostasis and physical exercise are closely linked with heat production. The overall efficiency of energy transformation in homeotherms is only on the order of 10% to 25%, meaning that most of the energy transformed during metabolic activities is liberated as heat and must either be eliminated or stored depending on the needs of the organism. For organisms that are tachymetabolic (including human neonates), the metabolic rate at rest is sufficient to increase body temperature by several degrees Celsius greater than the ambient temperature. The metabolic rate at rest is of great importance for the state of the controlled system.
The at-rest metabolic rate depends on several factors, including physical activity, environmental temperature, feeding, thermic effect of food, diet-induced thermogenesis (formerly called specific dynamic action ), time of day (diurnal rhythmicity), age, and growth rate. By convention, the conditions used for the measurement of at-rest metabolic rate have been standardized: (1) subjects must be awake and have fasted at least 12 hours; (2) they must be fully relaxed; and (3) thermoneutral conditions should be maintained.
Metabolic rate measured under these standard conditions is termed the basal metabolic rate (BMR). Neonates are unlikely to fulfill the first and second standard conditions at the same time; thus, special conditions for measurement must be defined. The following conditions have been suggested :
The infant should remain on a normal feeding schedule (this suggestion is not unreasonable because the maximum increase in energy metabolism in infants is only 4% to 10% after an ordinary feeding).
The measurements must be made in a period of 5 to 10 minutes, during which the infant is fully relaxed (in contrast with the BMR standard conditions, the infant does not have to be awake). The metabolic rate determination may even be made during postprandial sleep.
The measurements must be made under thermoneutral conditions.
The minimum metabolic rate measured in this way is called the standard metabolic rate (SMR) or minimum observed metabolic rate . The designation BMR should be used only for determinations performed under standard conditions as employed in adults. Depending on why the metabolic rate is being measured, longer periods of observation (i.e., 3 to 6 hours) are required. ,
Total heat production is related to body size; for example, the overall metabolic rate of sheep is greater than that of rabbits. Because body temperatures of homeothermic species are close to one another at similar preferred environmental temperatures, it can also be anticipated that the metabolic rate per kilogram of body mass will be larger in a rabbit than in a sheep. In fact, there is a close correlation between the logarithm of body weight and the logarithm of at-rest metabolic rate (oxygen consumption) with a slope of 0.75 for adult animals of various sizes. This means that the metabolic rate per (kilogram body weight) ¾ of at-rest animals of different sizes, including adult humans, is independent of body size. This relationship has been termed the law of metabolic reduction by Kleiber and has been found useful by some observers for cross-species comparisons of the physiology of metabolism.
Although the Kleiber equation can be useful for predicting metabolic rates when comparing different species, attempts to predict metabolic rates within a group of individual organisms of the same species but of different body sizes reveals its limited applicability. Several deviations from the law of metabolic reduction must be introduced. Age, sex, body shape, and body composition affect metabolic rate. The age dependency of metabolic rate makes it impossible to predict the metabolic rates of neonates and adults from an individual species with a single exponential function of weight, even if a higher exponent than Kleiber’s is used.
As illustrated in Fig. 42.2 , newborn infants to 1 week of age have an SMR that is lower than that predicted by Kleiber’s equation—that is, the SMR is lower in a 3-kg human newborn than in a 3-kg rabbit. Conversely, in the weight range of 5 to 20 kg, the SMR is higher in human infants than in adult animals of the same weight. During this period of growth, the SMR demonstrates a nearly linear relationship to body weight. Mathematically, this relationship can be expressed by an exponent of b = 1 in the equation SMR = a × m b where a is age and m is mass. During the period that corresponds to the weight range of 20 to 70 kg, the SMR approaches data in Kleiber’s curve.
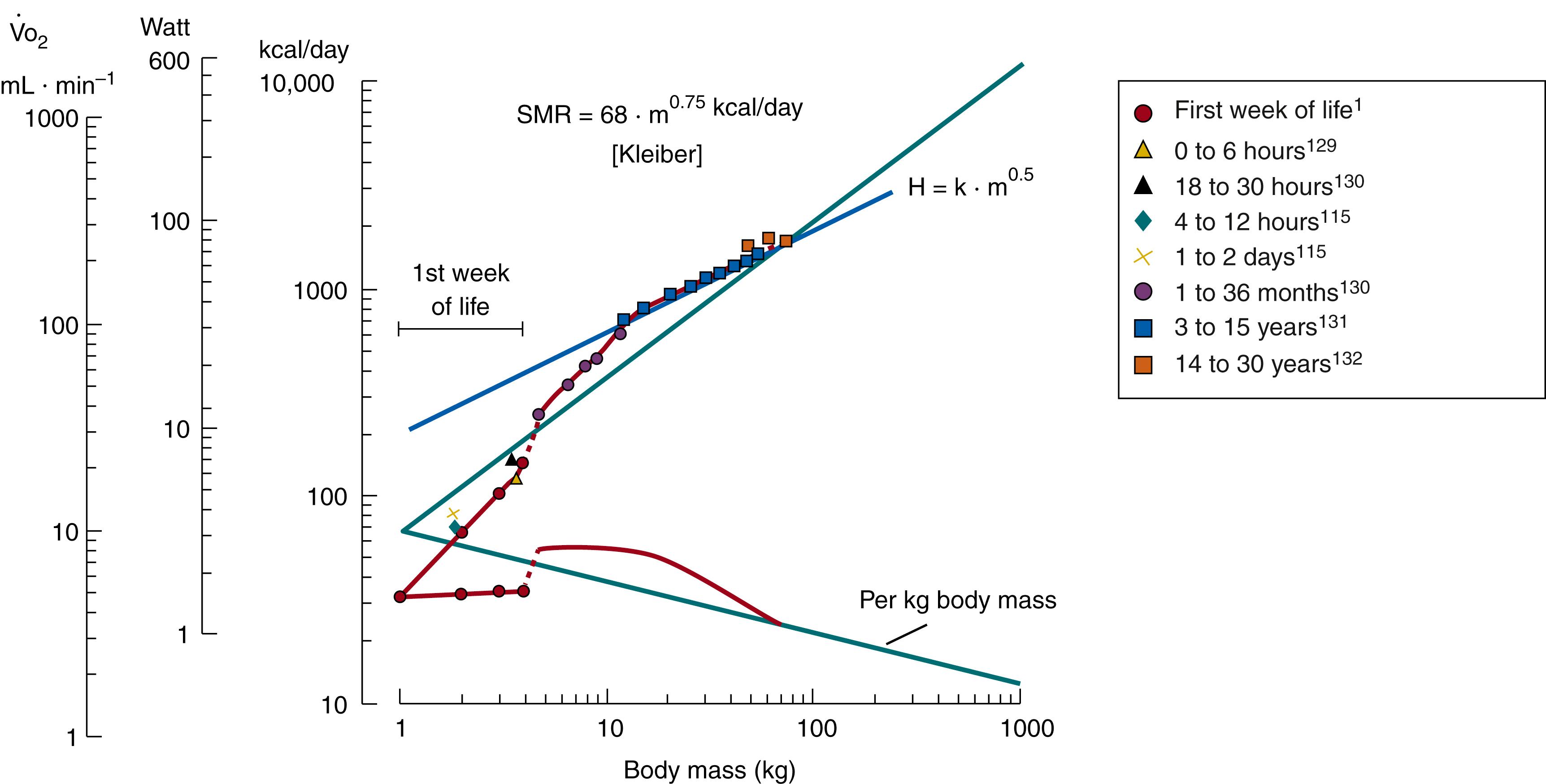
An exponent close to 1, or somewhat larger than 1, should also be used to calculate the SMR for infants weighing between 1 and 4 kg. This means that during the neonatal period, the SMR/body mass unit is almost independent of weight, whereas a decrease would be expected with increasing weight according to Kleiber’s equation (see Fig. 42.2 ). These SMR values demonstrate a relatively large amount of scatter during the first day of life. A survey of these data and a discussion of the possible reasons for the scattering may be found elsewhere. , Given these limitations, attempts to refer observations of the metabolism of human neonates to standards derived for other mammals should be avoided.
Certain quantitative changes in the SMR during early postnatal development are worth keeping in mind:
Immediately after birth, the SMR/kg body mass is lower in human infants than in adult animals of the same weight (see Fig. 42.2 ).
After birth, the SMR/kg body weight increases and eventually attains a value that is up to 50% higher in infants than in adult animals of the same body mass. The time course of this process appears to be variable. It may take 2 days to a few weeks (particularly in premature infants) to attain values that are characteristic of the post-neonatal period. ,
The seeming violation of the law of metabolic reduction (see Fig. 42.2 ) during the early neonatal period may be only a fictitious problem if one takes into account the considerable change in extracellular water content (extracellular fluid [ECF]) that occurs from the early fetal to the adult stage. Sinclair and colleagues have suggested using body weight minus ECF as the reference value in metabolic reference standards. According to their calculations, ECF composes as much as 44% of body weight in a 4000-g newborn infant and 58% in a 1000-g premature neonate. The corresponding average value for ECF in the adult is only 20%. Because the ECF does not participate in oxidative metabolism, the expression “body weight—ECF” may be considered representative of the active tissue mass. Thus, it seems theoretically justified to relate metabolic rate to this active tissue mass rather than to total body weight. In contrast, the lean body mass (i.e., total body mass—fat mass) has not been found to be a suitable reference value.
One can also relate metabolic rate in a neonate to the expression “active tissue mass + adult ECF”—that is, to a body mass that corresponds in composition to that of the adult organism. Metabolic rates related to this calculated value closely approximate Kleiber’s curve, whereas the metabolic rate related to the actual body weight remains less than the predicted values.
All chemical reactions in an organism are temperature dependent and obey the van’t Hoff law. According to this law, oxygen uptake and body temperature of a poikilothermic animal are expected to decrease with decreasing environmental temperature. This decrease has, in fact, been demonstrated in frogs, reptiles, and other poikilothermic animals without exception. The ratio of the reaction rates at temperatures differing by 10°C is called the Q 10 . In general, the Q 10 for the metabolic rate in poikilothermics is approximately 2 to 3. van’t Hoff’s law applies to homeotherms in the same way but is masked by regulatory processes.
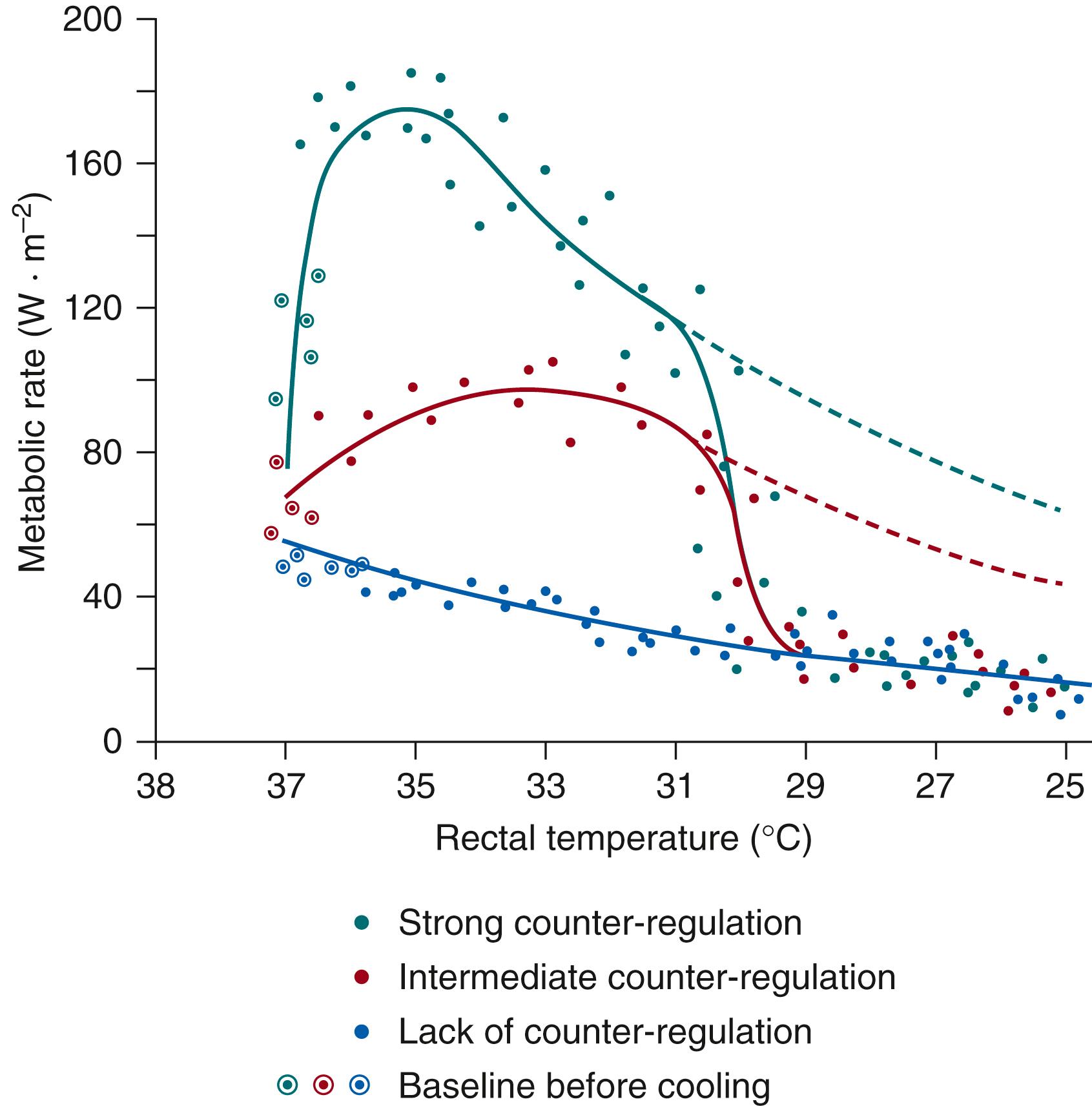
Thus, in the intact homeothermic organism, the metabolic rate increases initially with decreasing body temperature ( Fig. 42.3 , top curve ). This regulatory metabolic rise can be blunted or prevented by pharmacologic intervention, in particular by moderate or deep anesthesia (see Fig. 42.3 , middle and bottom curves ). In fully anesthetized dogs, metabolic rate decreases with decreasing body temperature (bottom curve) , obeying van’t Hoff’s law. The slope of the curve corresponds to a Q 10 of 2 to 3. At body temperatures below 30°C, the metabolic rate drops steeply to the basal curve ( solid lines in Fig. 42.3 ) because the thermoregulatory drive generated in the thermointegrative areas of the central nervous system vanishes with increasing hypothermia. The body temperature at which this reduction occurs may be species dependent.
Before describing the response of the integrated system to changes in environmental temperature, we review what is known about the individual components of the biocybernetic model.
The cutaneous thermal receptors are a group of structures that function as temperature sensors in the temperature control system (the term sensor is gaining preference to receptor to avoid confusion with chemical structures reacting with specific substances; both terms are used interchangeably within this section). It is generally agreed that the cutaneous thermosensors in the thermoregulatory system are identical with those mediating thermal sensation. The distribution of the cutaneous thermoreceptors can thus be studied in adult humans by stimulation of so-called warm and cold spots using fine temperature probes. There is scarcely any area of the skin that does not respond to cold stimulation, although the number of cold spots/cm 2 may vary between 1 and 5 on the palm of the hand and more than 15 on the face. The number of warm spots/cm 2 is much less in all skin areas (0.3 to 1.7). This agrees with the better spatial resolution of cold stimuli, but it does not mean that cutaneous warm sensitivity is any less developed than is cold sensitivity. In fact, with larger stimulus areas (using thermodes of 10- to 100-cm contact areas), warm sensitivity can be demonstrated on all skin areas with few exceptions (cornea, glans penis). Employing electrophysiologic methods (recording single-receptor activity from nerves), warm and cold receptors have been demonstrated on the faces of cats and other species and on the lower arms and legs of monkeys. By inserting fine metal electrodes through the intact skin into a branch of the radial nerve, it has even been possible to record the activity of single warm and cold receptors in humans in response to thermal stimulation on the skin of the back of the hand.
The morphologic correlate of cold sensors is the fine unmyelinated nerve endings penetrating into the basal layer of the epidermis. These endings contain numerous mitochondria, providing energy for a temperature-sensitive Na + , K + pump, which seems to be part of the transduction of the cold stimulus into an electrical signal.
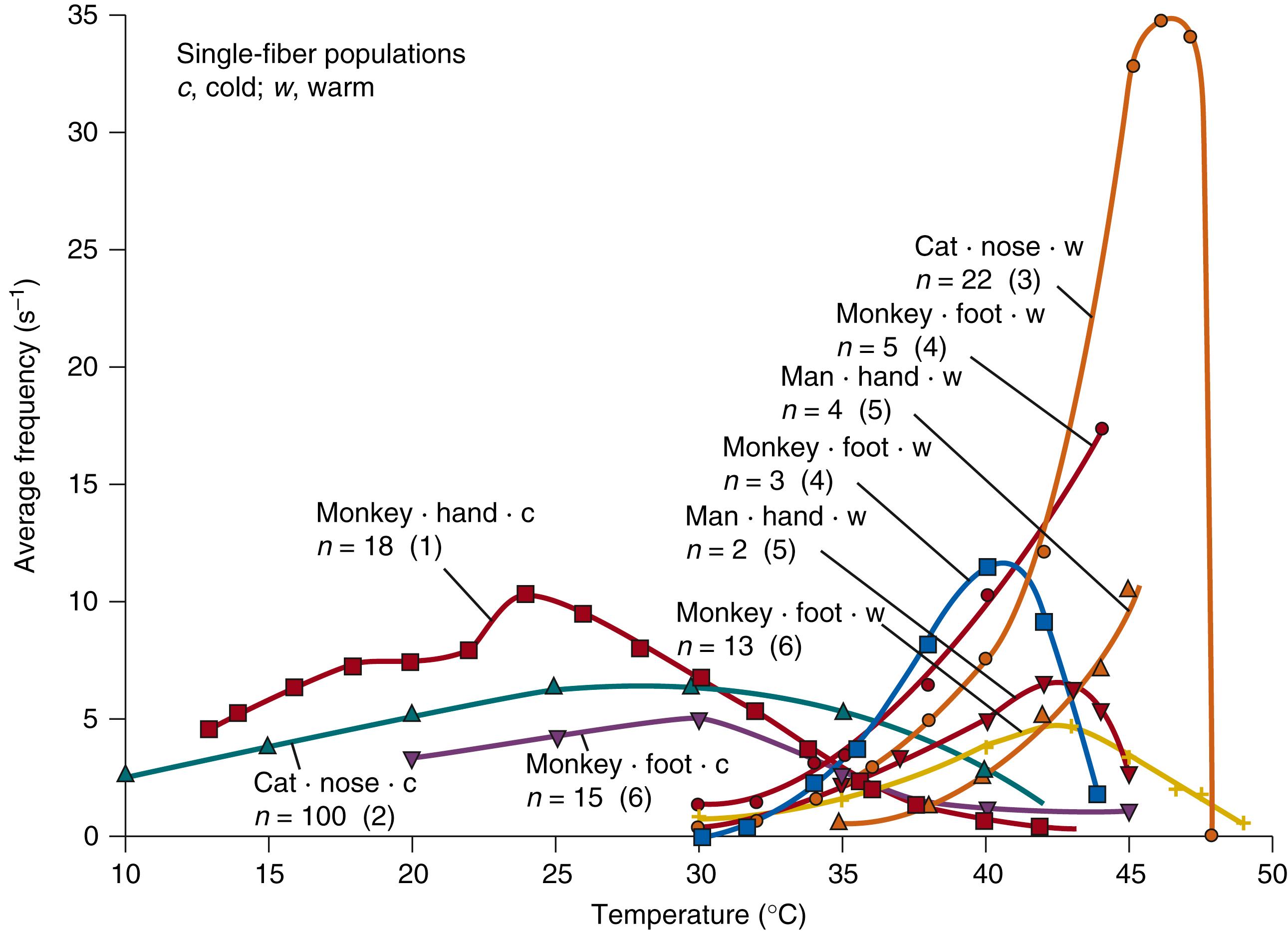
Fig. 42.4 illustrates the average response characteristics of cold and warm receptors from different species (including humans) under static conditions (i.e., after the temperature of the skin had been constant for several minutes). Minimum activity occurs at a skin temperature of 35°C. The maximum discharge frequency of cold receptors is found between 35°C and 20°C, and that of warm receptors takes place between 40°C and 45°C. At temperatures above 45°C, warm-receptor activity decreases. At temperatures lower than approximately 25°C, the activity of cold receptors may also be reduced.
Sensor activity during temperature changes is noteworthy in that receptor discharges may reach frequencies that are two- to three-fold greater than under static conditions. Irrespective of the initial temperature, a warm receptor will always show an overshoot of its discharge on sudden warming and a transient inhibition on cooling, whereas a cold receptor will respond in the opposite way (with an inhibition on warming and an overshoot on cooling). Correspondingly, sensitivity to temperature changes is amplified and increases with the size of the exposed area. With exposure of larger areas (e.g., the whole hand), a temperature change of less than 0.01°C/s may evoke a thermal sensation and probably a regulatory reaction.
In summary, thermoregulatory responses elicited through cutaneous thermoreceptors are determined by the average skin temperature (T skin ) , the rate and direction of temperature change (ΔTskin/Δt), and the size of the stimulated area. , Because of the temperature discharge characteristics (see Fig. 42.4 ), warm receptors may contribute to the stimulation of heat dissipation actions only when mean skin temperature is considerably higher than normal levels.
Evidence for the existence of deep body thermosensors has been obtained in three ways: (1) by demonstrating that there is poor correlation between body temperature and the action of the final control elements if only skin temperature is taken into consideration, (2) by observing thermoregulatory actions after heating and cooling of circumscribed areas within the body using implanted thermodes and vascular heat exchangers, and (3) by recording the activity of single units of the central nervous system and relating these responses to their own local temperatures.
According to thermal stimulation studies (using thermodes implanted on a long-term basis), deep body thermosensors are located in the preoptic area and the anterior hypothalamus. Local warming of this region stimulates heat-dissipating mechanisms (i.e., vasodilation, panting, sweating), whereas it inhibits heat production (i.e., metabolic reactions [ Fig. 42.5 ] and vasoconstriction). Inversely, cooling evokes heat production and vasoconstriction, whereas heat dissipation mechanisms are inhibited.
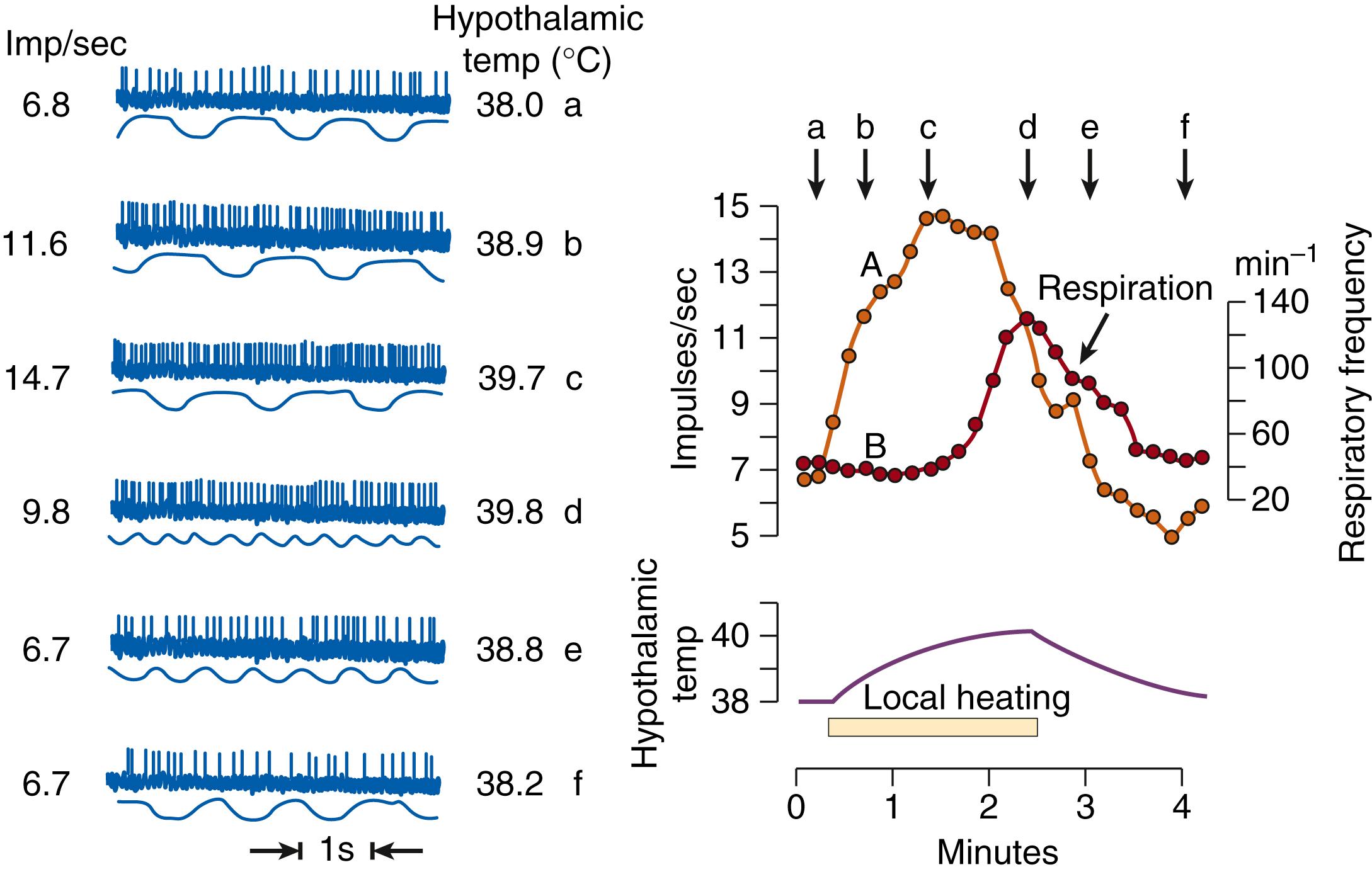
Thermal stimulation does not permit discrimination between internal cold and warm receptors (e.g., the effects of local cooling might be ascribed to actuation of central cold receptors and to the inhibition of central warm receptors). Here further clarification is obtained from single-unit studies. As shown in Fig. 42.6 , warming of the preoptic area results in markedly increased activity of one single unit and leads to an increase in respiratory frequency (panting). Units like this are considered to be warm sensors.
In vitro studies of hypothalamic slices and of cell cultures have addressed the question of whether hypothalamic thermosensitivity is tied to individual thermosensitive ganglion cells or is based on the temperature dependence of synaptic transmission between afferent and efferent neurons ( Fig. 42.7 ). After synaptic transmission is blocked by electrolyte solutions with a low Ca 2+ content and a high Mg 2+ content, the structures in question remain sensitive to thermal stimuli. This implies the existence of thermosensitive cells within the preoptic region and anterior hypothalamus. Both areas contain not only warm-sensitive cells but also thermosensitive and cold-sensitive cells, the latter two being less numerous than the warm-sensitive cells.

Thermosensitive structures have also been demonstrated in the lower brain stem (midbrain and medulla oblongata), and thermoregulatory reactions can be initiated by local warming of these areas. However, the thermosensitivity of this region is distinctly less than in the preoptic region and anterior hypothalamus. In contrast, the spinal cord is extremely thermosensitive. When the temperature of the spinal cord is raised only a few tenths of a degree along its entire length in dogs and other animals, the results include panting, vasodilation, and inhibition of thermogenesis. , Cooling of the spinal cord elicits shivering, but in this case a greater temperature change is required. In newborn and young guinea pigs, a local temperature change in the region of the cervical cord suffices to trigger thermoregulatory reactions.
Quantitative considerations suggest that thermoreceptive structures exist outside the central nervous system and the skin. Experimental evidence for the presence of thermosensors in the region of the dorsal wall of the abdominal cavity and in the musculature exists. Evidence for the existence of subcutaneous thermosensors has also been confirmed.
The cutaneous thermoreceptors are served by thin myelinated and unmyelinated axons belonging to the slowly conducting group III and group IV nerves. Warm fibers are mostly unmyelinated (group IV). The axons run within the afferent cutaneous nerve bundles, and they enter the spinal cord through the segmental dorsal root ganglia. Those axons cross over to the contralateral side and ascend within the spinothalamic tract in the anterolateral section of the spinal cord. On their way to the thalamus, the ascending thermal fibers join the medial lemniscus and are accompanied by the afferents coming from the trigeminal region. From the medial lemniscus, collaterals diverge and project to the hypothalamus through a pathway not definitively described. Evidence has been obtained that part of the cutaneous thermal input is conveyed through the spinoreticular pathway to the reticular formation; from there, it is projected to the hypothalamus through the raphe nuclei and the ventral noradrenergic system, which passes the subcerulean area. ,
The spinal cord thermal sensors are connected to the posterior hypothalamus through axons running in an anterolateral pathway of the spinal cord, as has been shown in young guinea pigs and cats. , The thermosensors of the preoptic area also end in the posterior hypothalamus; however, these short pathways have not yet been identified.
The theoretic concepts of thermoregulation require the demonstration of some elements that “process” the thermal information originating at the receptors in various sites of the body (multiple-input system) and that transform these inputs from the sensors into effector outputs.
There are many experimental studies implicating the hypothalamus (especially the posterior hypothalamic area, which has no appreciable thermosensitivity) as an integration center for thermoregulation. This premise is further supported by electrophysiologic findings. For example, neurons exist in the posterior hypothalamic area, the activity of which (discharge rate) is influenced by local thermal stimulation in either the preoptic region or the cervicothoracic part of the spinal cord. At the boundary between the anterior and posterior hypothalamus, neurons have been found that respond to changes in the skin temperature on the limbs and trunk. The posterior hypothalamus, therefore, is characterized by the presence of thermoresponsive cells (i.e., cells that respond to changes in the temperature of distant structures but are not sensitive to changes in their own temperature). However, there is no absolute spatial separation of receptive and integrative functions. In the preoptic region and anterior hypothalamus, cells shown to be thermosensitive have also been found to be affected by skin temperature changes and thus are simultaneously thermoresponsive. ,
A special feature of biologic thermoregulation (compared with the familiar simple technical system) is that two kinds of sensors in different locations (the cold and warm receptors) interact antagonistically. The cutaneous cold receptors are activated when the temperature decreases to less than the lower limit of the thermoneutral zone. This reaction is counteracted by heat-activated internal thermosensors when the body temperature increases as a result of overshooting cold-protective mechanisms or after bodily activity. This circuitry enables the protective mechanisms to be set in motion rapidly in case of external cooling, long before the core temperature has begun to decrease and internal thermoreceptors can be influenced.
Conversely, heat dissipation processes (vasodilation, sweating) may be activated by internal warm receptors, which are primarily stimulated when body core temperature increases. This effect is counteracted by cold activation of the cutaneous cold receptors. When the body is heated externally, sweat secretion is stimulated by the combined action of cutaneous and internal warm receptors.
Fig. 42.8 shows a simplified model of the neuronal circuitry underlying the central nervous system integrative processes. Three kinds of neuronal elements are distinguished: (1) efferent neurons located in the hypothalamus, the axons of which activate the peripheral controlling elements ( Fig. 42.9 ) either directly or, more probably, by way of a chain of interneurons; (2) facilitative and inhibitory interneurons within the hypothalamus; and (3) thermal afferents, arising in part from the cutaneous thermoreceptors and in part from internal receptors (e.g., those of the preoptic region).
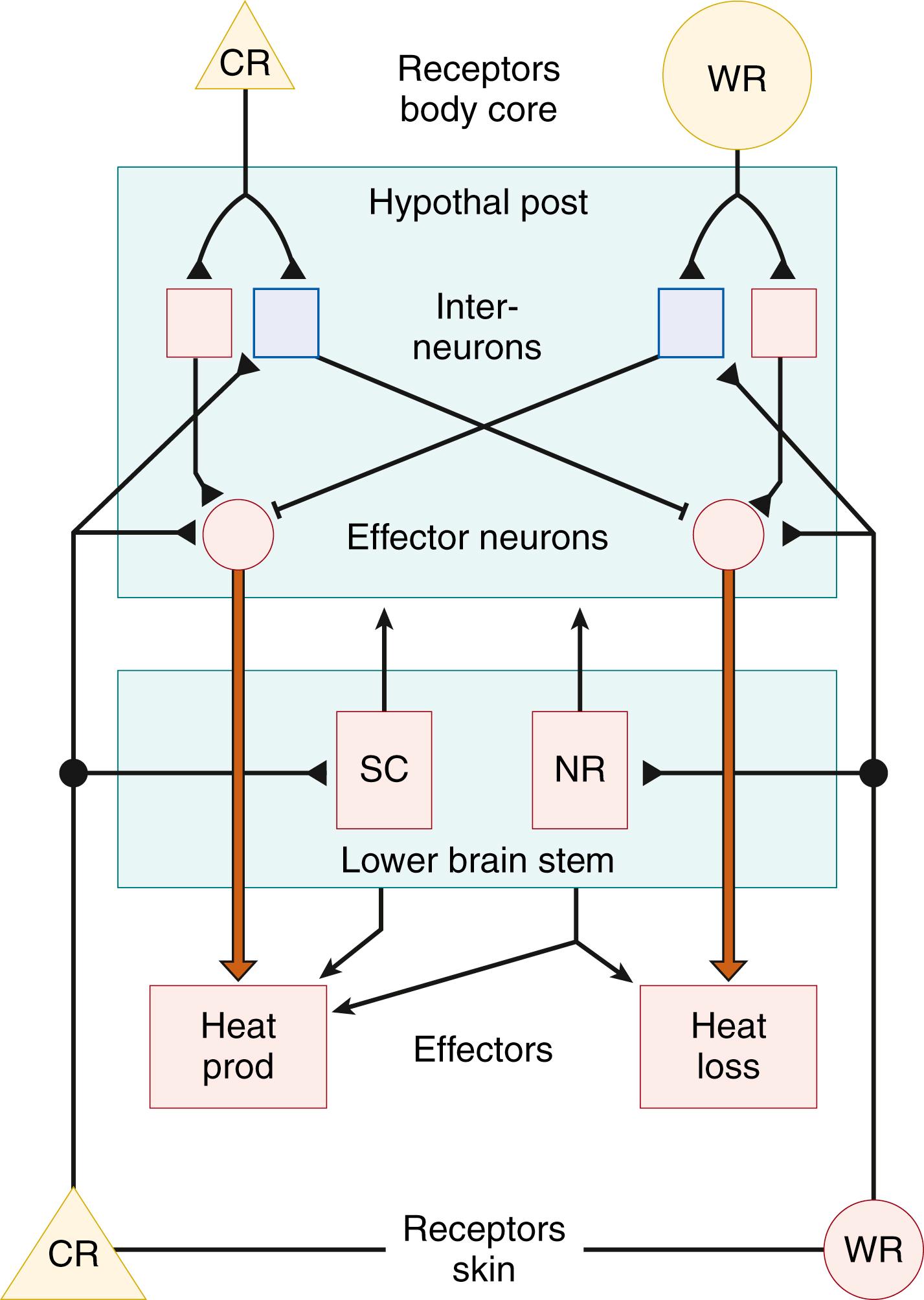
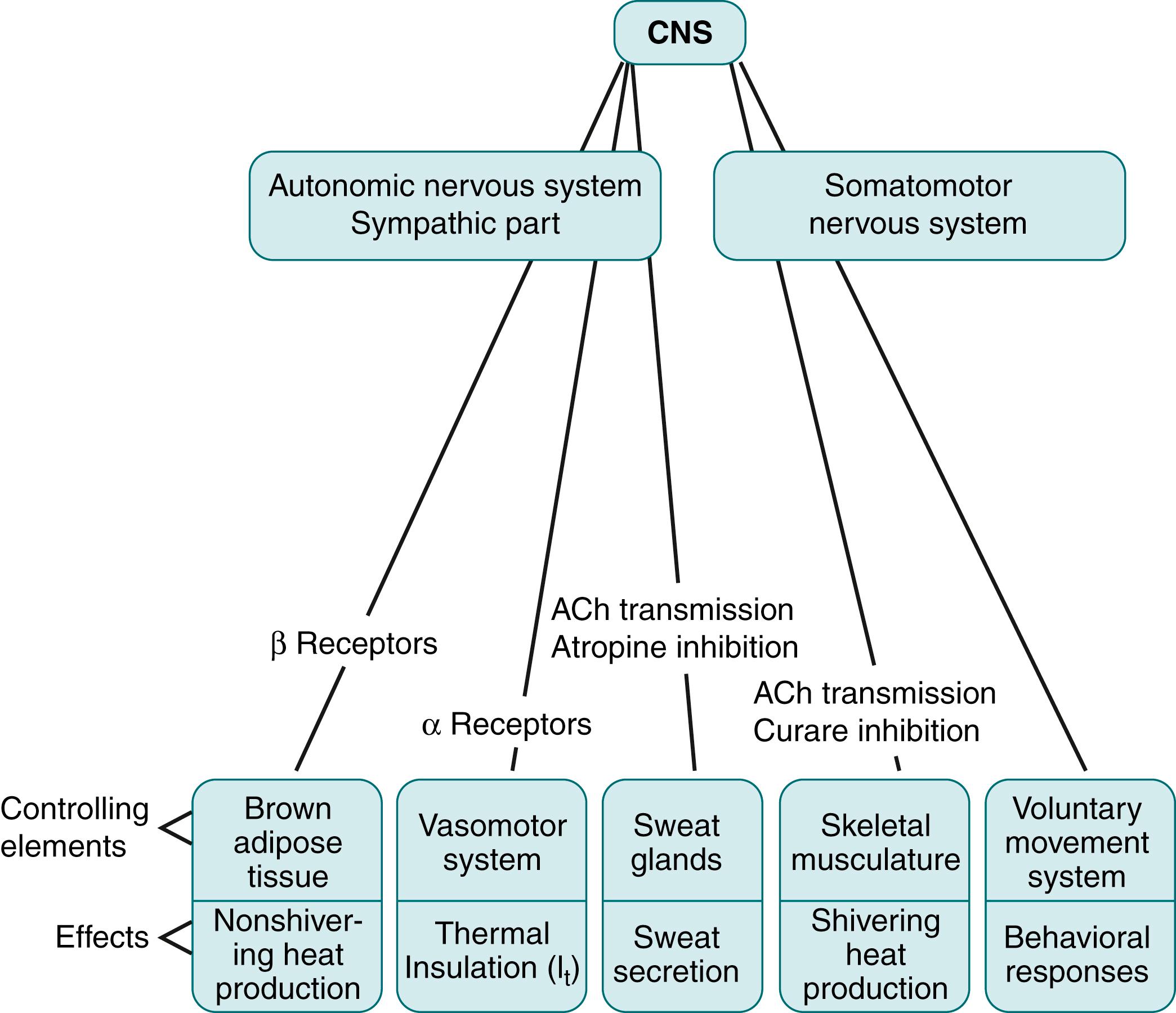
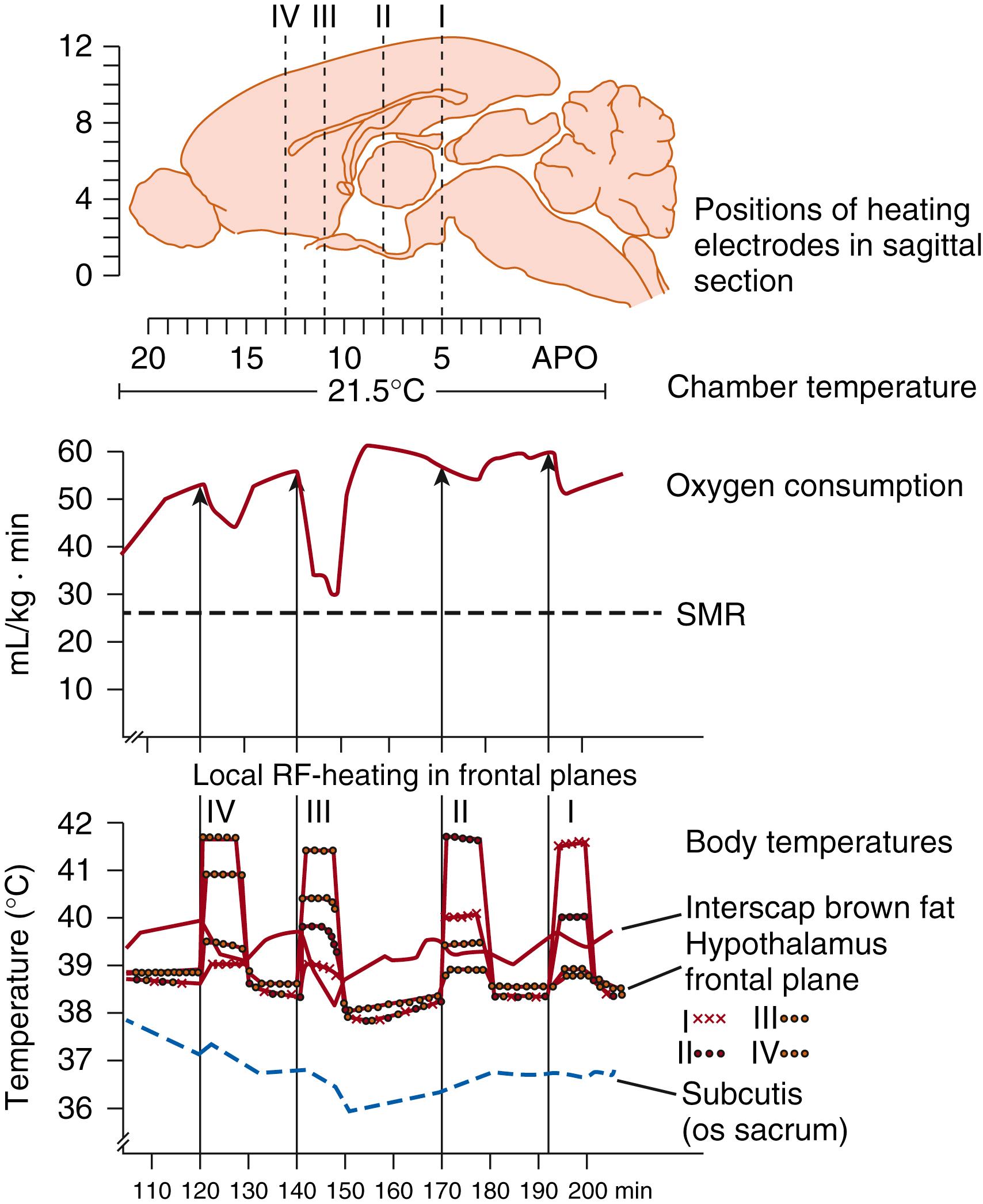
Cold receptors directly activate the effectors for thermogenesis. The inhibitory action on the efferents to heat loss effectors is exerted through interneurons. Activation of warm receptors excites the efferents to the heat loss effectors, simultaneously inhibiting (through interneurons) the efferents to the effectors for heat production. The various effector neurons may receive different combinations of thermal afferents. Thus, as shown in studies of newborn guinea pigs, nonshivering thermogenesis is driven by cutaneous cold receptors and is inhibited by hypothalamic warm receptors, whereas the inhibitory influence of shivering is exerted mainly by spinal cord warm-receptive structures ( Fig. 42.10 ). The cervical spinal cord is the region that preferentially receives (through vascular connections) the heat that is generated in the interscapular brown adipose tissue (BAT). , , As a result of this “meshed control” of the two heat-generating mechanisms, shivering remains suppressed in neonates so long as sufficient heat is supplied from the interscapular BAT to the spinal cord warm-sensitive structures and intrathoracic organs.
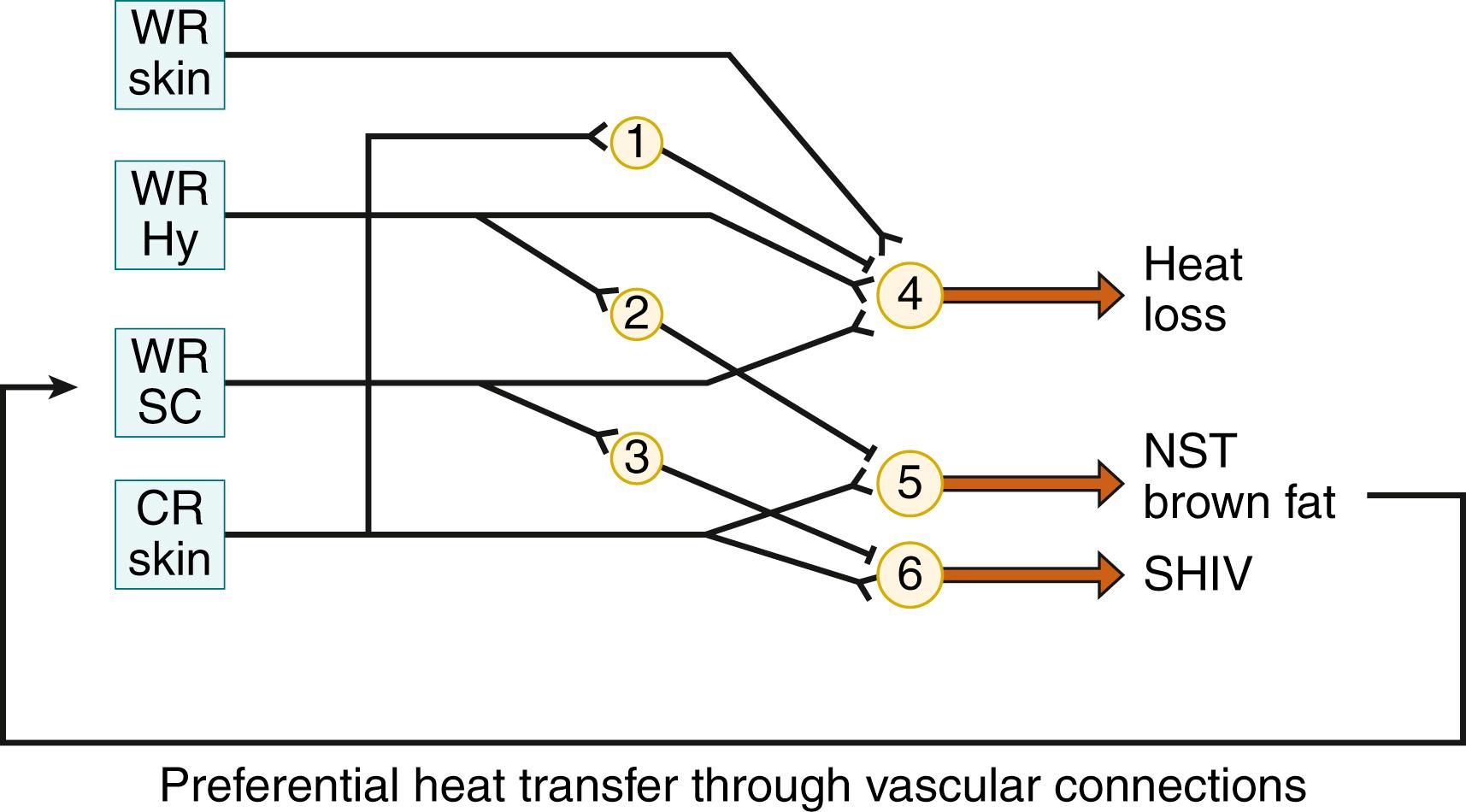
Given that the thermosensitive structures are distributed over the entire body, the thermoregulatory effector actions (see Fig. 42.9 ) cannot be described as a function of a single local temperature (e.g., the rectal temperature). Thus, the goal of thermophysiology is to describe thermoregulatory actions as a function of as many as possible of the temperatures associated with various thermosensitive parts of the body. Systems of equations with several variables are required for such a description. The data must be collected by experiments on animals in which spatially circumscribed temperature changes are produced by means of thermodes and heat exchangers ; in humans, limited opportunity exists for experimental manipulation of local temperature while the temperature of the rest of the body is kept constant. Only an approximate description is possible; the thermoregulatory parameters are presented as a function of two temperatures, the temperature of the interior of the body (measured at a representative site) and the mean skin temperature. , , Shivering and sweating threshold temperatures, as well as the temperature pairs yielding equal magnitudes of shivering and sweating, are represented by contour lines in a coordinate system with mean skin temperature and core temperature as the coordinates ( Fig. 42.11 ). Blood flow through the skin follows similar contour lines located between shivering and sweating threshold lines. The contour plots portrayed in Figs. 42.11 and 42.12 , although involving only two temperatures, are good semiquantitative illustrations of the performance of the multiple-input control system. Note that the relative impact of the sensors increases as temperatures become increasingly “out of range,” and that the whole contour plot depends on other attributes such as the warm/cold adapted state of the animal. Within a certain range of temperatures (i.e., near the set point), at which the contour lines can be approximated by straight lines, it is possible to express the effector responses and the threshold temperature conditions for the elicitation of effector responses in terms of a weighted mean body temperature, T b , calculated with the following linear equation:
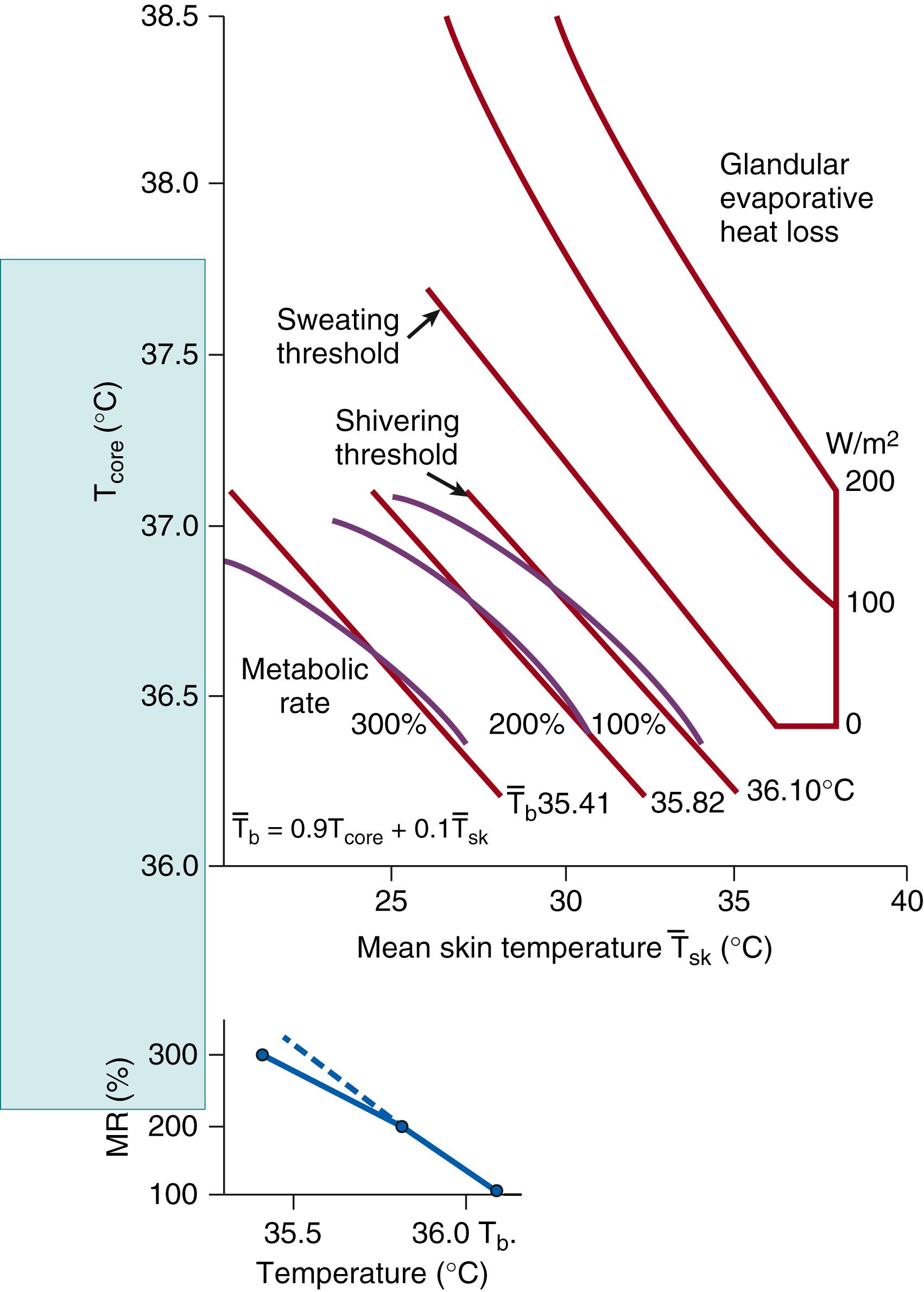
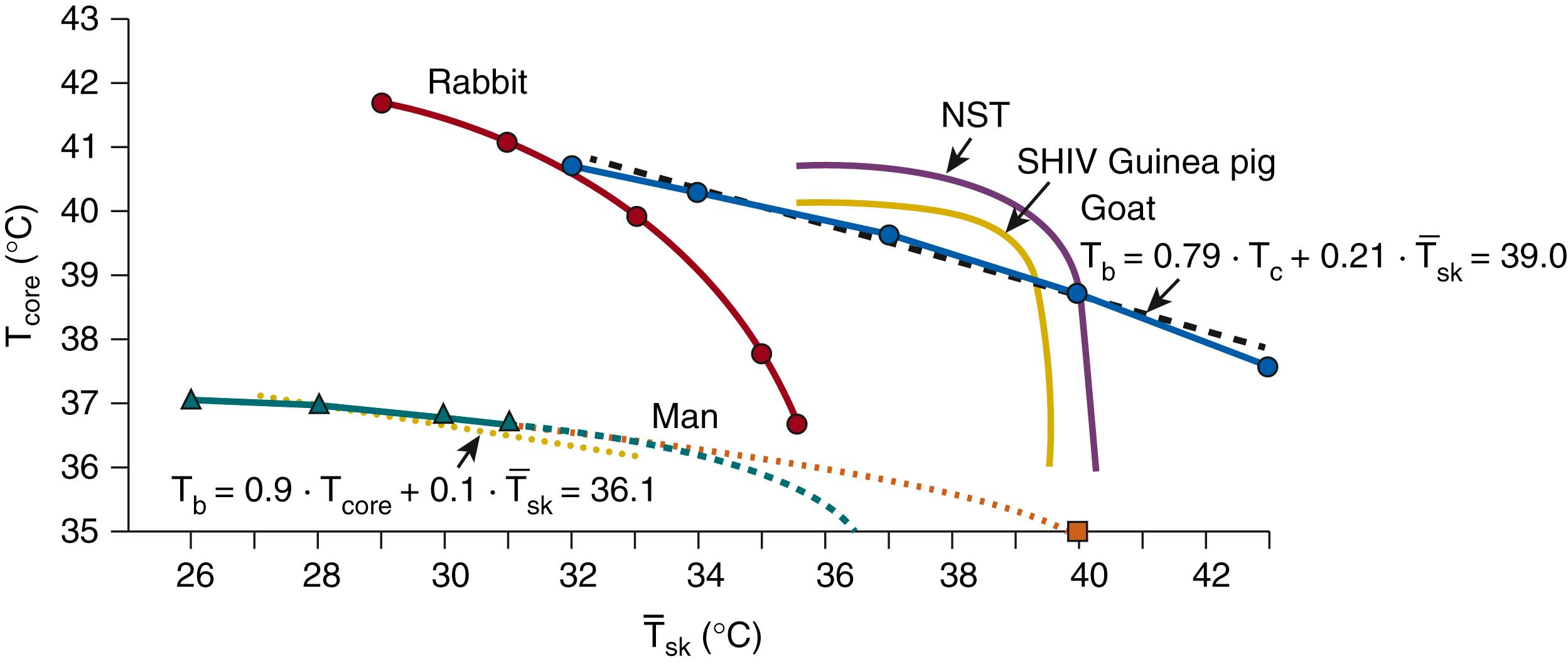
Data from human adults can be best fitted if values of 0.9 and 0.1 are chosen for the coefficients a and b , respectively. Fig. 42.12 shows the shivering threshold contour lines of adult humans, rabbits, goats, and young (4-week-old) guinea pigs. In addition, the threshold contour line for nonshivering thermogenesis in newborn guinea pigs is given. The curvilinear contour lines in small animals do not allow the use of arithmetic mean body temperature except for small sections of the temperature ranges that may be approximated by straight lines.
Become a Clinical Tree membership for Full access and enjoy Unlimited articles
If you are a member. Log in here