Physical Address

304 North Cardinal St.
Dorchester Center, MA 02124

Respiratory system resistance


Key points

  • Gas flow in the airway is a mixture of laminar and turbulent flow, becoming more laminar in smaller airways.

  • Respiratory system resistance is a combination of resistance to gas flow in the airways and resistance to deformation of tissues of both the lung and chest wall.

  • In smaller airways smooth muscle controls airway diameter under the influence of neural, humoral and cellular mechanisms.

  • The respiratory system can rapidly compensate for increases in either inspiratory or expiratory resistance.

Elastic resistance, which occurs when no gas is flowing, results from only two of the numerous causes of impedance to inflation of the lung (listed in Chapter 2 ). This chapter considers the remaining components, which together are referred to as nonelastic resistance or respiratory system resistance. Most nonelastic resistance is provided by frictional resistance to air flow and thoracic tissue deformation (both lung and chest wall), with small contributions from the inertia of gas and tissue and compression of intrathoracic gas. Unlike elastic resistance, work performed against nonelastic resistance is not stored as potential energy (and therefore recoverable), but is lost and dissipated as heat.

Physical principles of gas flow and resistance

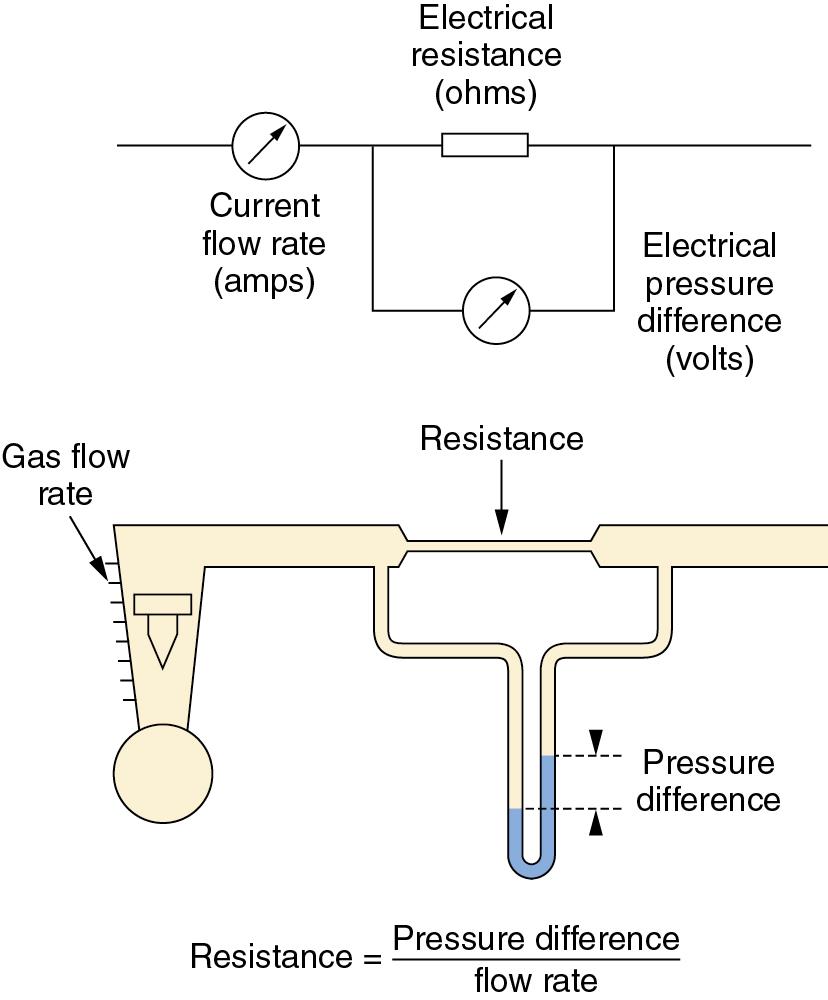
Gas flows from a region of high pressure to one of lower pressure. The rate at which it does so is a function of the pressure difference and the resistance to gas flow, analogous to the flow of an electrical current ( Fig. 3.1 ). The precise relationship between pressure difference and flow rate depends on the nature of the flow, which may be laminar, turbulent or a mixture of the two. It is useful to consider laminar and turbulent flow as two separate entities, but mixed patterns of flow usually occur in the respiratory tract. With a number of important caveats, similar basic considerations apply to the flow of liquids through tubes, which is considered in Chapter 6 .

• Fig. 3.1, Electrical analogy of gas flow. Resistance is pressure difference per unit flow rate. Resistance to gas flow is analogous to electrical resistance (provided that flow is laminar). Gas flow corresponds to electrical current (amps), gas pressure to potential difference (volts), gas flow resistance to electrical resistance (ohms), and Poiseuille’s law corresponds to Ohm’s law.

Laminar flow

With laminar flow, gas flows along a straight unbranched tube as a series of concentric cylinders that slide over one another, with the peripheral cylinder stationary and the central cylinder moving fastest, the advancing cone forming a parabola ( Fig. 3.2 , A ).

• Fig. 3.2, Laminar flow. (A) In laminar flow gas moves along a straight tube as a series of concentric cylinders of gas with the central cylinder moving fastest and the outside cylinder theoretically stationary. This gives rise to a ‘cone front’ of gas velocity across the tube. (B) The linear relationship between gas flow rate and pressure gradient. The slope of the lines indicates the resistance (1 Pa = 0.01 cmH 2 O).

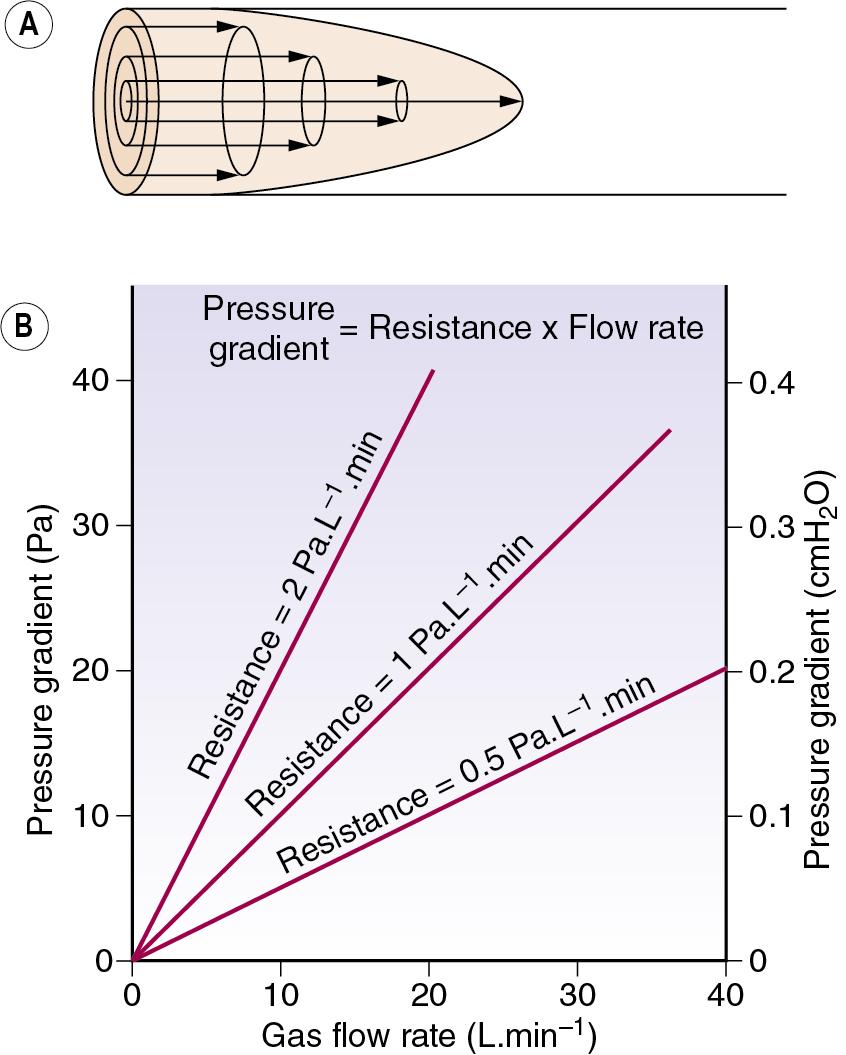
The advancing cone front means that some fresh gas will reach the end of a tube, but the volume entering the tube is still less than the volume of the tube. In the context of the respiratory tract, there may be significant alveolar ventilation when the tidal volume is less than the volume of the airways (the anatomical dead space), a fact that is very relevant to high-frequency ventilation (page 384). For the same reason, laminar flow is relatively inefficient for purging the contents of a tube.

In theory, gas adjacent to the tube wall is stationary, so friction between fluid and the tube wall is negligible. The physical characteristics of the airway or vessel wall should therefore not affect resistance to laminar flow. Similarly, the composition of gas sampled from the periphery of a tube during laminar flow may not be representative of the gas advancing down the centre of the tube. To complicate matters further, laminar flow requires a critical length of tubing before the characteristic advancing cone pattern can be established. This is known as the entrance length and is related to the diameter of the tube and the Reynolds number of the fluid (see later).

Quantitative relationships

With laminar flow, the gas flow rate is directly proportional to the pressure gradient along the tube ( Fig. 3.2 , B ); the constant is thus defined as resistance to gas flow:


ΔP = flow rate × resistance

where Δ P = pressure gradient.

In a straight unbranched tube, the Hagen–Poiseuille equation allows gas flow to be quantified


Flowrate = ΔP × π × ( radius ) 4 8 × length × viscosity

by combining these two equations:


Resistance = 8 × length × viscosity π × ( radius ) 4

In this equation, the fourth power of the radius of the tube explains the critical importance of narrowing of air passages. With constant tube dimensions, viscosity is the only property of a gas relevant under conditions of laminar flow. Helium has a low density but a viscosity close to that of air; therefore it will not improve gas flow if the flow is laminar (page 29).

In the Hagen–Poiseuille equation, the units must be coherent. In CGS units (see Appendix A ), dyn.cm −2 (pressure), mL.s −1 (flow) and cm (length and radius) are compatible with the unit of poise for viscosity (dyn.s.cm −2 ). In SI units, with pressure in kilopascals, the unit of viscosity is newton.s.m −2 . However, in practice it is still customary to express gas pressure in cmH 2 O and flow in L.s −1 . Resistance therefore continues to usually be expressed as cmH 2 O per litre per second (cmH 2 O.L −1 .s).

Turbulent flow

High flow rates, particularly through branched or irregular tubes, result in a breakdown of the orderly flow of gas described earlier. An irregular movement is superimposed on the general progression along the tube ( Fig. 3.3 , A ), with a square front replacing the cone front of laminar flow. Turbulent flow is almost invariably present when high resistance to gas flow is a problem.

• Fig. 3.3, Turbulent flow. (A) Four circumstances under which gas flow tends to be turbulent. (B) The square law relationship between gas flow rate and pressure gradient when flow is turbulent. Note that the value for ‘resistance,’ calculated as for laminar flow, is quite meaningless during turbulent flow.

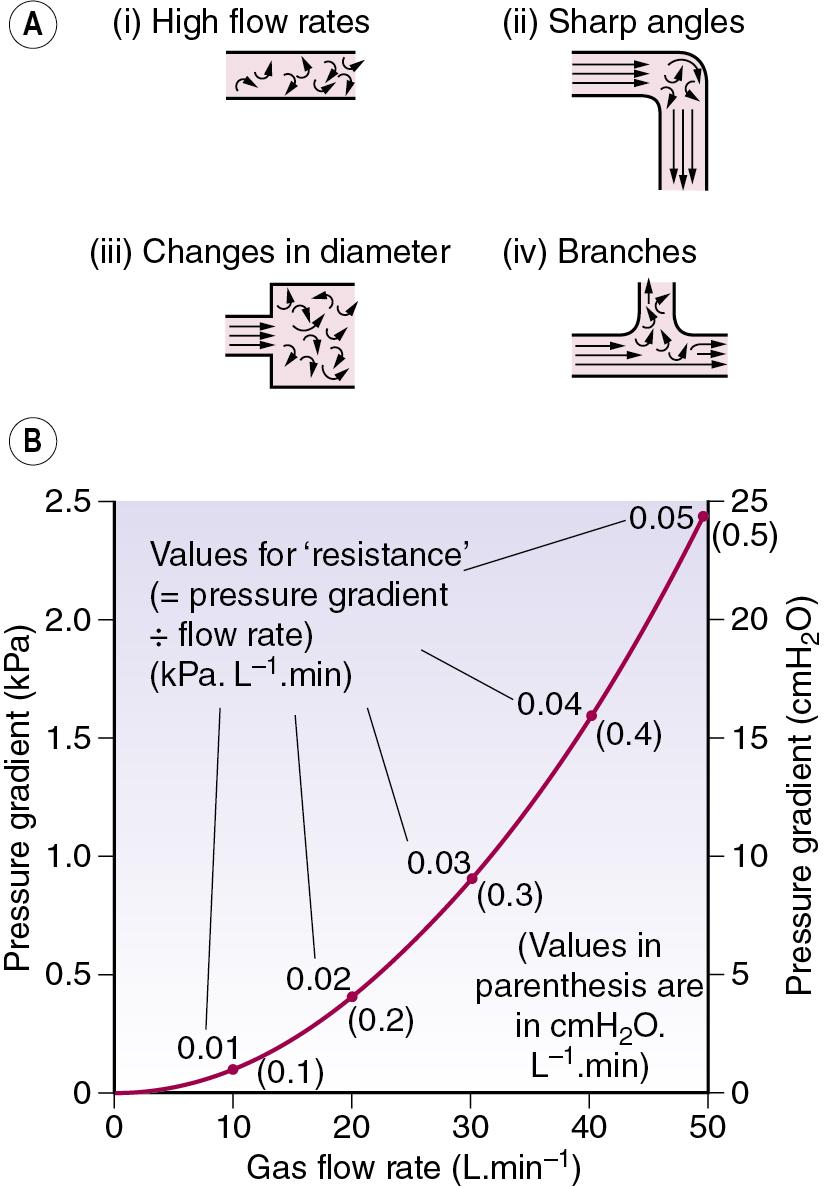
The square front means that no fresh gas can reach the end of a tube until the amount of gas entering the tube is almost equal to the volume of the tube. Turbulent flow is more effective than laminar flow in purging the contents of a tube, and also provides the best conditions for drawing a representative sample of gas from the periphery of a tube. Frictional forces between the tube wall and fluid become more important in turbulent flow.

Quantitative relationships

The relationship between driving pressure and flow rate differs from the relationship described earlier for laminar flow in three important respects:

  • 1.

    The driving pressure is proportional to the square of the gas flow rate.

  • 2.

    The driving pressure is proportional to the density of the gas and is independent of its viscosity.

  • 3.

    The required driving pressure is, in theory, inversely proportional to the fifth power of the radius of the tube (Fanning equation).

The square law relating driving pressure and flow rate is shown in Figure 3.3 , B . Resistance, defined as pressure gradient divided by flow rate, is not constant as in laminar flow, but increases in proportion to the flow rate. Units such as cmH 2 O.L −1 .s should therefore be used only when flow is entirely laminar. The following methods of quantification of ‘resistance’ may be used when flow is totally or partially turbulent.

  • Two constants. This method considers resistance as comprising two components, one for laminar flow and one for turbulent flow. The simple relationship for laminar flow given previously would then be extended as follows:


    Pressure gradient = k 1 ( flow ) + k 2 ( flow ) 2

    • where k 1 contains the factors of the Hagen–Poiseuille equation and represents the laminar flow component, and k 2 includes factors in the corresponding equation for turbulent flow.

  • The exponent n. Over a surprisingly wide range of flow rates, the previous equation may be condensed into the following single-term expression with little loss of precision:


    Pressure gradient = K ( flow ) n

    • where n has a value ranging from 1 with purely laminar flow to 2 with purely turbulent flow; the value of n being a useful indication of the nature of the flow. The constants for the normal human respiratory tract are


      Pressure gradient ( kPa ) = 0 .24 ( flow ) 1 .3

  • The graphical method. It is often convenient to represent ‘resistance’ as a graph of pressure difference against gas flow rate, on either linear or logarithmic coordinates. Logarithmic coordinates have the advantage that the plot is usually a straight line whether flow is laminar, turbulent or mixed, and the slope of the line indicates the value of n in the equation above.

Reynolds number

For long, straight unbranched tubes, the nature of the gas flow may be predicted from the value of the Reynolds number, which is a nondimensional quantity derived from the following expression:


Linear gas velocity × tube diameter × gas density Gas viscosity

The property of the gas that affects the Reynolds number is the ratio of density to viscosity. When the Reynolds number is less than 2000, flow is predominantly laminar, whereas at a value greater than 4000, flow is mainly turbulent. Between these values, both types of flow coexist. The Reynolds number also affects the entrance length (i.e., the distance required for laminar flow to become established), which is derived from:


Entrance length = 0 .03 × tube diameter × Reynolds number

Thus, for gases with a low Reynolds number, not only will resistance be less during turbulent flow, but laminar flow will become established more quickly after bifurcations, corners and obstructions.

Values for some gas mixtures that a patient may inhale are shown relative to air in Table 3.1 . Viscosities of respirable gases do not differ greatly, but there may be very large differences in density.

TABLE 3.1
Physical Properties of Clinically Used Gas Mixtures Relating to Gas Flow
Viscosity Relative to Air Density Relative to Air Density
Viscosity
Oxygen 1.11 1.11 1.00
70% N 2 O/30% O 2 0.89 1.41 1.59
80% He/20% O 2 1.08 0.33 0.31

Respiratory system resistance

Airway resistance

This results from frictional resistance in the airways. In the healthy subject, the small airways make only a small contribution to total airway resistance, because their aggregate cross-sectional area increases to very large values after about the eighth generation (see Fig. 1.5 ). Overall airway resistance is therefore dominated by the resistance of the larger airways.

Gas flow along pulmonary airways is complex compared with the theoretical tubes described earlier and consists of a variable mixture of both laminar and turbulent flow. Both the velocity of gas flow and airway diameter (and therefore Reynolds number) decrease in successive airway generations from a maximum in the trachea to almost zero at the start of the pulmonary acinus (generation 15). In addition, there are frequent divisions with variable lengths of approximately straight airway between. Finally, in large-diameter airways the entrance length is normally greater than the length of the individual airway. As a result of these purely physical factors, laminar flow cannot become established until approximately the 11th airway generation. Predominantly turbulent flow in the conducting airways has two practical implications. First, the physical characteristics of the airway lining will influence frictional resistance more with turbulent than with laminar flow, so changes in airway lining fluid consistency (page 165) will have a significant effect. Second, gas mixtures containing helium (low Reynolds number) are more beneficial in overcoming increased resistance in large airways and of less benefit in small airway disease such as asthma.

Tissue resistance

In 1955 a component of the work of breathing was attributed to the resistance caused by tissue deformation, and some years later this was measured in anaesthetized and paralysed subjects and termed the viscoelastic or ‘tissue’ component of respiratory resistance. Figure 3.4 shows the ‘spring and dashpot’ model, first described by D’Angelo et al., to illustrate tissue resistance. Dashpots here represent resistance and springs elastance (reciprocal of compliance). Upward movement of the upper bar represents an increase in lung volume, caused by contraction of the inspiratory muscles or the application of inflation pressure as shown in the diagram. There is good evidence that, in humans, the left-hand dashpot represents predominantly airway resistance. The spring in the middle represents the static elastance of the respiratory system. On the right there is a spring and dashpot arranged in series. With a rapid change in lung volume, the spring is extended, while the piston more slowly rises in the dashpot. In due course (2–3 seconds) the spring returns to its original length and so ceases to exert any influence on pressure/volume relationships. This spring therefore represents the time-dependent element of elastance. While it is still under tension at end-inspiration, the combined effect of the two springs results in a high elastance, of which the reciprocal is the dynamic compliance. If inflation is held for a few seconds and movement of the piston through the right-hand dashpot is completed, the right-hand spring ceases to exert any tension, and the total elastance is reduced to that caused by the spring in the middle. The reciprocal of this elastance is the static compliance, which is therefore greater than the dynamic compliance. The system shown in Figure 3.4 is only a simplified scheme to which many further components could be added; nevertheless the model accords well with experimental findings.

• Fig. 3.4, The spring and dashpot model of D’Angelo et al. 1 Inflation of the lungs is represented by the bar moving upwards. The springs represent elastance (reciprocal of compliance), and the dashpots represent resistance. The spring and dashpot in series on the right confers time dependence, which is because of viscoelastic tissue resistance.

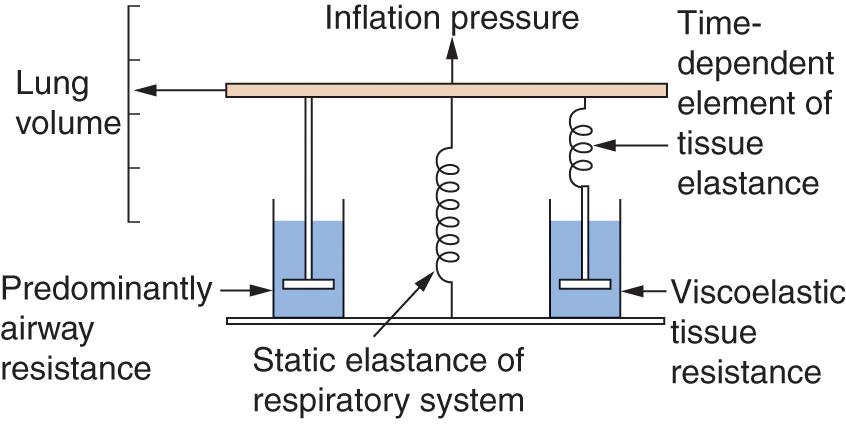
The time-dependent change in compliance represented by the spring and dashpot in series could be as a result of many factors. Redistribution of gas makes only a negligible contribution in a healthy human; the major component is because of viscoelastic flow resistance in tissue. In anaesthetized healthy subjects, tissue resistance is of the order of half of the respiratory system resistance, and seems to be largely unaffected by end-expiratory pressure or tidal volume. Tissue resistance originates from both lung and chest wall tissues, with a significant proportion originating in the chest wall. , The magnitude and importance of this component, particularly in lung disease, is often underestimated, and it is clearly important to distinguish airway resistance from that afforded by the total respiratory system. Separate measurement of tissue resistance is described in the following section.

Inertance as a component of respiratory system resistance

Respired gases, the lungs and the thoracic cage all have appreciable mass, and therefore inertia, which must offer an impedance to change in direction of gas flow, analogous to electrical inductance. This component, termed inertance, is extremely difficult to measure, but inductance and inertance offer an impedance that increases with frequency. Therefore, although inertance is generally believed to be negligible at normal respiratory frequencies, it may become appreciable during high-frequency ventilation ( Chapter 32 ).

Factors affecting respiratory resistance

In normal lungs respiratory resistance is controlled by changes in airway diameter mainly in small airways and bronchioles. This would be expected to alter only the airway component of respiratory resistance, but animal studies suggest that contraction of bronchial smooth muscle also causes changes in tissue resistance. Interdependence between the airway and parenchymal components of lung tissue is believed to occur such that airway constriction distorts the surrounding elastic tissue sufficiently to alter its viscoelastic properties. Airway calibre may be reduced by either physical compression (because of a reversal of the normal transluminal pressure leading to airway collapse) or by contraction of the smooth muscle in the airway wall.

Volume-related airway collapse

Effect of lung volume on resistance to breathing

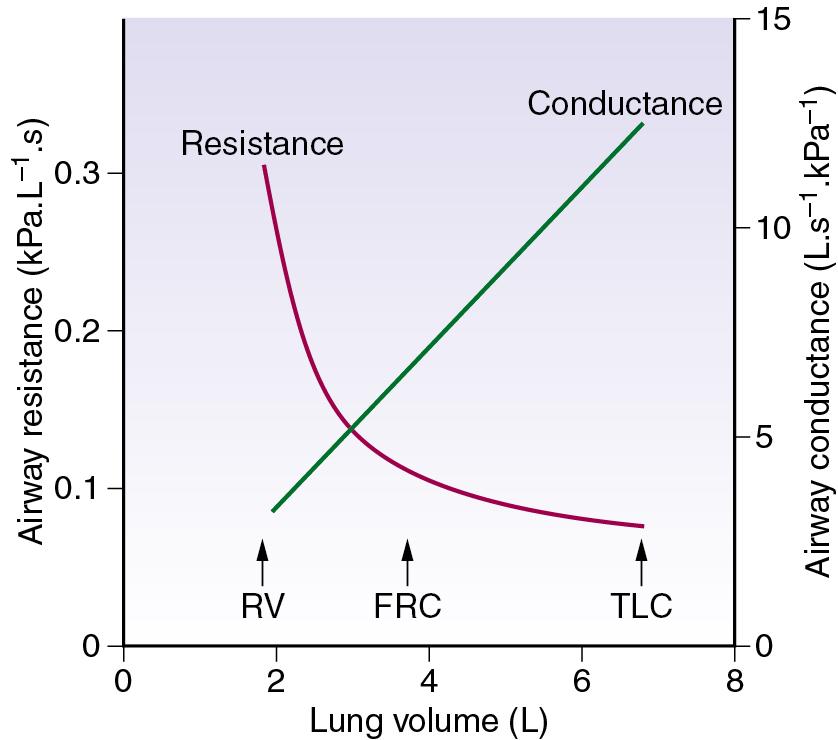
When lung volume is reduced, there is a proportional reduction in the volume of all air-containing components, including the air passages. Thus if other factors (such as bronchomotor tone) remain constant, airway resistance is an inverse function of lung volume ( Fig. 3.5 ), and there is a direct relationship between lung volume and the maximum expiratory flow rate that can be attained (see later). Quantifying airway diameter is difficult from these curves. It is therefore more convenient to refer to conductance, which is the reciprocal of resistance and is usually expressed as litres per second per cmH 2 O. Specific airway conductance (sG aw ) is the airway conductance relative to lung volume, or the gradient of the line showing conductance as a function of lung volume ( Fig. 3.5 ). Because it takes into account the important effect of lung volume on airway resistance, it is a useful index of bronchomotor tone.

• Fig. 3.5, Airway resistance and conductance as a function of lung volume (upright posture). The resistance curve is a hyperbola. Specific conductance (sG aw ) is the gradient of the conductance line. FRC , Functional residual capacity; RV , residual volume; TLC , total lung capacity.

You're Reading a Preview

Become a Clinical Tree membership for Full access and enjoy Unlimited articles

Become membership

If you are a member. Log in here

Related Posts