Physiology of Neurons

Neurons receive, combine, transform, store, and send information

Neurons have arguably the most complex job of any cell in the body. Consequently, they have an elaborate morphology and physiology. Each neuron is an intricate computing device. A single neuron may receive chemical input from tens of thousands of other neurons. It then combines these myriad signals into a much simpler set of electrical changes across its cellular membrane. The neuron subsequently transforms these ionic transmembrane changes according to rules determined by its particular shape and electrical properties and transmits a single new message through its axon, which itself may contact and inform hundreds of other neurons. Under the right circumstances, neurons also possess the property of memory; some of the information coursing through a neuron's synapses may be stored for periods as long as years.

This general scheme of neuronal function applies to most neurons in the vertebrate nervous system. However, the scheme is endlessly variable. For example, each region of the brain has several major classes of neurons, and each of these classes has a physiology adapted to perform specific and unique functions. In this chapter, the general principles of neuronal function are outlined, and the almost unlimited variability contained within the general schema is discussed.

Neural information flows from dendrite to soma to axon to synapse

Numerous dendrites converge on a central soma, or cell body, from which a single axon emerges and branches multiple times (see Fig. 10-1 ). Each branch culminates at a presynaptic terminal that contacts another cell. In most neurons, dendrites are the principal synaptic input sites, although synapses may also be found on the soma, on the axon hillock (the region of the soma neighboring the axon), or even directly on the axons. In some primary sensory neurons, the dendrites themselves are transducers of environmental energy. Regardless of their source, signals—in the form of voltage changes across the membrane—typically flow from dendrites to soma to axon and finally to synapses on the next set of cells. Under some circumstances electrical signals may also propagate in the opposite direction, from the soma outward along dendrites to their tips.

Excitatory input to a neuron usually generates an inward flow of positive charge (i.e., an inward current) across the dendritic membrane. Because the interior of a resting neuron is polarized negatively with respect to the external environment, this inward current, which makes the membrane voltage more positive (i.e., less negative), is said to depolarize the cell. Conversely, inhibitory input to a neuron usually generates an outward current and, thus, hyperpolarization.

If the neuron receives its input from a neighboring cell through a chemical synapse, neurotransmitters trigger currents by activating ion channels. If the cell is a sensory neuron, environmental stimuli (e.g., chemicals, light, mechanical deformation) activate ion channels and produce a flow of current. The change in membrane potential ( V _m ) caused by the flow of charge is called a postsynaptic potential (PSP) if it is generated at the postsynaptic membrane by a neurotransmitter and a receptor potential if it is generated at a sensory nerve ending by an external stimulus. In the case of synaptic transmission, the postsynaptic V _m changes may be either positive or negative. If the neurotransmitter is excit­atory and produces a depolarizing PSP, we refer to the PSP as an excitatory postsynaptic potential ( EPSP; see p. 210 ). On the other hand, if the neurotransmitter is inhibitory and produces a hyperpolarizing PSP, the PSP is an inhibitory postsynaptic potential (IPSP). In all cases, the stimulus produces a V _m change that may be graded from small to large depending on the strength or quantity of input stimuli (see p. 174 ). Stronger sensory stimuli generate larger receptor potentials; similarly, more synapses activated together generate larger PSPs. A graded response is one form of neural coding whereby the size and duration of the input are encoded as the size and duration of the change in the dendritic V _m .

The synaptic (or receptor) potentials generated at the ends of a dendrite are communicated to the soma, usually with substantial attenuation of the signal ( Fig. 12-1 A ). Extended cellular processes such as dendrites behave like leaky electrical cables (see pp. 201–203 ). As a consequence, dendritic potentials usually decline in amplitude before reaching the soma. As an EPSP reaches the soma, it may also combine with EPSPs arriving via other dendrites on the cell; this behavior is a type of spatial summation and can lead to EPSPs that are substantially larger than those generated by any single synapse (see Fig. 12-1 B, C ). Temporal summation occurs when EPSPs arrive rapidly in succession; when the first EPSP has not yet dissipated, a subsequent EPSP tends to add its amplitude to the residual of the preceding EPSP (see Fig. 12-1 D ).

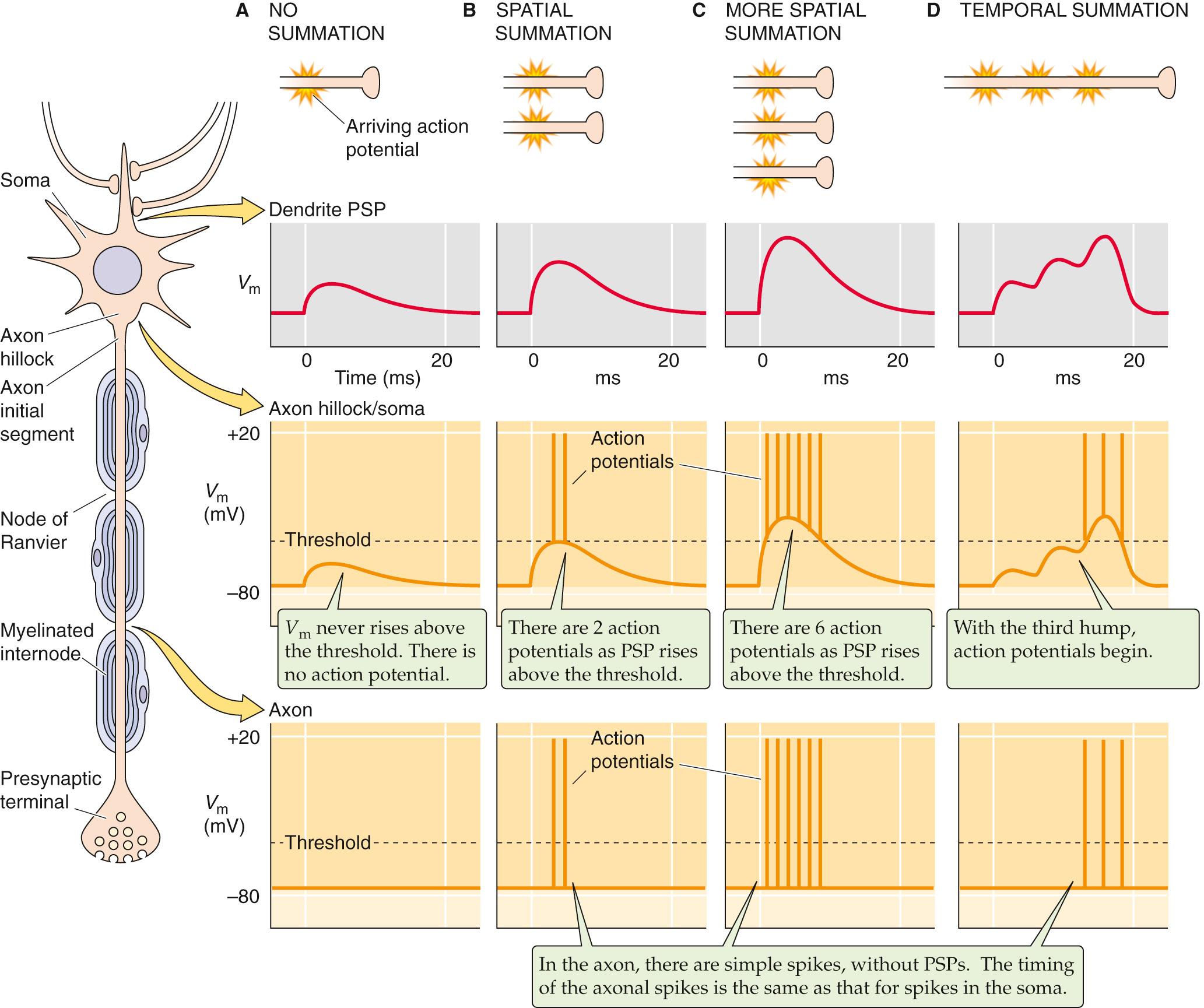
The tendency for synaptic and receptor potentials to diminish with distance along a dendrite puts significant limitations on their signaling abilities. If nothing else happened, these depolarizing potentials would simply dwindle back to the resting membrane potential as they spread through the soma and down into the axon. At best, this passive signal might be carried a few millimeters, clearly inadequate for commanding a toe to wiggle when the axon of the motor neuron stretching from the spinal cord to the muscles moving the foot might be 1000 mm long. Some amplification is therefore necessary for certain inputs to generate effective signals to and from the central nervous system (CNS). Amplification is provided in the form of regenerating action potentials. If the V _m change in the soma is large enough to reach the threshold voltage (see p. 173 ), the depolarization may trigger one or more action potentials between the soma and axon, as shown in Figure 12-1 B to D . Action potentials are large, rapid fluctuations in V _m . As described in Chapter 7 , an action potential is an efficient, rapid, and reliable way to carry a signal over long distances. However, notice that generation of action potentials entails another transformation of neuronal information: the neuron converts the graded-voltage code of the dendrites (i.e., the PSPs) to a temporal code of action potentials in the axon. The initiation site for action potentials in many types of neurons is the axon initial segment, the first stretch of unmyelinated axon past the soma.

Action potentials are fixed in amplitude, not graded, and have uniform shape. So how is information encoded by action potentials? This question has no simple answer and is still hotly debated. Because one axonal spike looks like another (with slight exceptions), neurons can vary only the number of spikes and their timing. For a single axon, information may be encoded by the average rate of action potential firing, the total number of action potentials, their temporal pattern, or some combination of these mechanisms. Figure 12-1 illustrates that as the synaptic potential in the soma increases in size, the resultant action potentials occur more frequently, and the burst of action potentials in the axon lasts longer. Notice also that by the time the signal has propagated well down the axon, the transformation has become complete—the graded potential has waned and vanished, whereas the action potentials have retained their size, number, and temporal pattern. The final output of the neuron is entirely encoded by these action potentials. When action potentials reach axonal terminals, they may trigger the release of a neurotransmitter at the next set of synapses, and the cycle begins again.

Dendrites attenuate synaptic potentials

Dendrites tend to be long and thin. Their cytoplasm has relatively low electrical resistivity, and their membrane has relatively high resistivity. These are the properties of a leaky electrical cable, which is the premise for cable theory (see p. 201 ). Leaky cables are like leaky garden hoses; if ionic current (or water) enters at one end, the fraction of it that exits at the other end depends on the number of channels (or holes) in the cable (hose). A good hose has no holes and all the water makes it through, but most dendrites have a considerable number of channels that serve as leaks for ionic current (see Fig. 7-22 ).

Cable theory predicts how much current flows down the length of the dendrite through the cytoplasm and how much of it leaks out of the dendrite across the membrane. As summarized in Table 7-3 , we can express the leakiness of the membrane by the resistance per unit area of dendritic membrane (specific membrane resistance, R _m ), which can vary widely among neurons. The intracellular resistance per cross-sectional area of dendrite (specific resistivity of the cytoplasm, R _i ) is also important in determining current flow inasmuch as a very resistive cytoplasm forces more current to flow out across the membrane rather than down the axis of the dendrite. Another important factor is cable diameter; thick dendrites let more current flow toward the soma than thin dendrites do. Figure 7-22 C illustrates the consequences of a point source of steady current flowing into a leaky, uniform, infinitely long cable made of purely passive membrane. The transmembrane voltage generated by the current falls off exponentially with distance from the site of current injection. The steepness with which the voltage falls off is defined by the length constant (λ; see p. 201 ), which is the distance over which a steady voltage decays by a factor of 1/ e (~37%). Estimates of the parameter values vary widely, but for brain neurons at rest, reasonable numbers are ~50,000 Ω ⋅ cm ² for R _m and 200 Ω ⋅ cm for R _i . If the radius of the dendrite (a) is 1 µm (10 ⁻⁴ cm), we can estimate the length constant of a dendrite by applying Equation 7-8.

Because dendrite diameters vary greatly, λ should also vary greatly. For example, assuming the same cellular properties, a thin dendrite with a radius of 0.1 µm would have a λ of only 354 µm, whereas a thick one with a radius of 5 µm would have a λ of 2500 µm. Thus, the graded signal voltage spreads farther in a thick dendrite.

Real dendrites are certainly not infinitely long, uniform, and unbranched, nor do they have purely passive membranes. Thus, quantitative analysis of realistic dendrites is complex. The termination of a dendrite decreases attenuation because current cannot escape farther down the cable. Branching increases attenuation because current has more paths to follow. Most dendrites are tapered. Gradually expanding to an increased diameter progressively increases λ and thus progressively decreases attenuation. Real membranes are never completely passive because all have voltage-gated channels, and therefore their R _m values can change as a function of voltage and time. Finally, in the working brain, cable properties are not constant but may vary dynamically with ongoing brain activity. For example, as the general level of synaptic input to a neuron rises (which might happen when a brain region is actively engaged in a task), more membrane channels will open and thus R _m will drop as a function of time, with consequent shortening of dendritic length constants. However, all these caveats do not alter the fundamental qualitative conclusion: voltage signals are attenuated as they travel down a dendrite.

So far, we have described only how a dendrite might attenuate a sustained voltage change. Indeed, the usual definition of length constant applies only to a steady-state voltage shift. An important complication is that the signal attenuation along a cable depends on the frequency components of that signal—how rapidly voltage changes over time. When V _m varies over time, some current is lost to membrane capacitance (see p. 158 ), and less current is carried along the dendrite downstream from the source of the current. Because action potentials and EPSPs entail rapid changes in V _m , with the fastest of them rising and falling within a few milliseconds, they are attenuated much more strongly than the steady-state λ implies. If V _m varies in time, we can define a λ that depends on signal frequency (λ _AC , where AC stands for “alternating current”). When signal frequency is zero (i.e., V _m is steady), λ = λ _AC . However, as frequency increases, λ _AC may fall sharply. Thus, dendrites attenuate high-frequency (i.e., rapidly changing) signals more than low-frequency or steady signals. Another way to express this concept is that most dendrites tend to be low-pass filters in that they let slowly changing signals pass more easily than rapidly changing ones.

Figure 12-2 A shows how an EPSP propagates along two different dendrites with very different length constants. If we assume the synapses trigger EPSPs of similar size in the end of each dendrite, then the dendrites with the longer λ deliver a larger signal to the axon hillock. How do leaky dendrites manage to communicate a useful synaptic signal to the soma? The problem is solved in two ways. The first solution deals with the passive properties of the dendrite membrane. The length (l) of dendrites tends to be relatively small in comparison to their λ; thus, none extends more than one or two steady-state length constants (i.e., the l /λ ratio is <1). One way that dendrites achieve a small l /λ ratio is to have a combination of diameter and R _m that gives them a large λ. Another way is that dendrites are not infinitely long cables but “terminated” cables. Figure 12-2 B shows that a signal is attenuated more in an infinitely long cable (curve a ) than in a terminated cable whose length ( l ) is equal to λ (curve b ). The attenuation of a purely passive cable would be even less if the terminated cable had a λ 10-fold greater than l (curve c ). Recall that in our example in Figure 12-2 A , such a 10-fold difference in λ underlies the difference in the amplitudes of the EPSPs arriving at the axon hillock.

The second solution to the attenuation problem is to endow dendrites with voltage-gated ion channels (see pp. 182–199 ) that enhance the signal more than would be expected in a purely “passive” system (curve d ). We discuss the properties of such “active” cables in the next section.

Dendritic membranes have voltage-gated ion channels

All mammalian dendrites have voltage-gated ion chan­nels that influence their signaling properties. Dendritic characteristics vary from cell to cell, and the principles of dendritic signaling are being studied intensively. Most dendrites have a relatively low density of voltage-gated channels (see pp. 182–199 ) that may amplify, or boost, synaptic signals by adding additional inward current as the signals propagate from distal dendrites toward the soma. We have already introduced the principle of an active cable in curve d of Figure 12-2 B . If the membrane has voltage-gated channels that are able to carry more inward current (often Na ⁺ or Ca ²⁺ ) under depolarized conditions, a sufficiently strong EPSP would drive V _m into the activation range of the voltage-gated channels. These voltage-gated channels would open, and their additional inward current would add to that generated initially by the synaptic channels. Thus, the synaptic signal would fall off much less steeply with distance than in a passive dendrite. Voltage-gated channels can be distributed all along the dendrite and thus amplify the signal along the entire dendritic length, or they can be clustered at particular sites. In either case, voltage-gated channels can boost the synaptic signal considerably, even if the densities of channels are far too low for the generation of action potentials.

An even more dramatic solution, used by a few types of dendrites, is to have such a high density of voltage-gated ion channels that they can produce action potentials, just as axons can. One of the best-documented examples is the Purkinje cell, which is the large output neuron of the cerebellum. As Rodolfo Llinás and colleagues have shown, when the dendrites of Purkinje cells are stimulated strongly, they can generate large, relatively broad action potentials that are mediated by voltage-gated Ca ²⁺ channels ( Fig. 12-3 ). Such Ca ²⁺ spikes can sometimes propagate toward—or even into—the soma, but these Ca ²⁺ action potentials do not continue down the axon. Instead, they may trigger fast Na ⁺ -dependent action potentials that are generated by voltage-gated Na ⁺ channels in the initial segment. The Na ⁺ spikes carry the signal along the axon in the conventional way. Both types of spikes occur in the soma, where the Na ⁺ spikes are considerably quicker and larger than the dendritic Ca ²⁺ spikes. The faster Na ⁺ spikes propagate only a short distance backward into the dendritic tree because the rapid time course of the Na ⁺ spike is strongly attenuated by the inherent filtering properties of the dendrites (i.e., the λ _AC is smaller for the rapid frequencies of the Na ⁺ action potentials than for the slower Ca ²⁺ action potentials). The dendrites of certain other neurons of the CNS, including some pyramidal cells of the cerebral cortex, can also generate spikes that are dependent on Ca ²⁺ , Na ⁺ , or both.

Dendritic action potentials, when they exist at all, tend to be slower and weaker, and with higher thresholds, than those in axons. The reason is probably that one of the functions of dendrites is to collect and to integrate information from a large number of synapses (often thousands). If each synapse were capable of triggering an action potential, there would be little opportunity for most of the synaptic inputs to have a meaningful influence on a neuron's output. The cell's dynamic range would be truncated; that is, a very small number of active synapses would bring the neuron to its maximum firing rate. However, if dendrites are only weakly excitable, the problem of signal attenuation along dendritic cables can be solved while the cell still can generate an output (i.e., the axonal firing rate) that is indicative of the proportion of its synapses that are active.

Another advantage of voltage-gated channels in dendrites may be the selective boosting of high-frequency synaptic input. Recall that passive dendrites attenuate signals of high frequency more than those of low frequency. However, if dendrites possess the appropriate voltage-gated channels, with fast gating kinetics, they will be better able to communicate high-frequency synaptic input.