Basics of Radiation Therapy

Summary of Key Points

Introduction and Historical Perspective
X-rays were discovered emanating from an energized Crookes tube by Wilhelm Roentgen in 1895. That some naturally occurring elements emitted ionizing radiation was discovered by Henri Becquerel 1896. The radioactive elements radium and polonium were isolated and characterized by the Curies in 1898.
Within a year or two, ionizing radiation was in use worldwide for medical imaging and radiation therapy.

Radiation Physics
Several types of ionizing radiation are used to treat patients; most are of the low linear energy transfer (LET), less biologically potent varieties.
Therapeutic x-rays (photons) and electrons are produced by linear accelerators but can also be produced by nuclear isotopes that undergo radioactive decay. These form the basis of, respectively, external beam radiation therapy and brachytherapy.
Ionizing radiation interacts with matter via several processes, the most important of which for clinical radiation therapy is Compton scattering.
Megavoltage photons from linear accelerators have the desirable property of delivering their maximum dose at 1 to 3 cm within the patient, thereby sparing the skin and, to some extent, other normal tissues.

Radiobiology of Radiation Therapy
Ionization of biomolecules from the deposition of energy by photons or particles can occur directly and indirectly. The most important cellular target for radiation is DNA, with irreparable or “misrepaired” double-stranded breaks believed to be the lesions most responsible for cell killing.
Radiation elicits diverse cellular responses that include the sensing of DNA damage, mobilization of DNA repair proteins, repair (or attempted repair) of DNA damage, triggering of cell cycle checkpoints, and, for irreparable or misrejoined damage, cell death by one of several mechanisms.
The most commonly applied model of cell survival probability is the linear-quadratic (alpha/beta) model, with the surviving fraction of irradiated cells described by the equation S = e ^{−(αD + βD ² )} . Whereas the α/β ratio can be used as a metric for describing cellular radiosensitivity, it has also been adapted to describe the time, dose, and fractionation response of irradiated tissues.
DNA damage and repair were initially inferred by monitoring increases in cell survival or tissue tolerance with fractionation. These phenomena were termed sublethal and potentially lethal damage repair or recovery.
Cells in different cell cycle phases possess different radiosensitivities; cells are most radiosensitive in the M phase of the cell cycle, and most resistant in S phase, particularly late S phase. Cells in G ₁ phase are of intermediate radiosensitivity.
Well-oxygenated cells are as much as three times more sensitive to radiation-induced killing than (severely) oxygen-deprived cells. Viable, hypoxic cells that exist in many human tumors but are mostly absent in normal tissues may be an impediment to tumor control. The elimination of such cells has been a long-standing clinical goal; however, hypoxia also may provide avenues for therapeutic gain through the use of hypoxia-directed therapies.
Radiation sensitizers, particularly cytotoxic chemotherapy, and, to a lesser extent, radiation protectors aim to improve the therapeutic ratio. Increasingly, targeted agents and immunotherapy are being combined with radiation in the hopes of therapeutic benefit.

Clinical Radiation Oncology
Radiation therapy is used in more than half of all patients with cancer, either as an adjuvant or neoadjuvant treatment in combination with surgery, as a definitive treatment alone or in combination with chemotherapy, as an organ-sparing therapy, or to palliate cancer-related symptoms.
Fractionation of radiation and altered fractionation schedules, including accelerated, hyperfractionated and hypofractionated radiation therapy, make use of differences in the fractionation sensitivity of normal and malignant tissues to achieve higher therapeutic ratios.
Radiation produces early effects such as mucositis, skin erythema, or desquamation and late effects such as fibrosis and carcinogenesis.

Planning and Delivery of Radiation Treatment
Patient simulation uses multiple imaging approaches to identify cancerous and healthy regions within the patient and to select appropriate beams to deliver dose to the tumor while minimizing dose to surrounding tissues.
The use of three-dimensional conformal treatment planning and delivery has permitted escalation of dose and improved sparing of normal tissues.
Intensity-modulated radiation therapy uses varying radiation beam intensities to more precisely sculpt the dose distribution around the tumor to improve the therapeutic ratio.
Image-guided radiation therapy uses real-time and/or daily imaging to ensure that the tumor is positioned such that the radiation beams are precisely delivered to the appropriate location within the patient.

Other Modalities in Radiation
Brachytherapy, the placement of radioactive sources immediately adjacent to the tumor, delivers extremely high-dose radiation to tumor tissue with a much lower dose to surrounding normal tissues.
Stereotactic radiosurgery and stereotactic body radiation therapy combine high dose-per-fraction irradiation with highly conformal treatment delivery to increase the therapeutic ratio while reducing overall treatment time.
Proton therapy has dose distribution advantages over photon therapy, and it may be used to deliver high doses of radiation to tumors in close proximity to sensitive normal structures.

Radiation therapy is one of the three established cancer treatment modalities and is used for the therapy of most types of solid tumors and for selected hematologic malignancies. It is used almost entirely for therapy of malignant disease, although it has a small role in preventing proliferation in selected benign diseases. Radiation therapy is routinely combined with surgery, chemotherapy, or both to improve therapeutic results. It is often used with surgery to destroy microscopic regions of tumor extension and with chemotherapy to destroy more effectively the primary tumor. An understanding of the therapeutic use of ionizing radiation requires a basic comprehension of both the physics of radiation therapy delivery and the biologic effects of the interaction of radiation with matter.

Overview of Radiation Physics

The toxic biologic effects of ionizing radiation, although complex, varied, and incompletely understood, form the basis for the use of radiation therapy as a cancer treatment. These biologic effects are initiated when packets of energy are deposited in a volume of tissue and remove electrons from constituent atoms through a process called ionization. Accordingly, the physics of radiation oncology is focused on the details of how, where, and how much energy can be deposited in diseased tissue in the hopes of eradicating it while minimizing the energy released in healthy tissue. This requires an understanding of the nature of the radiation and the matter through which it passes, and how that matter is changed as a result of the energy deposition events.

Nature of Matter and Radiation

All matter, biologic or otherwise, is composed of atoms. Atoms are made up of groups of electrons (small negatively charged particles) orbiting a nucleus consisting of protons (larger positively charged particles) and neutrons (uncharged particles having mass similar to that of a proton). A number of electrons that matches the number of protons is held in orbit around the nucleus by electrostatic attraction. A specific discrete set of possible electron orbits exists for each kind of atom, with each electron orbit corresponding to a specific energy. Moving an electron from one orbit to another requires adding or subtracting the energy difference between the two orbitals, and removing an electron from the atom entirely, a process called ionization, requires adding the full energy of the electron orbital.

The properties of a nucleus are defined by the number of protons and neutrons, with the number of protons defining the type of element and the number of protons and neutrons together defining the isotope. Analogously to the arrangement of electrons in an atom, protons and neutrons are arranged in discrete energy levels specific to a particular nucleus, and transitioning between energy levels requires adding or removing a comparable amount of energy. Of the 1400 known isotopes of the 92 naturally occurring elements, approximately 80% are unstable and spontaneously undergo a transition between energy levels, emitting energy in the process. The phenomenon of spontaneous energy release from a nucleus, called radioactivity, can take many forms, including combinations of the emission of γ-rays, the ejection of electrons, positrons, or alpha particles, and the transmutation of one element to another.

The term radiation refers to energy emitted from a source that is transmitted through a material or space. This radiation can then deposit its energy by interacting with the matter through which it passes. Regardless of source, most radiation used in radiation therapy involves electromagnetic interactions. This energy can take the form of packets of electromagnetic waves called photons, or it can be carried as the kinetic energy of freely propagating particulate radiation such as electrons, protons, or alpha particles.

Photons are packets of oscillating electric and magnetic fields propagating through space at the speed of light (3 × 10 ¹⁰ cm/s). Photons are characterized by their wavelength, which is the distance traversed by a wave over the course of a single oscillation. There are no real limits on the possible wavelengths of photons, but common examples range in wavelength from AM radio (10 ³ m) to visible light (10 ⁻⁷ m) to γ-rays (10 ⁻¹² m). Photons that have a shorter wavelength oscillate at a higher frequency (more oscillations per unit time) and are more energetic. Energy and frequency are related by the Planck constant (4.135 × 10 ⁻¹⁵ eV-s) and are generally expressed in units of electron volts (eV). Each eV is equivalent to the kinetic energy required to move one electron through a potential difference of 1 volt. The energy of a photon determines its ability to penetrate matter. Visible light (~1 eV) can interact with only the surface of objects. Diagnostic (kilo-electron-volts [keV]) and therapeutic (mega-electron-volts [MeV]) photons can penetrate much more deeply, allowing therapeutic effects anywhere in the body. Photons at therapeutic energies can pass through many centimeters of tissue before experiencing any interactions.

Most particulate radiation consists of energetic charged particles. The electric fields around these particles cause them to interact with all the other charged particles in the surrounding medium. Charged particles are therefore much more efficient at depositing energy in matter, as the particles will continuously lose their energy as they attract or repel other charged particles along their path. Many of these interactions will cause ionization, which correlates with the amount of biologic damage delivered. Heavy particles such as protons and alpha particles ionize matter very efficiently, lose energy more efficiently, and have a higher linear energy transfer (LET; i.e., amount of energy loss per length of the particle's track; see also later) than lighter particles such as electrons and positrons. Although most particulate radiation is charged, uncharged neutrons are also capable of depositing energy in a material. Unlike charged particles, neutrons can interact only with other nuclei. In general, this interaction takes the form of a collision with a proton. The proton recoils with some fraction of the neutron's initial energy. The positively charged proton then ionizes the surrounding particles, causing most of the biologic damage.

Interactions of Radiation and Matter

In order of roughly increasing energy, photons can interact with:

1.
The atom as a whole
2.
Tightly bound inner shell electrons
3.
Loosely bound outer shell electrons
4.
The extranuclear space surrounding the nucleus
5.
The nucleus itself

Coherent Scatter

Low-energy photons can be briefly absorbed by the bound electrons of an atom. If the photon lacks the energy to remove the electron from the atom, the photon energy will be immediately reemitted as another photon. The reemitted photon has the same energy as the incident photon, and close to the same direction of travel. Because no energy is deposited, coherent scattering does not contribute to dose deposition, but the small deflections of the photons from coherent scatter can cause blurring in diagnostic images. Coherent scattering accounts for approximately 10% of interactions at 30 keV and is negligible for most therapeutic energy beams.

Photoelectric Effect

Photons having sufficient energy to ionize an atomic electron can undergo the photoelectric effect ( Fig. 27.1 ). In this process, the photon energy is entirely absorbed. Some energy is lost to breaking the electron binding energy, and the rest is carried away as kinetic energy of the ejected electron. The probability of a photoelectric interaction scales with the cube of the atomic number (Z) and the inverse cube of the photon energy (E), making the photoelectric effect very sensitive to material type and much more prevalent for lower photon energies. The photoelectric effect is the dominant photon interaction in tissue below 30 keV.

Compton Scattering

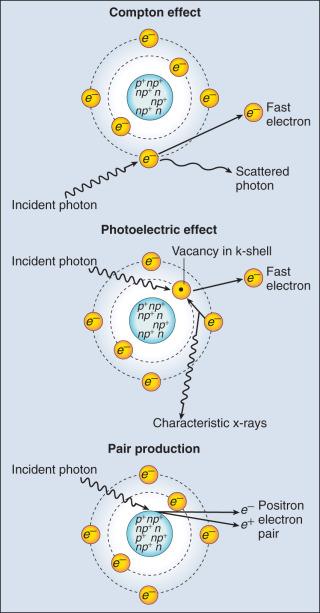
When photon energy is significantly higher than the binding energy of an electron, the photon can scatter from the electron without being absorbed, as illustrated in Fig. 27.1 . The result of this interaction is a photon with reduced energy and new direction, and a recoil electron with some fraction of the initial photon energy. The energy of the scattered electron varies with the scattering direction. An electron scattered in the direction of the incident photon claims most of the initial photon energy, whereas electrons scattered at greater angles have successively less energy. Compton scattering is only weakly dependent on Z and is the dominant photon interaction in tissue between 30 keV and 30 MeV.

Pair Production

Above 1.022 MeV, photons can interact in the presence of a strong nuclear field. The photon will disappear and spontaneously become an electron-positron pair (see Fig. 27.1 ). The electron and positron will divide the initial photon energy between them to create their mass and kinetic energy. These particles will lose their energy as they interact with the surrounding materials. On losing all their energy, the electrons will be absorbed into an atom. The positron, on the other hand, will annihilate by interacting with a local electron, creating two 511-keV photons. (This annihilation reaction is what is detected during positron emission tomography [PET] scanning.) Pair production is the dominant atomic interaction in tissue for photons above 30 MeV, and therefore has only a minor effect in radiation therapy in which energies are significantly lower.

Photodisintegration

Above a threshold energy, a photon can be absorbed into an atomic nucleus and cause one of the nucleons (a proton or a neutron) to be ejected. This process is called photodisintegration. Photodisintegration is more probable in high- Z materials (such as metals) and therefore is more likely to happen during photon generation in a linear accelerator than in tissue. Neutrons produced in this manner can contribute a significant background radiation dose to patients receiving radiation therapy from very high-energy machines. However, photodisintegration is negligible for accelerators operating below 10 MV.

Charged Particle Interactions

Charged particles will lose and transfer their energy to a medium through two mechanisms: collision and radiation. Collision energy loss by an energetic charged particle refers to the energy transfer resulting in ionization, excitation, and molecular damage. In a collision event, energy is absorbed in the medium at or very near the site of the interaction. Collision energy loss accounts for more than 95% of energy loss in tissue for therapeutic energy electrons, and is the major source of absorbed dose along the path of the electrons. Radiative energy loss occurs when particles are accelerated in the electric field of a nucleus and emit a fraction of their energy as a photon. This process, called bremsstrahlung, is relatively unimportant in tissue but is fundamental to the production of therapeutic photons in a linear accelerator.

Most electromagnetic interactions result from an interplay between photons and electrons because many photon interactions result in atomic ionization and release of an energetic electron, with some of the electron energy being converted back into photons through the bremsstrahlung process. Thus the effects of therapeutic beams passing through tissue can be described as a photon-electron shower, with the highly penetrating photons carrying energy deeper into tissue until a scattering event occurs, and the resulting scattered electrons depositing most of the resulting energy locally through collisional interactions.

Generation of Therapeutic Radiation

To be useful in radiation therapy, radiation must be generated in a manner in which it can be directed at the targeted tissues. Radiation for cancer therapy is predominantly generated through two means: linear accelerators and radioactive sources.

Linear Accelerators

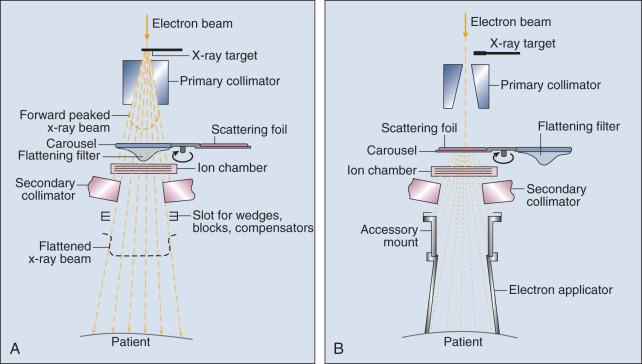
The most common modality used in radiation oncology is external beam radiation therapy (EBRT). Although a small number of radiation therapy facilities generate external beams using radioactive sources such as cobalt-60 ( ⁶⁰ Co), the vast majority of therapeutic electromagnetic radiation is generated in a linear accelerator. A linear accelerator is a device that accelerates charged particles (electrons) to velocities near the speed of light by using oscillating electric fields to push the electrons through a series of accelerating cavities. A schematic of a linear accelerator is shown in Fig. 27.2A . Electrons are accelerated to energies between 4 and 18 MeV. Electric and magnetic fields focus and steer the high-energy electrons such that they strike a thin metal target that stops the electron beam, with some fraction of the electron energy being converted to a spray of photons through the bremsstrahlung process. The bremsstrahlung photons, called x-rays, move approximately in the same direction as the electrons and have an energy spectrum ranging from a few 10s of keV up to the maximum energy of the initial electrons. The resulting photon beam then passes through a series of filters and beam-shaping elements that flatten and define the edges of the beam.

Figure 27.2, Schematic of the treatment head of a modern linear accelerator operating in photon-production mode (A) or electron-production mode (B).

The dose from a photon beam is related to its intensity, defined as the number of photons per unit area. Two major effects serve to decrease the intensity of a photon beam as it passes through tissue. First, as with any photon source, the beam intensity decreases with increasing distance from the source, just as is the case for a light bulb. In addition, beam intensity decreases as photons are attenuated from the beam via various scattering and absorption effects. This leads to a characteristic decrease in intensity versus depth in tissue that varies based on photon energy. Although the photon intensity begins decreasing immediately on entering a material, the energy released through the photon interactions is spread over a few centimeters as the electrons scattered by the photons gradually lose their energy as they pass through the material. The resulting dose distribution is characterized by a region of rapid increase near the surface, a leveling off of dose at a depth of 1 to 3 cm, and a gradual dose fall-off as depth increases. The plot of dose versus depth is called a percentage depth dose (PDD) curve, as shown in Fig. 27.3 . Because higher-energy photons are more penetrating, higher-energy beams will attenuate more slowly, leading to a more gradual decrease in dose with depth.

Figure 27.3, Percent depth-dose curves for a variety of radiation types used in clinical radiation oncology. These include photons (110 KV, cobalt-60 [ 60 Co], 6 MV and 18 MV) and various energies of electrons (6 MeV, 12 MeV, and 20 MeV). The inset shows the pattern or absorption at shallow depths and provides an illustration of the skin-sparing effect of photons.

Linear accelerators designed to produce photon beams can also be configured to produce therapeutic electron beams. Removing the photon-generating target and replacing it with a comparatively thinner electron scattering foil allows the transmission of the initial electron beam, but not without scattering the initially narrow beam into a broader distribution. Multiple filters and beam-shaping elements, as shown in Fig. 27.2B , produce an even distribution of customized shape at the surface of the patient. Electron beams lose their energy through different types of interactions than photons, leading to a different pattern of dose versus depth for electron beams. Rather than periodically removing photons from the beam through attenuation, electrons lose their energy gradually and at a relatively constant rate until the entire kinetic energy of the electron is expended, and the particles simply stop. Ideally, this would lead to a region of constant dose with increasing depth until the depth of full energy loss is reached, at which point the dose would drop abruptly to zero, with the depth of the dose drop-off being dependent on the initial electron energy. In practice, scattering and redirection of electrons within the beam lead to a mildly peaked dose plateau region and a somewhat more gradual dose fall-off, as shown in Fig. 27.3 .

Radioactive Sources

Unstable isotopes can spontaneously decay to lower energy states, releasing energy in the process. This radioactive decay can result in the emission of therapeutically useful photons, electrons, or other decay products. The degree to which a sample is radioactive is called its activity and is defined as a number of decays per unit time. The activity of a sample depends both on the amount of the isotope and how quickly the isotope decays. The historical unit of activity is the curie (Ci), which is defined as 3.7 × 10 ¹⁰ atomic decays per second, corresponding to the decay rate of 1 g of radium-226. Activity is also specified in becquerels (Bq), defined as one decay per second.

Because the rate of disintegration is proportional to the number of nuclei present, the absolute number of radioactive nuclei will decay exponentially. Activity is proportional to the number of nuclei, so a sample's activity, and therefore its ability to deliver dose, will decay with the same exponential behavior. The decay is described by A(t) = A ₀ e ^−λt , where A is the current activity, A ₀ is the activity at time zero, and λ is a decay constant for the isotope in question. The decay rates for various isotopes are more commonly given as the time required for one-half of the sample to decay away; this period of time is called the half-life of the isotope.

Therapeutically useful isotopes vary in half-life and in energy of emitted particles, as shown in Table 27.1 . Isotopes emitting higher-energy particles can deliver significant amounts of dose farther from the radioactive source than those emitting lower-energy particles. One type of radioactive source, ⁶⁰ Co, emits photons with an average energy of 1.25 MeV, which is sufficiently similar to photon energies found in linear accelerators that ⁶⁰ Co can be used as an external source to treat targets deep in a patient. Most other therapeutically useful radioactive sources emit lower-energy radiation with less penetrating power and must be placed in close proximity to the area to be treated. Sources are formed into small sealed seeds, typically 1 to 5 mm in size, and can be inserted into the treatment area on a temporary or permanent basis. The dose rate falls off very quickly with distance from a seed because of both rapid attenuation and the rapid spread of photons as they move away from the source.

Table 27.1

Therapeutically Useful Radioisotopes

Isotope	Half-Life Average	Energy (keV)
PHOTON
Radium-226 ( ²²⁶ Ra)	1620 yr	830
Cesium-137 ( ¹³⁷ Cs)	30 yr	662
Gold-198 ( ¹⁹⁸ Au)	2.7 days	412
Iridium-192 ( ¹⁹² Ir)	73.8 days	370
Iodine-125 ( ¹²⁵ I)	60 days	28
Palladium-103 ( ¹⁰³ Pd)	16.97 days	21
BETA
Phosphorus-32 ( ³² P)	14.3 days	1710
Strontium-90/yttrium-90 ( ⁹⁰ Sr/ ⁹⁰ Y)	28.5 yr/2.7 days	550/2280
Tungsten-188/rhenium-188 ( ¹⁸⁸ W/ ¹⁸⁸ Re)	69.4 days /17 h	350/2120
Rhenium-186 ( ¹⁸⁶ Re)	3.8 days	1070
Zinc-62/copper-62 ( ⁶² Zn/ ⁶² Cu)	9.3 h/9.7 min	660/2930
Xenon-133 ( ¹³³ Xe)	5.2 days	360
Iodine-131 ( ¹³¹ I)	8.0 days	600
Strontium-89 ( ⁸⁹ Sr)	50.5 days	1495
Holmium-166 ( ¹⁶⁶ Ho)	26.8 h	1850

Delivery of Therapeutic Radiation

The cytotoxic properties of ionizing radiation provide an opportunity for tumor control, but also require that care be exercised to limit the exposure of healthy tissue to radiation. For EBRT, linear accelerators are typically mounted on rotating gantries ( Fig. 27.4 ) that allow beams to pass through the patient and the target from a variety of directions. By placing the area to be treated at or near the center of rotation, multiple beams can be made to overlap in the region of the tumor, delivering a high dose to the overlap area and a comparatively low dose to other areas. For treatment based on implanted radioactive sources, a procedure known as brachytherapy, proper dose delivery consists of designing and delivering a three-dimensional (3D) distribution of radioactive seeds within the volume to be treated, creating a high-dose region that decreases rapidly beyond the treatment volume. Both of the aforementioned treatment modalities require a 3D understanding of the patient anatomy, and require that:

1.
The tumor be precisely located within the patient anatomy
2.
A treatment plan be customized for an individual patient
3.
The patient be reliably and reproducibly positioned relative to the radiation sources such that the intended radiation pattern can be precisely delivered

Figure 27.4, Layout for gantry-based radiation therapy machines. The linear accelerator rotates around a single point called the isocenter. Patients are placed on a movable table to align the area to be treated and the isocenter.

You're Reading a Preview

Become a Clinical Tree membership for Full access and enjoy Unlimited articles

Become membership

If you are a member. Log in here

Summary of Key Points

Introduction and Historical Perspective

Radiation Physics

Radiobiology of Radiation Therapy

Clinical Radiation Oncology

Planning and Delivery of Radiation Treatment

Other Modalities in Radiation