Physical Address
304 North Cardinal St.
Dorchester Center, MA 02124
Both ventilation and perfusion are distributed preferentially to dependent regions of the lung as a result of gravity and lung structure, and vary with posture and lung volume.
In healthy lungs ventilation and perfusion are closely matched, with little variation of the ventilation to perfusion ( ![]() ) ratio in different lung regions.
) ratio in different lung regions.
Regions of lung with a ![]() ratio of 0 represent intrapulmonary shunting of mixed venous blood, whilst regions with a
ratio of 0 represent intrapulmonary shunting of mixed venous blood, whilst regions with a ![]() ratio of infinity contribute to the alveolar dead space.
ratio of infinity contribute to the alveolar dead space.
Physiological dead space describes the part of each tidal volume that does not take part in gas exchange, and is made up of alveolar and anatomical dead space components.
The lung may be considered as a simple exchanger with a gas inflow and outflow, and a blood inflow and outflow ( Fig. 7.1 ). There is near-equilibrium of oxygen and carbon dioxide tensions between the two outflow streams from the exchanger itself. This theoretical model assumes that gas flow in and out of the alveolus and blood flow through the pulmonary capillary are both continuous. This assumption may be true within some human alveoli where, at normal tidal volumes, gas movement is by diffusion (page 6), but pulmonary capillary blood flow is pulsatile (page 74). This model has been deliberately drawn without countercurrent flow, which would be far more efficient. Such a system operates in the gills of fishes and the lungs of birds (page 301), and brings the P o 2 of arterial blood close to the P o 2 of their environment.
Gas exchange will clearly be optimal if ventilation and perfusion are distributed in the same proportion to one another throughout the lung. Conversely, to take an extreme example, if ventilation was distributed entirely to one lung and perfusion to the other, there could be no gas exchange, although total ventilation and perfusion might each be normal. This chapter begins with consideration of the spatial and temporal distribution of ventilation, followed by similar treatment for the pulmonary circulation. Distributions of ventilation and perfusion are then considered in relation to one another. Finally, the concepts of dead space and shunt are presented.
Distribution between the two lungs in the normal subject is influenced by posture and by the manner of ventilation. By virtue of its larger size, the right lung normally enjoys a ventilation slightly greater than the left lung in both the upright and the supine positions ( Table 7.1 ). In a conscious patient in the lateral positions, the lower lung is always better ventilated, regardless of the side on which the subject is lying, although there still remains a bias in favour of the right side. Fortunately, the preferential ventilation of the lower lung accords with increased perfusion of the same lung, so the ventilation/perfusion ratios of the two lungs are not greatly altered on assuming the lateral position. However, the upper lung tends to be better ventilated in the anaesthetized patient in the lateral position, regardless of the mode of ventilation and particularly with an open chest ( Table 7.1 ).
| Supine | Right Lateral (Left Side Up) | Left Lateral (Right Side Up) | ||||
|---|---|---|---|---|---|---|
| Right Lung | Left Lung | Right Lung | Left Lung | Right Lung | Left Lung | |
| Conscious | 1.69 53% |
1.39 47% |
1.68 61% |
2.07 39% |
2.19 47% |
1.38 53% |
| Anaesthetized spontaneous breathing | 1.18 52% |
0.91 48% |
1.03 45% |
1.32 55% |
1.71 56% |
0.79 44% |
| Anaesthetized artificial ventilation | 1.36 52% |
1.16 48% |
1.33 44% |
2.21 56% |
2.29 60% |
1.12 40% |
| Anaesthetized thoracotomy | — 83% |
— 17% |
||||
In addition to causing postural differences between ventilation of the left and right lungs, gravity also influences the distribution of ventilation within each lung. Lung tissue may be considered as a semifluid or gel-like substance confined within the chest cavity, and the weight of the tissue above compresses the tissue below such that the density of the lung increases as vertical height reduces ( Fig. 7.5 ). Thus in dependent areas lung tissue is less expanded than in nondependent areas, and so is more compliant and receives more ventilation.
Distribution of ventilation to horizontal slices of lung has been studied for many years by inhalation of radioactive isotopes, and more recently by advanced computerized tomography techniques. In the upright position, with slow vital capacity inspirations, uppermost slices of the lung have a ventilation of around one-third that of slices at the bases. A slow inspiration from functional residual capacity (FRC), as occurs during normal resting ventilation, results in a smaller vertical gradient down the lung, with the ratio of basal to apical ventilation being approximately 1.5:1.
In any horizontal position the vertical height of the lung is reduced by about 30%, and therefore the gravitational force generating maldistribution is much less. A variety of scanning techniques have been described that quantify regional ventilation in the supine position (page 105), and have confirmed earlier findings that normal tidal breathing results in a small degree of preferential ventilation of the posterior slices of the lungs compared with the anterior slices.
Gravity is not the only factor influencing regional ventilation; lung structure also contributes. A study of normal human lungs, rapidly frozen at a constant transpulmonary pressure and assessed using microcomputed tomographic imaging, found that the density of alveoli present in the parenchyma varies with lung height and volume. At total lung capacity (TLC) in the upright position was found to be 32 alveoli per mm 3 at the lung apex and 21 at the lung base. This difference in alveolar density will tend to mitigate the effect of the different transpulmonary pressure between apex and base, with a smaller number of alveoli at the lung base each receiving more ventilation. This may explain why there is such a small difference in regional ventilation at FRC. However, when these anatomical results were scaled down to lung volume at FRC, the authors estimated similar alveolar density at apex and base (approximately 47 alveoli per mm 3 ), which contradicts many other earlier studies described below suggesting a greater alveolar density in dependent regions. Scanning techniques with the ability to measure ventilation in areas of lung only a few cubic millimetres in size have also demonstrated increased ventilation in central, compared with peripheral, lung regions. This is likely to result from unequal branching patterns of the airways in a similar fashion to that seen in pulmonary blood vessels (see later).
Starting from FRC, preferential ventilation of the dependent parts of the lung is only present at inspiratory flow rates below 1.5 L.s −1 . At higher flow rates, distribution becomes approximately uniform. Fast inspirations from FRC reverse the distribution of ventilation, with preferential ventilation of the upper parts of the lungs, which is contrary to the distribution of pulmonary blood flow (see later). Normal inspiratory flow rate is, however, much less than 1.5 L.s −1 (∼0.5 L.s −1 ), so there will be a small vertical gradient of ventilation during normal breathing.
The rate of inflation of the lung as a whole is a function of inflation pressure, compliance and airway resistance. It is convenient to think in terms of the time constant (explained in Appendix E ), which is the product of the compliance and resistance and is (1) the time required for inflation to 63% of the final volume attained if inflation is prolonged indefinitely or (2) the time that would be required for inflation of the lungs if the initial gas flow rate were maintained throughout inflation (see Appendix E , Fig. E.6 ).
These considerations apply equally to large and small areas of the lungs; Figure 2.6 shows fast and slow alveoli, the former with a short time constant and the latter with a long time constant. Figure 7.2 shows some of the consequences of different functional units of the lung having different time constants. For simplicity, Figure 7.2 describes the response to passive inflation of the lungs by development of a constant mouth pressure, but the considerations are fundamentally similar for both spontaneous respiration and artificial ventilation.
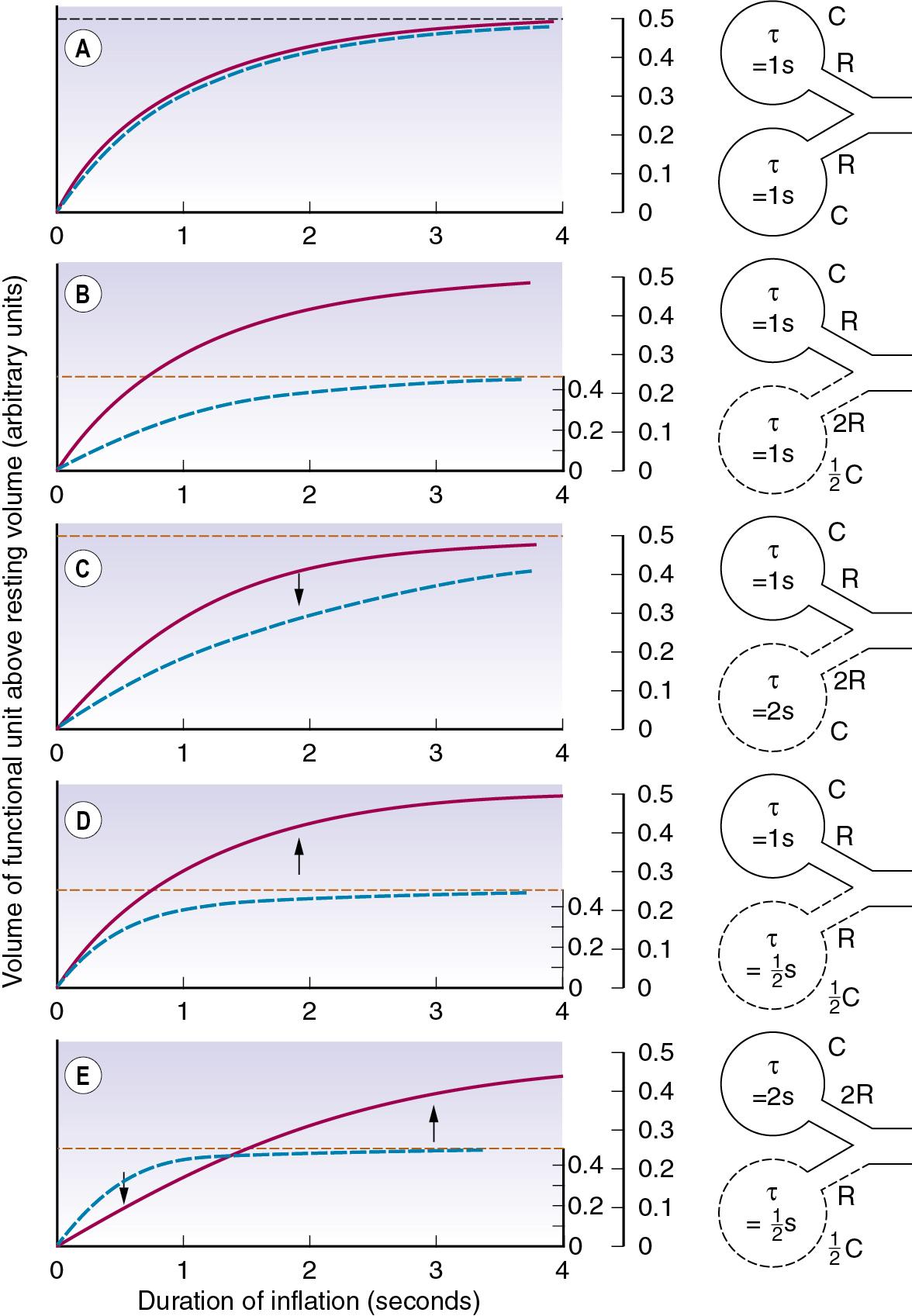
Figure 7.2 , A , shows two functional units of identical compliance and resistance. If the mouth pressure is increased to a constant level, there will be an increase in volume of each unit equal to the mouth pressure multiplied by the compliance of the unit. The time course of inflation will follow the wash-in type of exponential function ( Appendix E ), and the time constants will be equal to the product of compliance and resistance of each unit, and therefore identical. If the inspiratory phase is terminated at any instant, the pressure and volume of each unit will be identical, and no redistribution of gas will occur between the two units.
Figure 7.2 , B , shows two functional units, one of which has half the compliance but twice the resistance of the other. The time constants of the two will thus be equal. If a constant inflation pressure is maintained, the one with the lower compliance will increase in volume by half the volume change of the other. Nevertheless, the pressure build-up within each unit will be identical. Thus, as in the previous example, the relative distribution of gas between the two functional units will be independent of the rate or duration of inflation. If the inspiratory phase is terminated at any point, the pressure in each unit will be identical, and no redistribution will occur between the different units.
In Figure 7.2 , C , the compliances of the two units are identical, but the resistance of one is twice that of the other. Therefore its time constant is double that of its fellow, and it will fill more slowly, although the volume increase in both units will be the same if inflation is prolonged indefinitely. Relative distribution between the units is thus dependent on the rate and duration of inflation. If inspiration is checked by closure of the upper airway after 2 seconds (for example), the pressure will be higher in the unit with the lower resistance. Gas will then be redistributed from one unit to the other, as shown by the arrow in the diagram.
Figure 7.2 , D , shows a pair of units with identical resistances, but the compliance of one is half that of the other. Its time constant is thus half that of its fellow, and it has a faster time course of inflation. However, because its compliance is half that of the other, the ultimate volume increase will only be half that of the other unit when inflation is prolonged indefinitely. The relative distribution of gas between the two units is dependent on the rate and duration of inflation. Pressure rises more rapidly in the unit with the lower compliance, and if inspiration is checked by closure of the upper airway at 2 seconds (for example), gas will be redistributed from one unit to the other, as shown by the arrow.
An interesting and complex situation occurs when one unit has an increased resistance and the other a reduced compliance ( Fig. 7.2 , E ). This combination also features in the presentation of the concept of fast and slow alveoli in Figure 2.7 . In the present example the time constant of one unit is four times that of the other, whereas the ultimate volume changes are determined by the compliance as in Figure 7.2 , D . When the inflation pressure is sustained, the unit with the lower resistance (the ‘fast alveolus’) shows the greater volume change at first, but rapidly approaches its equilibrium volume. Thereafter the other unit (the ‘slow alveolus’) undergoes the major volume changes, the inflation of the two units being out of phase with one another. Throughout inspiration, the pressure build-up in the unit with the shorter time constant is always greater and, if inspiration is checked by closure of the upper airway, gas will be redistributed from one unit to the other, as shown by the arrows in Figure 7.2 , E .
These complex relationships may be summarized as follows. If the inflation pressure is sustained indefinitely, the volume change in different units of the lungs will depend solely upon their regional compliances. If their time constants are equal, the build-up of pressure in the different units will be identical at all times during inflation, and therefore:
Distribution of inspired gas will be independent of the rate, duration or frequency of inspiration.
Dynamic compliance (so far as it is influenced by considerations discussed in relation to Fig. 2.6 ) will not be affected by changes in frequency and should not differ greatly from static compliance.
If inspiration is checked by closure of the upper airway, there will be no redistribution of gas within the lungs.
If, however, the time constants of different units are different, it follows that:
Distribution of inspired gas will be dependent on the rate, duration and frequency of inspiration.
Dynamic compliance will be decreased as respiratory frequency is increased and should differ significantly from static compliance.
If inspiration is checked by closure of the upper airway, gas will be redistributed within the lungs.
If different functional units of the lung empty synchronously during expiration, the composition of the expired air will be approximately constant after the gas in the airways (anatomical dead space) has been flushed out. However, this will not occur when there is maldistribution with fast and slow units, as shown in Figure 2.6 . The slow units are slow both to fill and to empty, and thus are hypoventilated for their volume; therefore they tend to have a high P co 2 and low P o 2 , and are slow to respond to a change in the inspired gas composition. This forms the basis of the single-breath test of maldistribution, in which a single breath of 100% oxygen is used to increase alveolar P o 2 and decrease alveolar P n 2 . The greatest increase of P o 2 will clearly occur in the functional units with the best ventilation per unit volume, which will usually have the shortest time constants. The slow units will make the predominant contribution to the latter part of exhalation, when the mixed exhaled P o 2 will decline, and the P n 2 will increase. Thus the expired alveolar plateau of nitrogen will be sloping upwards in patients with maldistribution. It should, however, be stressed that this test will only be positive if maldistribution is accompanied by sequential emptying of units because of differing time constants. For example, Figure 7.2 , B , shows definite maldistribution because of the different regional compliances that directly influence the regional ventilation. However, because time constants are equal, there will be a constant mix of gas from both units during the course of expiration (i.e., no sequential emptying), and therefore the alveolar plateau would remain flat in spite of P o 2 and P n 2 being different for the two units. However, maldistribution because of the commoner forms of lung disease is usually associated with different time constants and sequential emptying. Routine continuous monitoring of expired carbon dioxide concentration during anaesthesia allows some assessment of maldistribution of ventilation. As for the single-breath nitrogen test, an upward sloping expiratory plateau of carbon dioxide indicates sequential emptying of alveoli with different time constants (page 135), but a level plateau does not indicate normal distribution of ventilation, just equal time constants of lung units.
Because the pulmonary circulation operates at low pressure, it is rarely distributed evenly to all parts of the lung, and the degree of nonuniformity is usually greater than for gas.
Measuring unilateral pulmonary blood flow in humans is difficult, but indirect methods show that unilateral pulmonary blood flow is similar to the distribution of ventilation observed in the supine position ( Table 7.1 ). In the lateral position, the diameter of the thorax is of the order of 30 cm, and so the column of blood in the pulmonary circulation exerts a hydrostatic pressure that is high in relation to the mean pulmonary arterial pressure. A fairly gross maldistribution therefore occurs, with much of the upper lung comprising zone 2 and much of the lower lung comprising zone 3 (see Fig. 6.5 ).
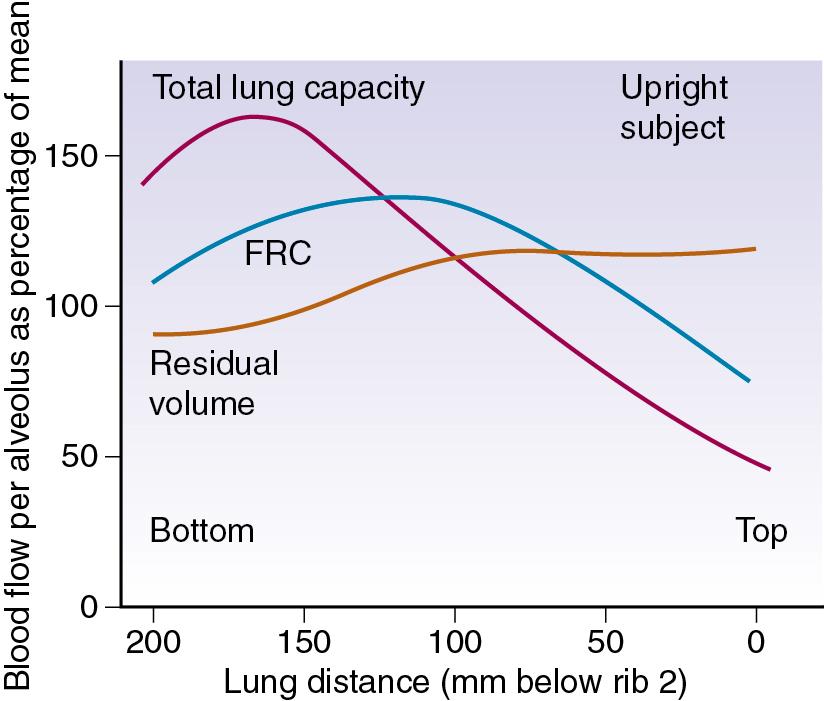
In the previous chapter, it was shown how the pulmonary vascular resistance is mainly in the capillary bed and is governed by the relationship between alveolar, pulmonary arterial and pulmonary venous pressures. Early studies with radioactive tracers in the blood took place at TLC and showed flow increasing progressively down the lung in the upright position. However, Hughes et al. later found that there was also a significant reduction of flow in the most dependent parts of the lung, which became known as zone 4, an effect that became progressively more important as lung volume was reduced from TLC towards the residual volume (RV). Figure 7.3 is derived from the work of Hughes’ group, and shows that pulmonary perfusion per alveolus is, in fact, reasonably uniform at the lung volumes relevant to normal tidal exchange. However, the dependent parts of the lung contain slightly larger numbers of smaller alveoli than the apices at FRC, and the perfusion per unit lung volume is therefore increased at the bases. The mechanism of reduced blood flow in zone 4 remains uncertain, possibilities including compression of extraalveolar vessels (page 77), hypoxic pulmonary vasoconstriction (page 80) or the local branching structure of pulmonary blood vessels.
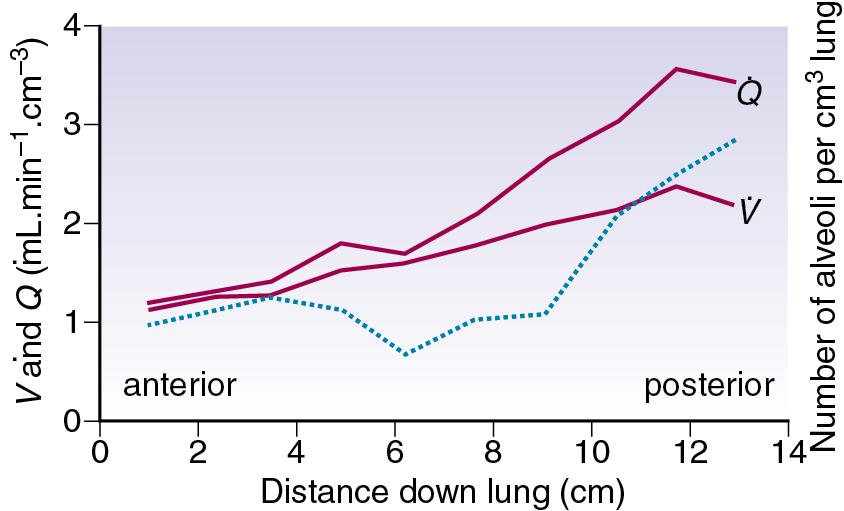
In the supine position the differences in blood flow between apices and bases are replaced by differences between anterior and posterior regions. Supine subjects can be studied using the same variety of scanning techniques as used for assessing ventilation (page 106), revealing the same height-dependent gradients in alveolar size and perfusion as seen in earlier observations in upright subjects. Blood flow per unit lung volume increases by 11% per centimetre of descent through the lung, whereas ventilation increases but less dramatically ( Fig. 7.4 ), resulting in a smaller ventilation to perfusion ![]() ) ratio in dependent areas. These studies also showed that the number of alveoli per cubic centimetre of lung was approximately 30% greater in the posterior compared with anterior lung ( Fig. 7.4 ). Thus the increased perfusion in dependent areas of lung is again mainly caused by an increase in the number of (relatively small) alveoli.
) ratio in dependent areas. These studies also showed that the number of alveoli per cubic centimetre of lung was approximately 30% greater in the posterior compared with anterior lung ( Fig. 7.4 ). Thus the increased perfusion in dependent areas of lung is again mainly caused by an increase in the number of (relatively small) alveoli.
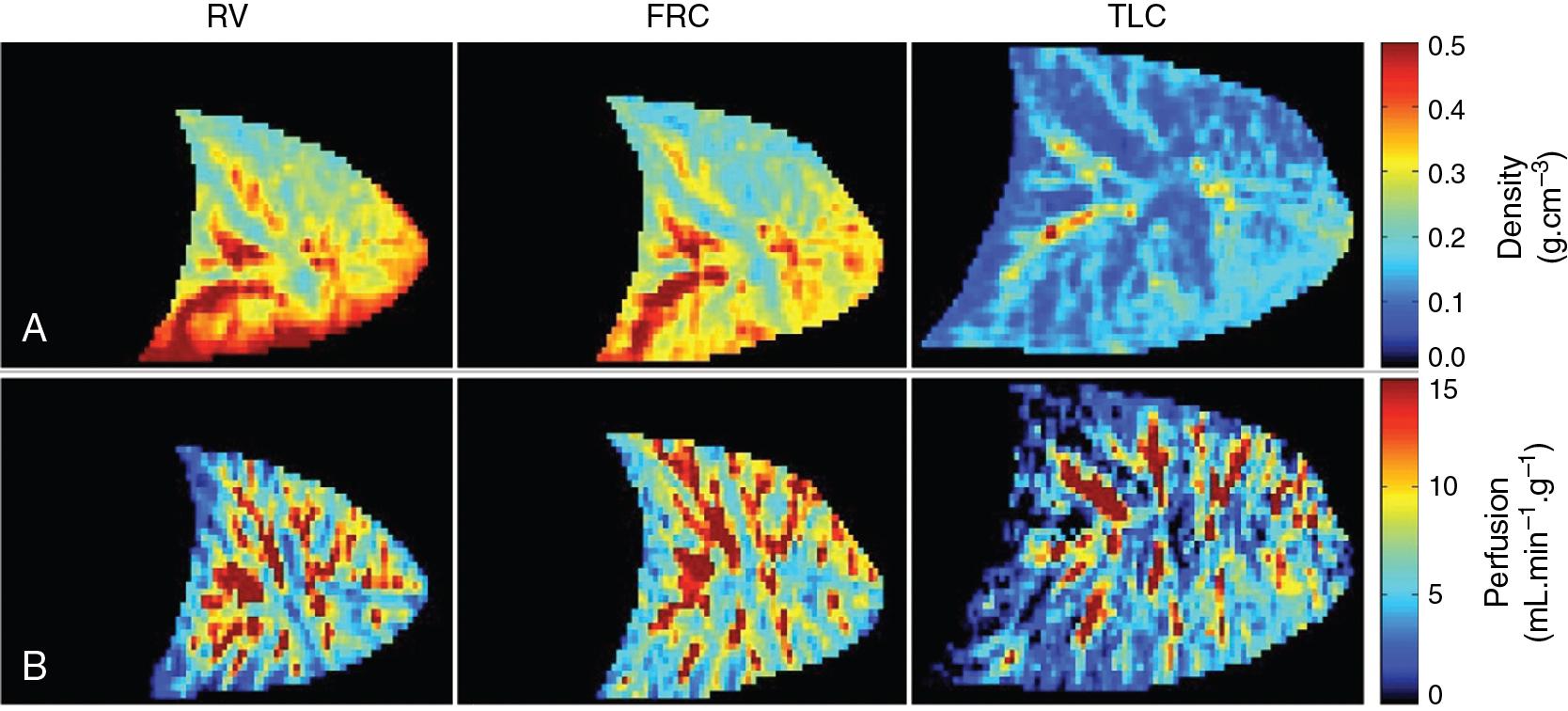
Lung volume also affects regional blood flow distribution. Differences in the density of lung between nondependent and dependent regions are maximal at RV and almost absent at TLC, and when this is taken into account to calculate regional blood flow, the gravitational variation is small and unaffected by lung volume ( Fig. 7.5 ). A similar study using magnetic resonance imaging in healthy subjects demonstrated the variation in lung perfusion with lung height at FRC, FRC+ 500 mL and FRC + 1000 mL to replicate tidal ventilation. Changes in transmural and hydrostatic pressures at different lung volumes appeared to be more important factors in the redistribution of pulmonary perfusion than changes in pulmonary vascular resistance within different lung regions.
It is now accepted that gravity is not the only cause of the variability of regional pulmonary blood flow, although its relative contribution remains controversial. Physiological studies in space some years ago showed that, at microgravity, regional blood flow becomes more uniform than on Earth, but residual nonuniformity still persists (page 229). Also, many studies have measured pulmonary blood flow in the prone position, and found that although blood flow becomes more uniform, the flow distribution when prone is not simply a reversal of the supine position, as may be expected if gravity was the only influence. A tilt table has also been used to assess gravitational effects on lung blood flow at FRC by moving subjects from upright to the extreme position of 80 degrees head down. Gravity-dependent variation in blood flow was clearly demonstrated between the two positions, but lung structure remained a more important determinant of regional blood flow distribution.
Some groups estimate that gravity is responsible for only 25% of the regional blood flow variability seen. Pulmonary blood flow may vary in a radial fashion, with greater flow to central compared with peripheral lung regions in each horizontal slice of lung ( Fig. 7.5 ). Regional flow is believed to be influenced by vascular architecture, with the branching pattern of the pulmonary vasculature responsible for the observed gravity-independent variation (the fractal hypothesis). Two aspects of vascular structure contribute to the variations in flow. First, bifurcations of pulmonary arteries into two slightly different size vessels will have a large effect on the flow rates in each. Second, pulmonary arteries are more numerous than pulmonary airways, as a result of small extra branches, often given off at right angles, throughout the pulmonary arterial tree. Mathematical modelling indicates that these ‘supernumerary’ branches contribute significantly to the heterogeneity of regional perfusion.
It is convenient to consider the relationship between ventilation and perfusion in terms of the ![]() ratio. Each quantity is measured in litres per minute, and, taking the lungs as a whole, typical resting values might be 4 L.min −1 for alveolar ventilation and 5 L.min −1 for pulmonary blood flow. Thus the overall ventilation/perfusion ratio would be 0.8. If ventilation and perfusion of all alveoli were uniform, then each alveolus would have an individual
ratio. Each quantity is measured in litres per minute, and, taking the lungs as a whole, typical resting values might be 4 L.min −1 for alveolar ventilation and 5 L.min −1 for pulmonary blood flow. Thus the overall ventilation/perfusion ratio would be 0.8. If ventilation and perfusion of all alveoli were uniform, then each alveolus would have an individual ![]() ratio of 0.8. In fact, ventilation and perfusion are not uniformly distributed, but may range all the way from unventilated alveoli to unperfused alveoli, with every gradation in between. Unventilated alveoli will have a
ratio of 0.8. In fact, ventilation and perfusion are not uniformly distributed, but may range all the way from unventilated alveoli to unperfused alveoli, with every gradation in between. Unventilated alveoli will have a ![]() ratio of 0, and the unperfused alveoli a
ratio of 0, and the unperfused alveoli a ![]() ratio of infinity.
ratio of infinity.
Alveoli with no ventilation ![]() ratio of 0) will have P o 2 and P co 2 values that are the same as those of mixed venous blood, because the trapped air in the unventilated alveoli will equilibrate with mixed venous blood. Alveoli with no perfusion (
ratio of 0) will have P o 2 and P co 2 values that are the same as those of mixed venous blood, because the trapped air in the unventilated alveoli will equilibrate with mixed venous blood. Alveoli with no perfusion ( ![]() ratio of infinity) will have P o 2 and P co 2 values that are the same as those of the inspired gas, because there is no gas exchange to alter the composition of the inspired gas that is drawn into these alveoli. Alveoli with intermediate
ratio of infinity) will have P o 2 and P co 2 values that are the same as those of the inspired gas, because there is no gas exchange to alter the composition of the inspired gas that is drawn into these alveoli. Alveoli with intermediate ![]() ratio values will thus have P o 2 and P co 2 values that are intermediate between those of mixed venous blood and inspired gas. Figure 7.6 is a P o 2 / P co 2 plot, with the red line joining the mixed venous point to the inspired gas point. This line covers all possible combinations of alveolar P o 2 and P co 2 , with an indication of the
ratio values will thus have P o 2 and P co 2 values that are intermediate between those of mixed venous blood and inspired gas. Figure 7.6 is a P o 2 / P co 2 plot, with the red line joining the mixed venous point to the inspired gas point. This line covers all possible combinations of alveolar P o 2 and P co 2 , with an indication of the ![]() ratio that determines them.
ratio that determines them.
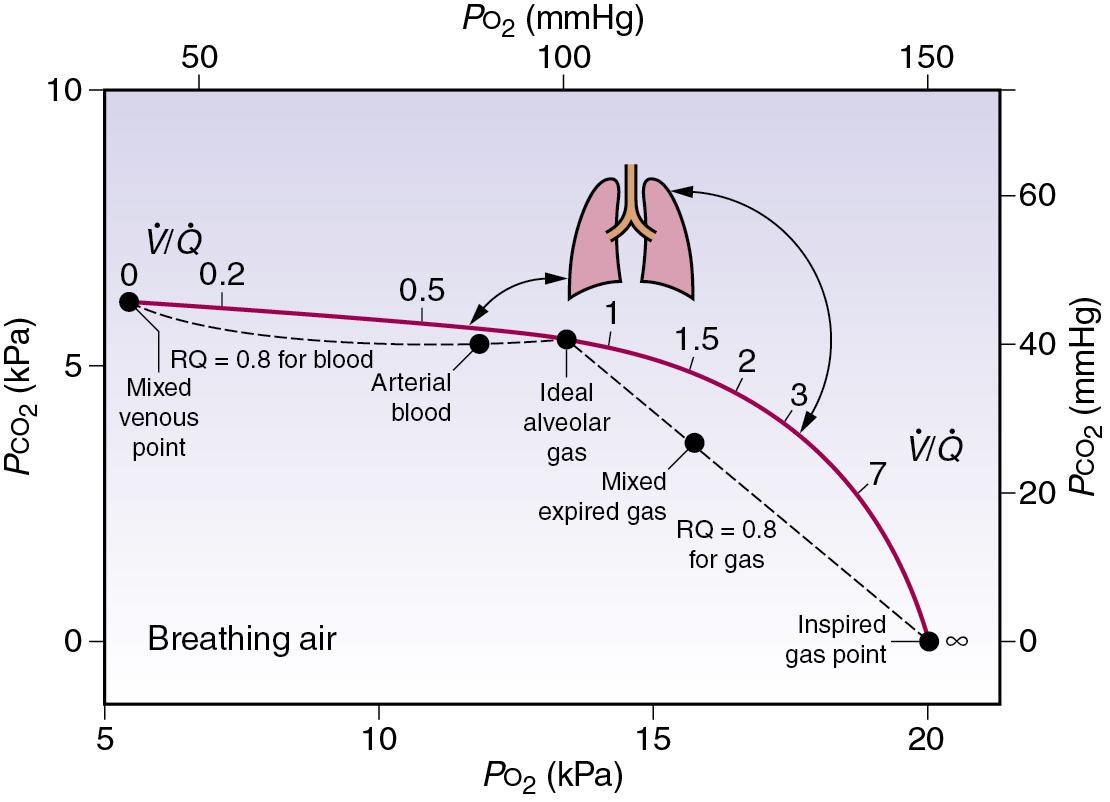
The inhalation of higher than normal partial pressures of oxygen moves the inspired point of the curve to the right. The mixed venous point also moves to the right, but only by a small amount, for reasons that are explained on page 285. A new curve must be prepared for each combination of values for mixed venous blood and inspired gas. The curve can then be used to demonstrate the gas tensions in the horizontal strata of the lung according to their different ![]() ratios ( Fig. 7.6 ).
ratios ( Fig. 7.6 ).
Computational models of ventilation and perfusion based on computed tomography (CT) imaging have shown that the effects of gravity are the most important in ensuring passive ![]() matching in the supine adult human lung. Such gravitational effects include tissue deformation, regional compliance distribution and the effect of the hydrostatic pressure gradient on vessel size. The geometric matching of the arterial tree and the airways has a more minor role in
matching in the supine adult human lung. Such gravitational effects include tissue deformation, regional compliance distribution and the effect of the hydrostatic pressure gradient on vessel size. The geometric matching of the arterial tree and the airways has a more minor role in ![]() matching during supine normal breathing.
matching during supine normal breathing.
As already described, both ventilation and perfusion increase in more dependent regions of the lung, with perfusion increasing slightly more than ventilation. In upright positions in healthy, young subjects breathing at FRC, the ![]() ratio is approximately 2 at the lung apex and 0.8 at the lung bases ( Fig. 7.7 ). In horizontal positions, there is no major difference in
ratio is approximately 2 at the lung apex and 0.8 at the lung bases ( Fig. 7.7 ). In horizontal positions, there is no major difference in ![]() ratio between cranial and caudal ( Fig. 7.7 ) or dependent and nondependent lung regions.
ratio between cranial and caudal ( Fig. 7.7 ) or dependent and nondependent lung regions.

Techniques either employing radioactive tracers or using scanning modalities (page 105) measure regional ventilation and perfusion in three dimensions. The older approaches in particular could only discriminate between functionally fairly large regions of lung tissue. A different approach is the multiple inert gas elimination technique (MIGET), the methodology of which is outlined on page 107, which plots the distribution of pulmonary ventilation and perfusion, not in relation to anatomical location, but in a large number of compartments defined by their ![]() ratios, expressed on a logarithmic scale.
ratios, expressed on a logarithmic scale.
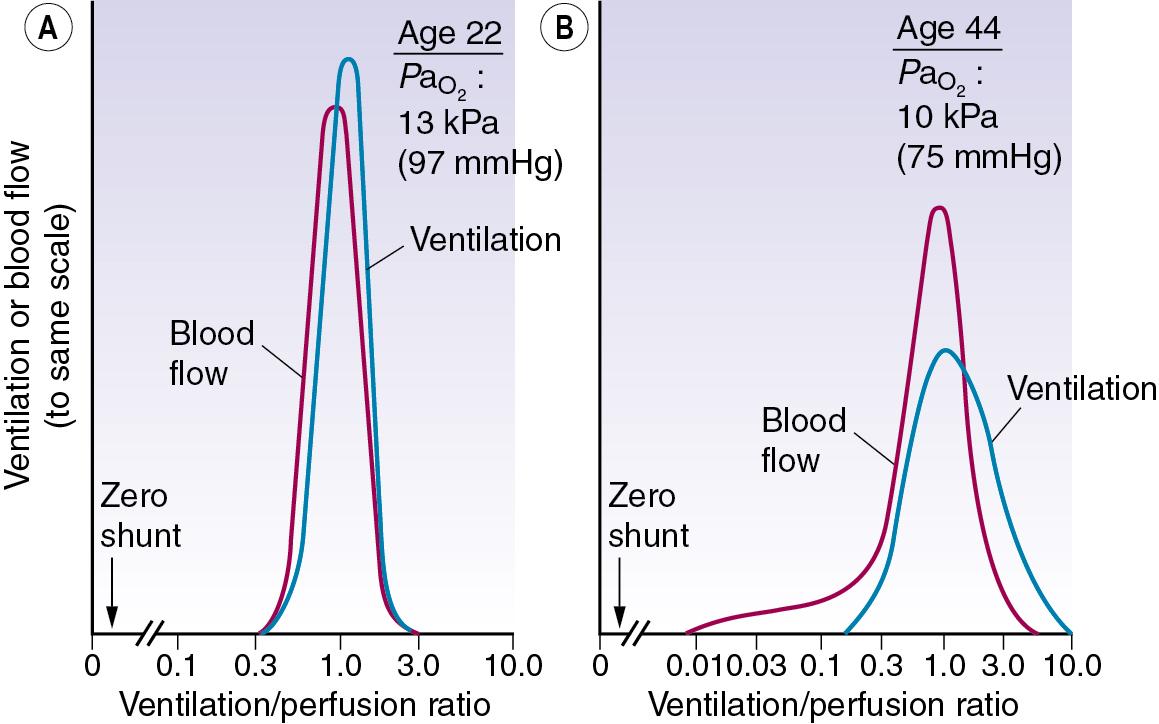
Figure 7.8 shows typical plots for healthy subjects. For the young adult ( Fig. 7.8 , A ), both ventilation and perfusion are mainly confined to alveoli with ![]() ratios in the range 0.5 to 2.0. There is no measurable distribution to areas of infinite
ratios in the range 0.5 to 2.0. There is no measurable distribution to areas of infinite ![]() (i.e., alveolar dead space) or zero
(i.e., alveolar dead space) or zero ![]() ratio (i.e., intrapulmonary shunt), but the method does not detect extrapulmonary shunt, which must be present to a small extent (page 100). For the older subject ( Fig. 7.8 , B ), there is a widening of the distribution of
ratio (i.e., intrapulmonary shunt), but the method does not detect extrapulmonary shunt, which must be present to a small extent (page 100). For the older subject ( Fig. 7.8 , B ), there is a widening of the distribution of ![]() ratios, with the main part of the curve now in the range of
ratios, with the main part of the curve now in the range of ![]() ratios 0.3 to 5.0. In addition, there is the appearance of a ‘shelf’ of distribution of blood flow to areas of low
ratios 0.3 to 5.0. In addition, there is the appearance of a ‘shelf’ of distribution of blood flow to areas of low ![]() ratio in the range 0.01 to 0.3. This probably represents underventilation of dependent areas of the lung because of airway closure when the closing capacity exceeds the functional residual capacity (see Fig. 2.11 ). The effect of increased spread of
ratio in the range 0.01 to 0.3. This probably represents underventilation of dependent areas of the lung because of airway closure when the closing capacity exceeds the functional residual capacity (see Fig. 2.11 ). The effect of increased spread of ![]() ratios on gas exchange is considered in the next section (page 104).
ratios on gas exchange is considered in the next section (page 104).
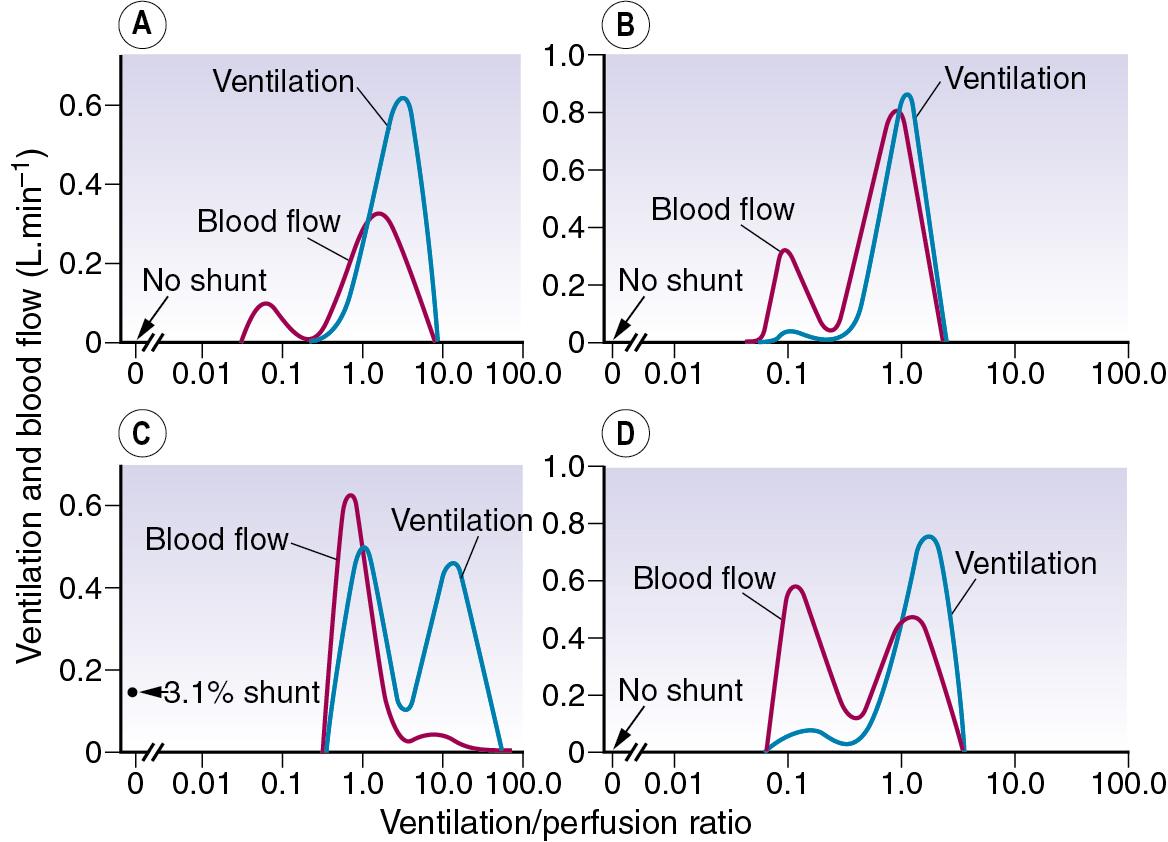
The pattern of distribution of ![]() ratios shows characteristic changes in a number of pathological conditions such as pulmonary oedema and pulmonary embolus. Some examples are shown in Figure 7.9 .
ratios shows characteristic changes in a number of pathological conditions such as pulmonary oedema and pulmonary embolus. Some examples are shown in Figure 7.9 .
The MIGET method of analysis illustrated in Figures 7.8 and 7.9 is technically complex. A less precise but highly practical approach was described in the 1940s by groups including those led by Riley and Cournard. The essence of what has generally become known as the Riley approach is to consider the lung as if it were a three-compartment model ( Fig. 7.10 ) comprising (1) ventilated but unperfused alveoli (alveolar dead space), (2) perfused but unventilated alveoli (intrapulmonary shunt) and (3) ideally perfused and ventilated alveoli.
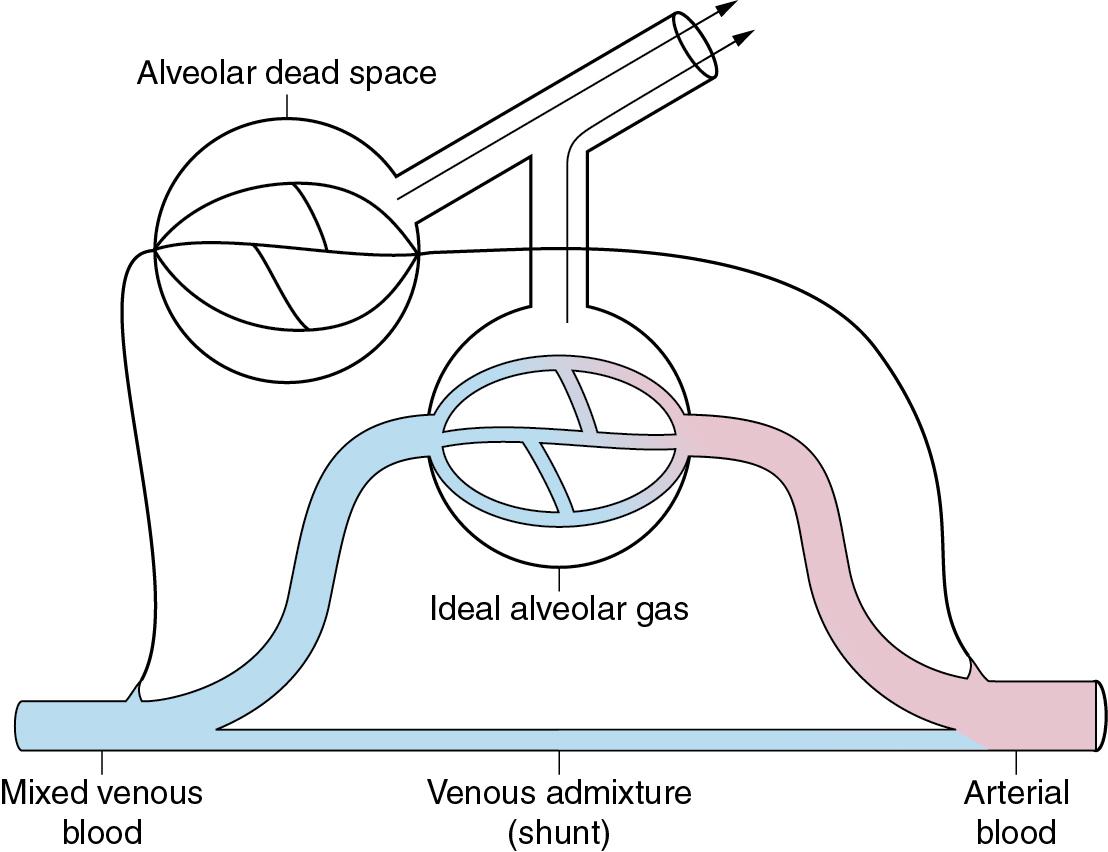
Gas exchange can only occur in the ‘ideal’ alveolus. There is no suggestion that this is an accurate description of the actual state of affairs, which is better depicted by the type of plot shown in Figure 7.8 , where the analysis would comprise about 50 compartments in contrast to the three compartments of the Riley model. However, the parameters of the three-compartment model may be easily determined, and the values obtained are of direct relevance to therapy. Thus an increased dead space can usually be offset by an increased minute volume, and arterial P o 2 can be restored to normal with shunts up to about 30% by an appropriate increase in the inspired oxygen concentration ( Fig. 7.13 ).
Methods for calculating dead space and shunt for the three-compartment model are described at the end of the chapter, but no analytical techniques are required beyond measurement of blood and gas P co 2 and P o 2 . It is then possible to determine what fraction of the inspired tidal volume does not participate in gas exchange and what fraction of the cardiac output constitutes a shunt. However, it is most important to remember that the measured value for ‘dead space’ will include a fraction representing ventilation of relatively underperfused alveoli ( ![]() >1 and
>1 and ![]() <∞), and the measured value for ‘shunt’ will include a fraction representing perfusion of relatively underventilated alveoli (
<∞), and the measured value for ‘shunt’ will include a fraction representing perfusion of relatively underventilated alveoli ( ![]() >0 and
>0 and ![]() <1). Furthermore, although perfusion of relatively underventilated alveoli will reduce arterial P o 2 , the pattern of change, in relation to the inspired oxygen concentration, is quite different from that of a true shunt ( Fig. 7.14 ).
<1). Furthermore, although perfusion of relatively underventilated alveoli will reduce arterial P o 2 , the pattern of change, in relation to the inspired oxygen concentration, is quite different from that of a true shunt ( Fig. 7.14 ).
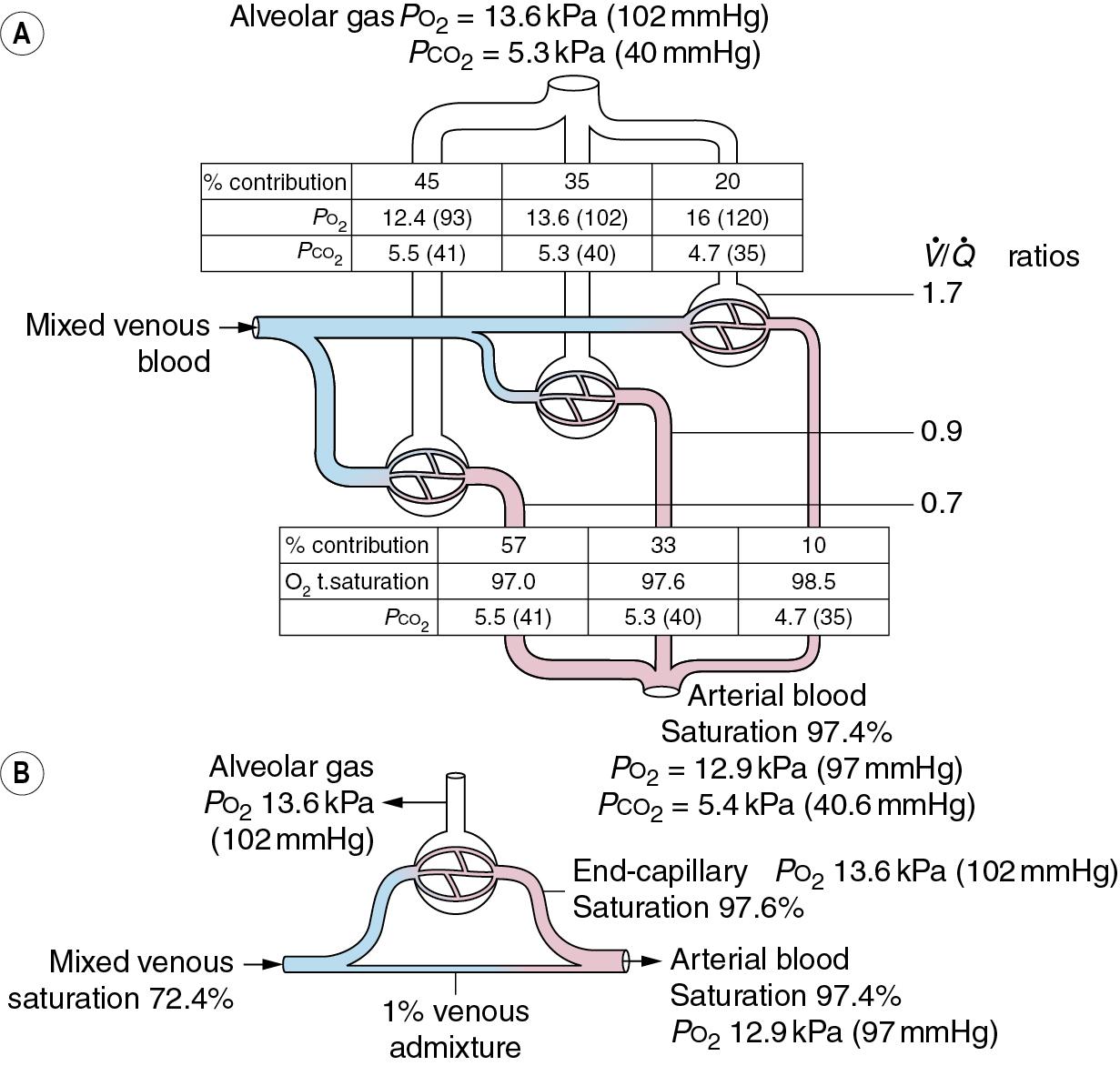
The concept of ideal alveolar gas is considered on page 107, but it will be clear from Figure 7.10 that ideal alveolar gas cannot be sampled for analysis. There is a convention that ideal alveolar P co 2 is assumed to be equal to the arterial P co 2 , and that the respiratory exchange ratio of ideal alveolar gas is the same as that of expired air.
Become a Clinical Tree membership for Full access and enjoy Unlimited articles
If you are a member. Log in here